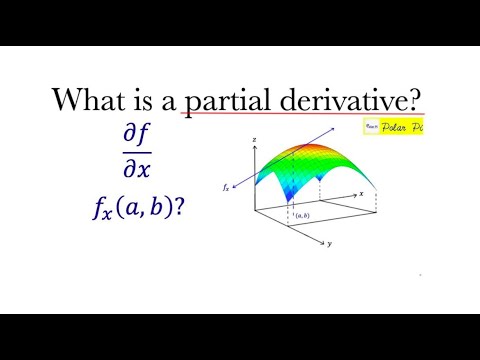
Formal definition of partial derivatives

Partial derivatives are formally defined using a limit, much like ordinary derivatives.

About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content.

For free. For everyone. Forever. #YouCanLearnAnything

[gurmita]

What i love the most about Khan Academy is that every concept is explained to its core.

[IvanGoncharovAI]

It's super cool to listen to 3b1b in this sort of live format))

[jeanphilippepuyravaud]

Difficult to remember to put "like" to all videos. Amazing teaching.

[papername]

That h notation is really just there to cause confusion. Thank you for pointing out what it really just it.

[_huzefajohar]

Thank you so much for these incredibly valuable explainations that help us not only understand but also get a real feel of the difficult and complex concepts of calculus. This level of understanding of the concepts even most maths professors dont have, and what they teach is nowhere near to what we can learn from here.

[futterkulcha]

This is my favourite video in the series. Somewhere along the way I thought I was catching glimpses of the secrets of the universe...

[Adam]

I love your videos. With your videos I do believe anything can be learned.

[curtpiazza]

Thank you for clarifying ...great explanation! 🎉

[JrDarkPhantom]

When he wrote the limits, he didn't extend the fraction signs for the entire numerator... It should include all of [F(a+h, b) - F(a, b)]. I hope no one was confused by that.

[cliffordwilliams]

Whenever I hear that voice, I know I'm going to learn some _real math!
WE LOVE YOU GRANT SANDERSON!!!

[JoaoJGabriel]

at 0:55 f(x) gets prettier as the value of x increases

[wast]

Really interseting approach of interpreting the derivative 5:13

[BigNWide]

Why is h used for a nudge in the x direction? I suspect it is related to the use of (h, k) to represent the image of the origin for a geometric transformation. The variable h is used there as well for a shift in the x direction.

[tdavis]

you could start this from about 7 minutes and go from there, refer to prev lessons will save time. also, could mention that the new thing is each value (x, y, z, i, , j, k...) just becomes a function, after that its all familiar but now for n dimensional geometries

[ndianzon]

(a, f(a)) and (b, f(b)) are points on the graph x^2 and h = b - a, solve for b, b = h+a, then (a, f(a)) and (h+a, f(a+h)). slope = f(a+h) - f(a)/h

[ookere]

how can i find out which playlist is this video from ?

[kaustubhpandey]

"room for rigour"
Funny phrase right

[michaelkeller]

I think Khan Academy deserves the 400 bucks I paid my school for Calculus 1.

[mstevens]

Shouldn't the partial derivative be "del" not "d"?

[thomasdiprima]

Sorry, but Grant takes 10 minutes to explain something that Sal can do in five minutes. When you go into that much detail it tends to get muddled.