Quantum Tomography and Schwinger's Approach to Quantum Mechanics by Guiseppe Marmo

Title: Quantum Tomography and Schwinger's Approach to Quantum Mechanics
Presenter: Guiseppe Marmo, Università di Napoli Federico II, Napoli, Italy
Conference: Koopman Methods in Classical and Classical-Quantum Mechanics
Sponsored by: Wilhelm und Else Heraeus-Stiftung and Tulane University

The description of any physical system, be it classic or quantum, requires the identification of i) States; ii)Observables ; iii) Probability map, a pairing between states and observables with values in the space of probability measures on the real line ; iv) Evolution ; v) Composition rule ,to construct systems out of elementary ones.
Heisenberg , Born ,and Jordan started from observables as primary objects .They constructed Matrix Mechanics (later developed into the C*-algebraic approach ). Schrödinger ,Dirac ,and von Neumann started from vector states (the Hilbert-space approach) as primary objects.
Quantum Tomography deals with statistical-probabilistic aspects as primary
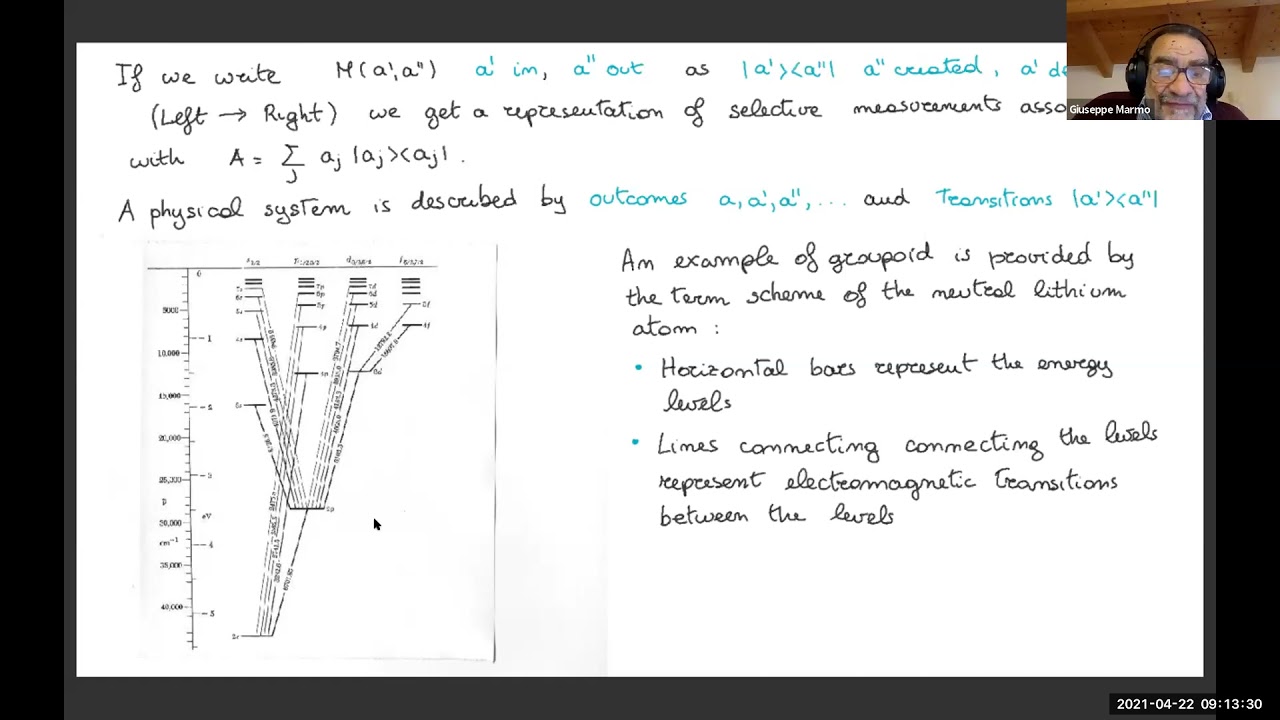
objects. It may be considered as “Quantum Mechanics on Phase Space” ,and may be ascribed, mainly, to Wigner and Weyl. Tomography describes quantum states by means of fair probability distributions ( marginal distributions) associated with quasidistributions on phase-space introduced by Wigner .In this respect it allows to deal with “quantum” and “classical” systems by means of the same ingredients. On the other hand ,Schwinger’s approach (The Algebra of Atomic Measurements) has been formalized recently by means of groupoids. This approach ,stemming from Stern-Gerlach experiment, was presented as “purely quantum”. But as Einstein used to say: “ I do not believe in micro and macro laws, but only in (structural) laws of general validity," we will argue that the groupoid approach allows to deal with quantum and classical systems in the same structural setting.