filmov
tv
What if gravity were proportional to sin(r)/r² instead of 1/r²? | Not-Quite-Physics Simulations
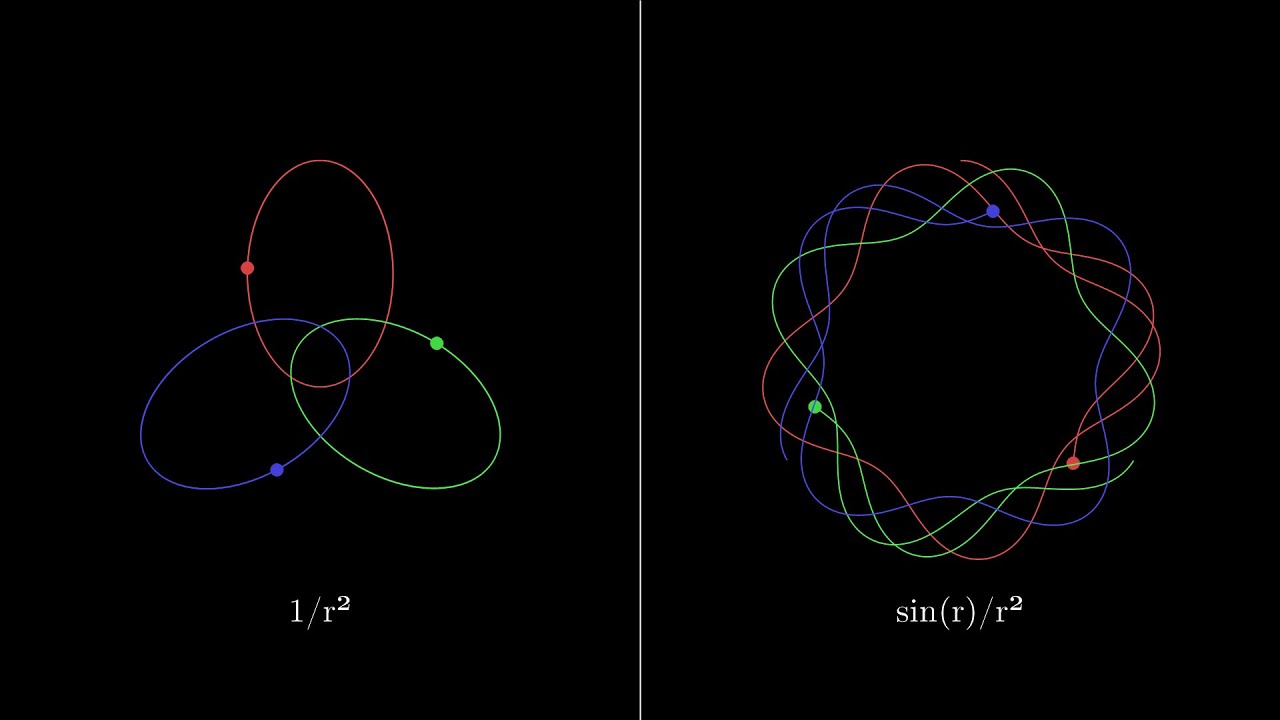
Показать описание
Two simulations of the three-body problem. The forces between the masses in the simulation on the left are proportional to 1/r². The forces between the masses in the simulation on the right are proportional to sin(r)/r². In both simulations, all three masses have the same starting positions and velocities.
The simulation on the right is basically nonsense - it's just for fun.
- all masses equal
- symmetric start
- 25fps, 60s
#Physics #PhysicsSimulations #ThreeBodyProblem #Gravity
The simulation on the right is basically nonsense - it's just for fun.
- all masses equal
- symmetric start
- 25fps, 60s
#Physics #PhysicsSimulations #ThreeBodyProblem #Gravity
What if gravity were proportional to 1/r³ instead of 1/r²? | N-Body Problem | Physics Simulations
What if gravity were proportional to 1/r instead of 1/r²? | N-Body Problem | Physics Simulations
What if gravity were proportional to 1/r³ instead of 1/r²? | Physics Simulations
What if gravity were proportional to 1/r instead of 1/r²? | Three-Body Problem | Physics Simulations...
What if gravity were proportional to e^-r? | Physics Simulations
What if gravity were proportional to e^-r? | Physics Simulations
What if gravity were proportional to sech(r)? | Three-Body Problem | Physics Simulations
What if gravity were proportional to sech²(r)? | Three-Body Problem | Physics Simulations
A Gravitational Forces
What if gravity were proportional to 1/arctan²(r)? | Three-Body Problem | Physics Simulations
What if gravity were proportional to 1/r^(1.5) instead of 1/r²? | Physics Simulations
What if gravity were proportional to sech²(r)? | Three-Body Problem | Physics Simulations
What if gravity were proportional to sech²(r)? | N-Body Problem | Physics Simulations
What if gravity were proportional to e^-r? | N-Body Problem | Physics Simulations
What if gravity were proportional to sech²(r)? | N-Body Problem | Physics Simulations
What if gravity were proportional to sech(r)? | N-Body Problem | Physics Simulations
What if gravity were proportional to sin(r) instead of 1/r²? | Not-Quite-Physics Simulations
What if gravity were proportional to sin(r)/r² instead of 1/r²? | Not-Quite-Physics Simulations
What if gravity were proportional to sin(r) instead of 1/r²? | Not-Quite-Physics Simulations
What if gravity were proportional to e^-r? | N-Body Problem | Physics Simulations
What if gravity were proportional to 1/r^(1.5) instead of 1/r²? | Physics Simulations
What if gravity were proportional to sech(r)? | N-Body Problem | Physics Simulations
What if gravity were proportional to 1/arctan²(r)? | N-Body Problem | Physics Simulations
What if gravity were proportional to sech(r)? | Three-Body Problem | Physics Simulations
Комментарии
 0:01:30
0:01:30
 0:01:30
0:01:30
 0:01:30
0:01:30
 0:01:30
0:01:30
 0:00:57
0:00:57
 0:01:00
0:01:00
 0:01:00
0:01:00
 0:01:00
0:01:00
 1:05:02
1:05:02
 0:01:01
0:01:01
 0:01:30
0:01:30
 0:00:57
0:00:57
 0:01:00
0:01:00
 0:00:57
0:00:57
 0:00:57
0:00:57
 0:00:57
0:00:57
 0:01:01
0:01:01
 0:01:00
0:01:00
 0:01:31
0:01:31
 0:01:00
0:01:00
 0:02:30
0:02:30
 0:01:00
0:01:00
 0:01:00
0:01:00
 0:00:57
0:00:57