filmov
tv
Moment Generating Function #2 (Continuous Distribution)
Показать описание
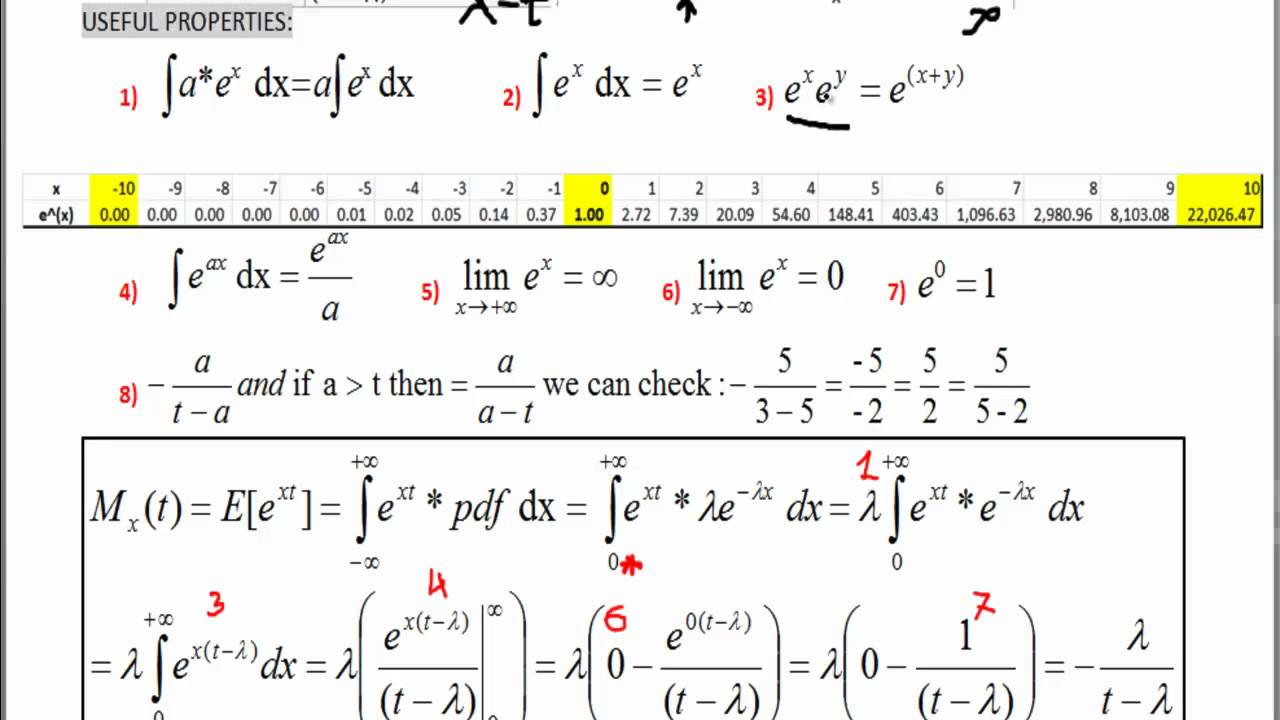
In this video I define the concept of Moment Generating Function and show how to derive it (using exponential density as an example). Exponential density function is not easy to work with however this will pay off as we review a lot of integration/exponential properties.
Moment Generating Function #2 (Continuous Distribution)
What is a Moment Generating Function (MGF)? ('Best explanation on YouTube')
Moment generating functions -- Example 2
Moment generating functions -- Example 1
MA 381: Section 11.1: Moment Generating Functions, Part 4
Properties of Moment Generating Functions: An Example.
Moment generating function MGF for continuous case
MA 381: Section 11.1: Moment Generating Functions, Part 3
Using moment generating functions to generate moments
Moment Generating Functions (II)
Bivariate distributions; moment generating functions
Moment Generating Functions (Part 1)
Introduction to moment generating functions
Moment Generating Function - Part 1
Normal distribution moment generating function
Moment Generating Function for Continuous Random Variable
'Moment Generating Functions of Continuous Distributions' | Probability with Educator.com
Moment generating function technique -- Example 2
24.5: Probability & Statistics - Moment generating function
Lecture 14 (Part 8): M.G.F of i.i.ds; Joint Moment Generating functions with examples
PTSP#Problem on MGF
Using Moment Generating Functions to find the Variance: Example 1
Moment Generating Function - Part 2
Probability: moment generating functions
Комментарии
 0:08:35
0:08:35
 0:08:51
0:08:51
 0:04:38
0:04:38
 0:05:19
0:05:19
 0:08:54
0:08:54
 0:04:39
0:04:39
 0:11:50
0:11:50
 0:06:43
0:06:43
 0:02:51
0:02:51
 0:18:22
0:18:22
 0:02:16
0:02:16
 0:08:25
0:08:25
 0:09:05
0:09:05
 0:19:32
0:19:32
 0:02:43
0:02:43
 0:17:27
0:17:27
 0:07:10
0:07:10
 0:03:09
0:03:09
 0:05:34
0:05:34
 0:22:05
0:22:05
 0:02:58
0:02:58
 0:04:52
0:04:52
 0:07:23
0:07:23
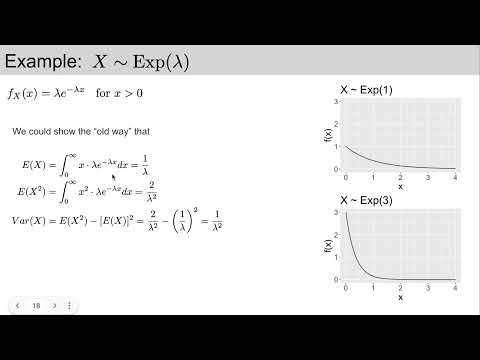 0:14:58
0:14:58