Integration of Hyperbolic Functions (6) | ExamSolutions

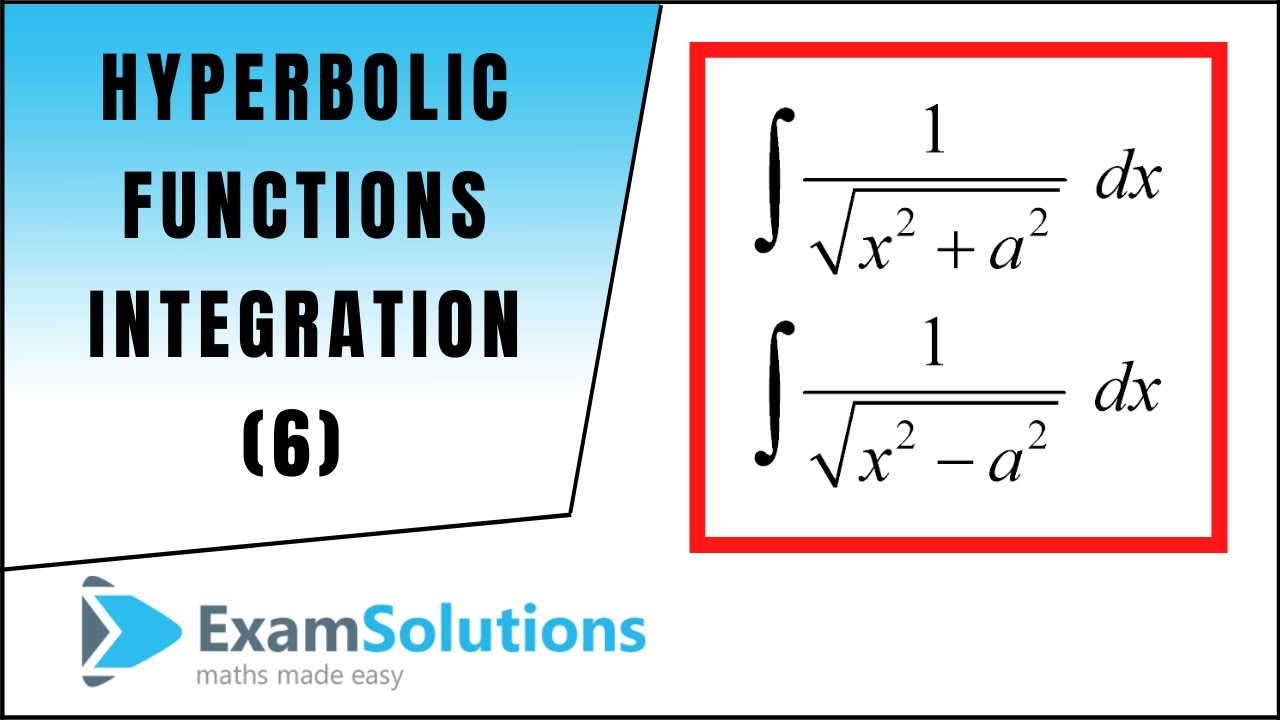
This is the 6th video in this series on integrating hyperbolic functions where I look at integrals that resolve to inverse hyperbolic functions, sinh^-1(x/a) and cosh^-1(x/a) or arsinh(x/a) and arcosh(x/a).

RELATED TUTORIALS
Integration of hyperbolic functions playlist:

hyperbolic functions: (Playlist includes identities)

differentiation of hyperbolic functions:

[Shaan_Suri]

Why not use the substitution x = a*tanu for the first problem? Doesn't that identity also simplify the integrand, yielding the result ln|sqrt(a^2+x^2 + x)/a| + C ?

[farhatfatima]

How can we understand that we suppose x=coshu or x= sinhu???

[tuneese]

where did you get the substitution x=asinhu