filmov
tv
A Quick and Easy Radical System
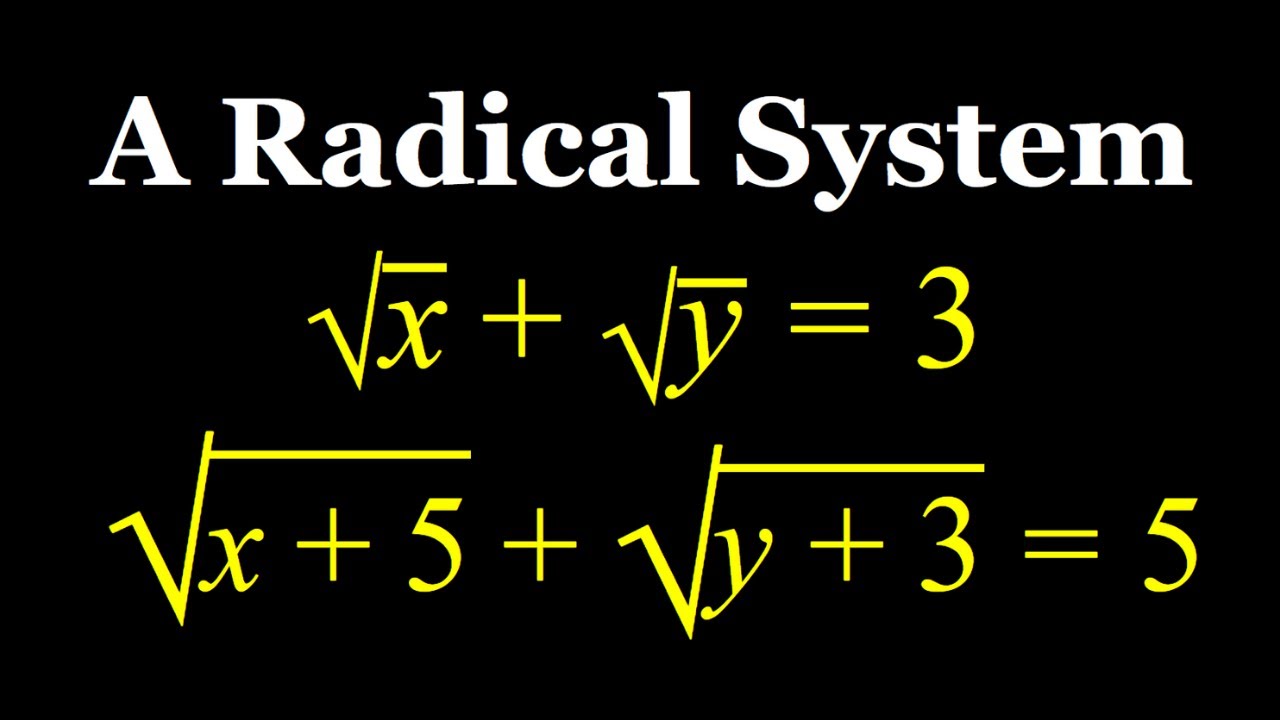
Показать описание
This video is about solving a Radical System
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #RadicalSystems
EXPLORE:
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #RadicalSystems
EXPLORE:
A Quick and Easy Radical Equation
Simplifying a Quick and Easy Radical Expression
A Quick and Easy Radical System
A Quick and Easy Radical Equation
Let's Solve A Quick and Easy Radical Equation #maths #mathematics #algebra
A Quick and Easy Exponential/Radical Equation
Pre-Algebra 31 - Simplifying Radical Expressions
Radical Math Made EASY with These Simple Tricks!
'The Unbearable Lightness of Being' - Milan Kundera
Radical Simplicity
Solving Radical Equations
How To Simplify a Radical
Learn how to simplify a radical
Pokemon Radical Red: VS. Move Maniac | Easy Puzzle Solve
Simplify a radical expression with variables
Radical And Radicands With Complete Rules | How to Simplify Radicals and Radicands | Sir Shuja.|9th.
Learn to add and subtract radical expressions
Germany l can you solve this radical problem?? l Easy Solution l find value of x?
Can you solve this Radical Expression in 30 Seconds? | Fast & Easy Trick
How to Solve Radical Equations that have Two Radicals - Simple Method
Simplifying a radical expression using rational exponents
Simplifying the cube root of a radical expression
Solving radical equations | Exponent expressions and equations | Algebra I | Khan Academy
A Very Nice Math Olympiad Problem | Solve for x? | Algebra | Radical equation
Комментарии
 0:09:16
0:09:16
 0:05:01
0:05:01
 0:08:48
0:08:48
 0:06:03
0:06:03
 0:00:58
0:00:58
 0:03:50
0:03:50
 0:09:28
0:09:28
 0:12:23
0:12:23
 9:35:46
9:35:46
 0:45:53
0:45:53
 0:17:11
0:17:11
 0:03:27
0:03:27
 0:04:09
0:04:09
 0:03:19
0:03:19
 0:05:14
0:05:14
 0:26:09
0:26:09
 0:03:41
0:03:41
 0:09:12
0:09:12
 0:02:03
0:02:03
 0:08:23
0:08:23
 0:04:26
0:04:26
 0:02:13
0:02:13
 0:03:11
0:03:11
 0:10:38
0:10:38