filmov
tv
Krzysztof Krupinski: Amenable theories

Показать описание
The lecture was held within the framework of the Hausdorff Trimester Program: Logic and Algorithms in Group Theory.
Abstract:
I will introduce the notion of an amenable theory as a natural counterpart of the notion of a definably amenable group. Roughly speaking, amenability means that there are invariant (under the action of the group of automporphism of a sufficiently saturated model), Borel, probability measures on various types spaces. I will discuss several equivalent definitions and give some examples. Then I will discuss the result that each amenable theory is Gcompact. This is a part of my recent paper (still in preparation) with Udi Hrushovski and Anand Pillay.
Abstract:
I will introduce the notion of an amenable theory as a natural counterpart of the notion of a definably amenable group. Roughly speaking, amenability means that there are invariant (under the action of the group of automporphism of a sufficiently saturated model), Borel, probability measures on various types spaces. I will discuss several equivalent definitions and give some examples. Then I will discuss the result that each amenable theory is Gcompact. This is a part of my recent paper (still in preparation) with Udi Hrushovski and Anand Pillay.
 1:00:44
1:00:44
 0:51:19
0:51:19
 1:43:59
1:43:59
 0:53:53
0:53:53
 0:56:04
0:56:04
 0:56:44
0:56:44
 0:48:10
0:48:10
 0:55:46
0:55:46
 0:53:28
0:53:28
 0:50:54
0:50:54
 0:53:26
0:53:26
 0:54:39
0:54:39
 1:00:52
1:00:52
 0:14:10
0:14:10
 0:25:11
0:25:11
 0:00:41
0:00:41
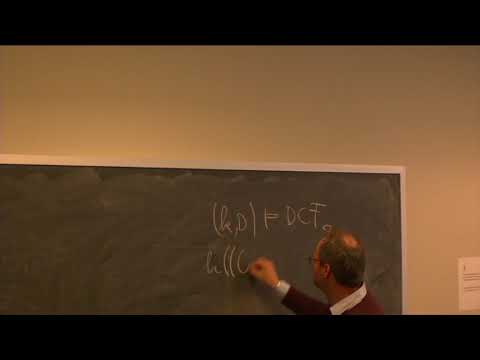 1:14:27
1:14:27
 1:25:21
1:25:21
 0:32:42
0:32:42
![Vílne (Vilnius) [LT]:](https://i.ytimg.com/vi/u0Wako3md4c/hqdefault.jpg) 0:02:41
0:02:41