filmov
tv
Indefinite integral of 1/x | AP Calculus AB | Khan Academy

Показать описание
In differential _calculus we learned that the derivative of ln(x) is 1/x. Integration goes the other way: the integral (or antiderivative) of 1/x should be a function whose derivative is 1/x. As we just saw, this is ln(x). However, if x is negative then ln(x) is undefined! The solution is quite simple: the antiderivative of 1/x is ln(|x|). Created by Sal Khan.
AP Calculus AB on Khan Academy: Bill Scott uses Khan Academy to teach AP Calculus at Phillips Academy in Andover, Massachusetts, and heÕs part of the teaching team that helped develop Khan AcademyÕs AP lessons. Phillips Academy was one of the first schools to teach AP nearly 60 years ago.
For free. For everyone. Forever. #YouCanLearnAnything
Indefinite integral of 1/x | AP Calculus AB | Khan Academy
Indefinite Integral of 1/x^2
The Integral of 1/x EXPLAINED. It's NOT what you think...
∫1/x dx | Evaluate the Indefinite Integral | Worked out Solution
Integral of 1/x
∫-(1/x) dx | Evaluate the Indefinite Integral | Worked out Solution
Indefinite Integral ( ∫ 1 / (x+1) dx )
Antiderivative of x^n and 1/x - Basic Integration Rules for Indefinite Integrals | Glass of Numbers
Indefinite Integral
Integral of 1/x^4 ( Complex valued)
Integrating a Definite Integral vs. An Indefinite Integral Example #Shorts #calculus #math
Indefinite Integral
Indefinite integral of 1/x
Math Integration Timelapse | Real-life Application of Calculus #math #maths #justicethetutor
[CalcYou] Solving Indefinite Integrals Example 1
Curious case of Integration of 1/x (Indefinite Integral of 1/x) - Teachoo
Calculus SPEEDRUN (U-Sub)!!
Use substitution to evaluate to indefinite integral dx/(1-x)^2
Differentiation and integration important formulas||integration formula
How REAL Men Integrate Functions
Why Do We Use a Constant After Integrating an Indefinite Integral? #Shorts #calculus #math #learn
INDEFINITE INTEGRAL PART 6 #math #intergral #shorts
Integral of 1/(1+x^2)
INTEGRATION IMPORTANT QUESTION | CBSE BOARDS | CLASS 12 MATHS | STATE BOARDS | CUET #shorts_
Комментарии
 0:07:35
0:07:35
 0:10:09
0:10:09
 0:03:12
0:03:12
 0:01:12
0:01:12
 0:00:50
0:00:50
 0:01:24
0:01:24
 0:02:00
0:02:00
 0:22:56
0:22:56
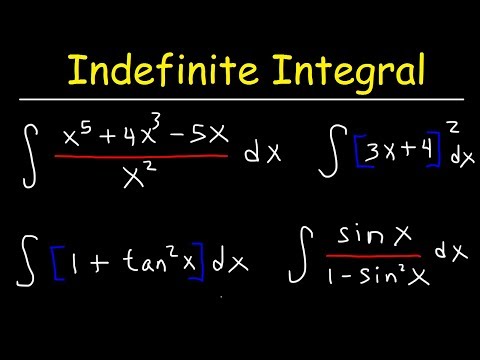 0:10:47
0:10:47
 0:12:13
0:12:13
 0:00:24
0:00:24
 0:05:15
0:05:15
 0:13:44
0:13:44
 0:00:09
0:00:09
![[CalcYou] Solving Indefinite](https://i.ytimg.com/vi/UIHzbXY85ps/hqdefault.jpg) 0:01:32
0:01:32
 0:05:05
0:05:05
 0:01:00
0:01:00
 0:02:20
0:02:20
 0:00:16
0:00:16
 0:00:35
0:00:35
 0:00:38
0:00:38
 0:00:11
0:00:11
 0:02:29
0:02:29
 0:00:33
0:00:33