How to rotate in higher dimensions? Complex dimensions? | Lie groups, algebras, brackets #2

Around 11:50, can't imagine that this error got in - it should have been SU(n) = {U in U(n), det U = 1}.

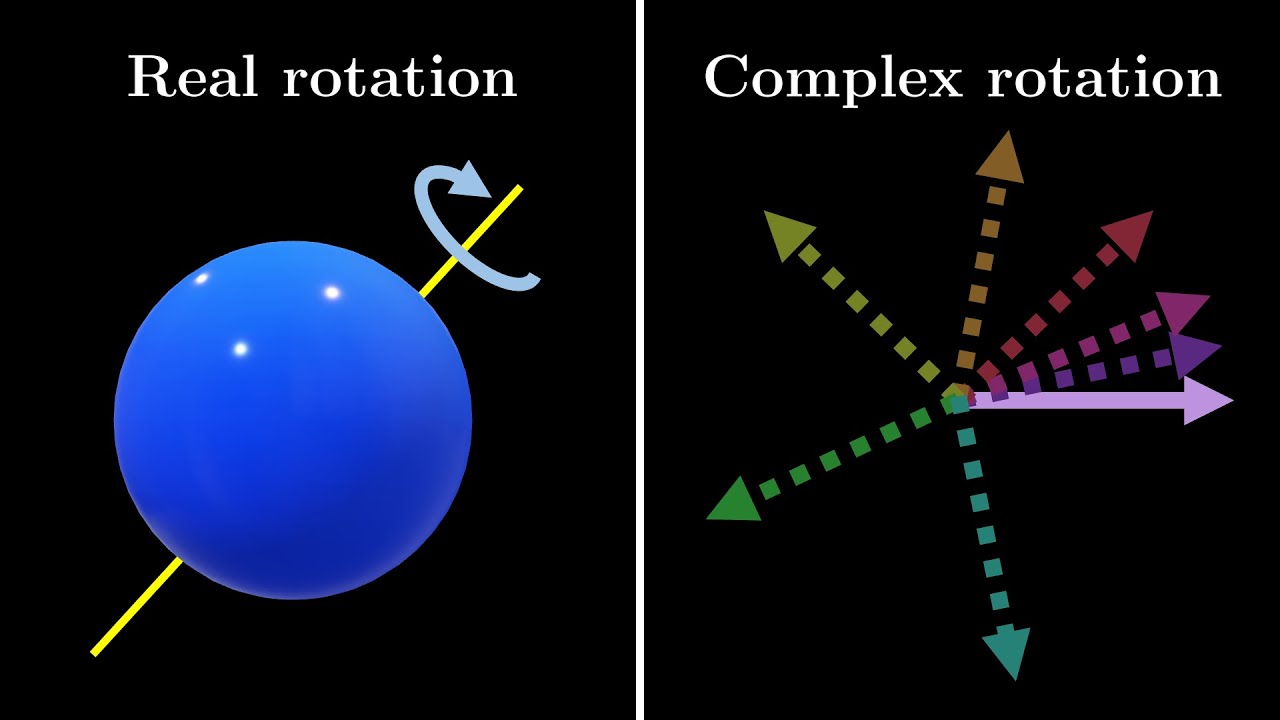
Orthogonal and unitary groups. Rotational symmetries, real and complex, are particularly useful in the field of Lie theory, because their (complexified) Lie algebras, together with that of the symplectic group Sp(n), are the only infinite families of simple Lie algebras. This video is to familiarise with the SO(n), SU(n) notations, and provides further motivation to study Lie theory.

Files for download:

Video chapters:
00:00 Introduction
01:04 Real rotation in n dimensions
07:03 Complex rotation

Other than commenting on the video, you are very welcome to fill in a Google form linked below, which helps me make better videos by catering for your math levels:

If you want to know more interesting Mathematics, stay tuned for the next video!

SUBSCRIBE and see you in the next video!

If you are wondering how I made all these videos, even though it is stylistically similar to 3Blue1Brown, I don't use his animation engine Manim, but I use PowerPoint, GeoGebra, and (sometimes) Mathematica to produce the videos.

Social media:

For my contact email, check my About page on a PC.

See you next time!

[mathemaniac]

Originally I wanted to include at least the definition of a group in this prerequisite video, but really couldn’t fit it in properly, so I will postpone it to the next video (together with a rough definition of a manifold). This is an extremely standard video, but I will promise a lot more visuals in the upcoming ones - as said, this is just a prerequisite, and mainly for me to use the notation SO(n), SU(n) without explaining again.

[hoggif]

This was the clearest explanation for O(n)/U(n)/SO(n)SU(n) I've seen so far. Well done! Looking forward to the rest of this series!

[colinbarker]

This is the most natural introduction to the rotational and unitary groups I've ever seen. It makes me feel like I've missed out on the geometry of these groups for years! Thanks for your hard work.

[lordeji]

THIS is what teacher should do.
Never drop an definition without explaining WHY. Amazing job, i can't wait !!

[JakubS]

Interesting, so now I know what an SU(n) group is! I've heard that it's used to describe the behaviour of forces in Quantum Mechanics.

[tempiadem]

These videos make higher level maths so much more approachable. Looking forward to the rest of the series!

[frozencryo]

Cutting all the fluff and educating in a direct, simplistic and elegant manner, exactly what maths education videos should be. Look forward to the rest of the series and recapping some good old QFT.

[sophiophile]

I was the person who asked if you could cover the topic in the community post asking fod suggestions.

Thank you so much. Your style is fantastic, and I can't wait to continue watching.

[paunb]

You were a lifesaver! I was reading Visual Complex Analysis by T. Needham and I was stuck at this part; and you just popped up in my recommendations and answered all my questions

[dindian]

Amazing. As a student of engineering, the walls to learn pure math are very high. Thank you for providing a passage through!

[howhuiliew]

Thanks for your amazingly good introduction of orthogonal group.

[dominikbaron]

Great video! I recently fell into the quantum mechanics rabbit hole on Wikipedia and "lie group" was one of the first terms where I had no idea what it meant. I was really happy when you announced your lie group series not long after - great timing ^^. Now I am really looking forward to the rest of the series, to get one step closer to understanding the math behind QM

[polymergel]

What a fascinating video about Lie group this is! I have waited for this kind of videos for decades.

[johnchessant]

Ooh this is a really good motivation! I'm on the edge of my seat for the next video!

[ominollo]

Thanks! I finally learnt what SO and SU stand for 🙂

[KarlyVelez-uk]

GREAT video! Looking forward to seeing the rest :). Superb clarity! First time I see this all exposed so clearly..

[wafikiri_]

Superb clarity! First time I see this all exposed so clearly.

[alejrandom]

I knew that R^T R = I for orthogonal matrices, and knew the algebraic proof but didn't realize that just by noticing that angles and dot product are preserved, you can get there quicker. So intuitive. Thanks 😊

[fedebonons]

Nice video, waiting for the next one!

[MahdiSahranavard-hgev]

Your voice is the best
Clearly calm and nice
Thanks for understand who love mathematics phisic and more science but not English first language or native English