filmov
tv
3d curl intuition, part 1
Показать описание
Here we start transitioning from the understanding of two-dimensional curl into an understanding of three-dimensional curl.
3d curl intuition, part 1
3d curl intuition, part 1
3d curl formula, part 1
3d curl intuition, part 2
Divergence intuition, part 1
The CURL of a 3D vector field // Vector Calculus
Divergence and curl: The language of Maxwell's equations, fluid flow, and more
2d curl intuition
3d curl computation example
Curl
Gradient→Curl→Divergence. Intuition with John Harland
3d curl formula, part 1
Resulting curl vector field
GRADIENT, DIVERGENCE & CURL || Part 1: Intuitive Understanding of Fields|| Calculus || Taha Saqu...
Vector Calculus: Understanding Curl
Laplacian intuition
2d vector field with curl vectors shown
What Does the Gradient Vector Mean Intuitively?
Curl math
Calculus 3 Lesson The Divergence, Curl and Del in 2D and 3D
Curl of a vector
Divergence and Curl
Intuition on the Divergence Theorem (Part 1)
Stokes' Theorem // Geometric Intuition & Statement // Vector Calculus
Комментарии
 0:05:50
0:05:50
 0:05:50
0:05:50
 0:07:40
0:07:40
 0:08:17
0:08:17
 0:06:21
0:06:21
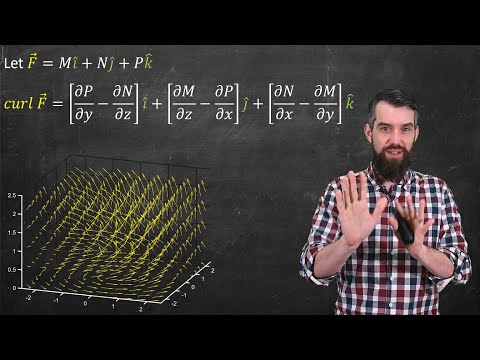 0:08:26
0:08:26
 0:15:42
0:15:42
 0:03:54
0:03:54
 0:05:35
0:05:35
 0:06:26
0:06:26
 1:25:56
1:25:56
 0:07:40
0:07:40
 0:00:19
0:00:19
 0:27:43
0:27:43
 0:10:33
0:10:33
 0:05:31
0:05:31
 0:00:24
0:00:24
 0:02:14
0:02:14
 0:00:24
0:00:24
 0:25:22
0:25:22
 0:01:29
0:01:29
 0:25:33
0:25:33
 0:27:11
0:27:11
 0:08:32
0:08:32