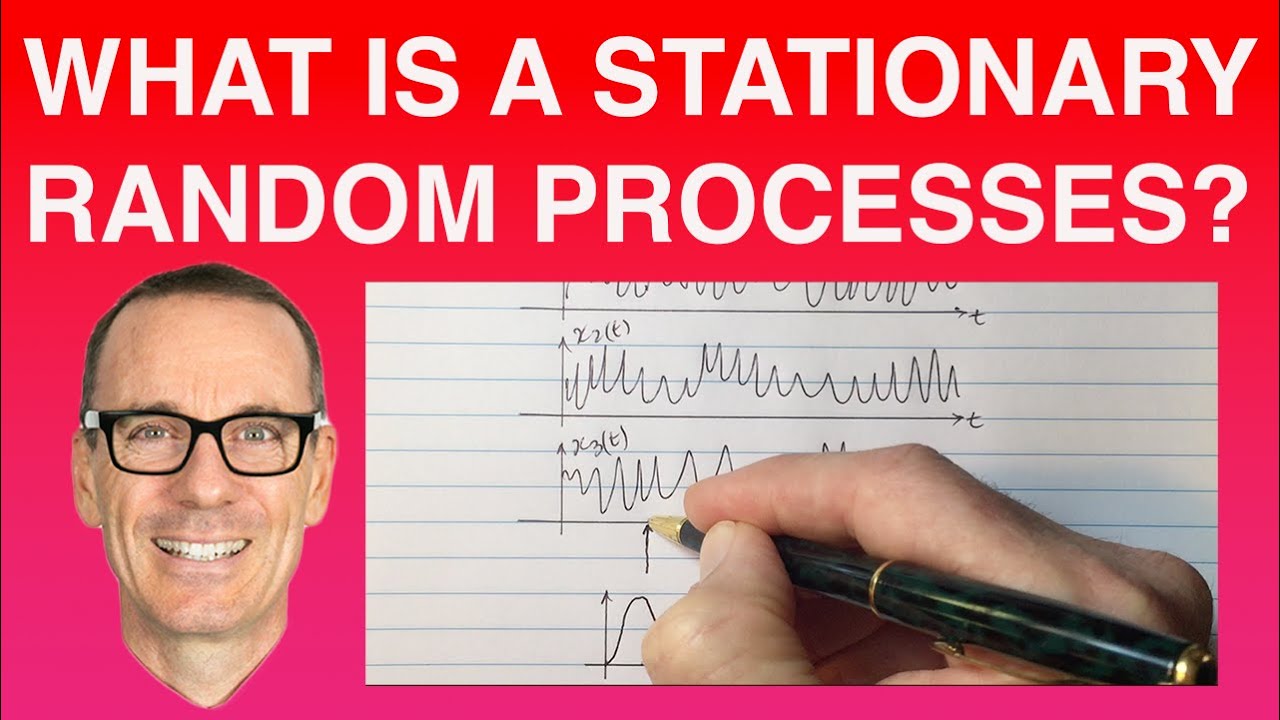
What is a Stationary Random Process?

Explains the concept of stationarity in random processes, using an example and diagrams.

* Note that I unfortunately forgot to mention that Stationarity also requires that the joint distribution of X(t1) and X(t2) is the same as the joint distribution of X(t1+Δ) and X(t2+Δ), for any t1, any t2, and for all Δ. In summary, this means that the way in which X(t1) and X(t2) are related, is the same as the way in which X(t1+Δ) and X(t2+Δ) are related. In other words, the level of dependency between two values that are spaced Δ apart, doesn't change over time. Or from yet another perspective, how _independent_ they are from each other, doesn't change over time.

* If you would like to support me to make these videos, you can join the Channel Membership, by hitting the "Join" button below the video, and making a contribution to support the cost of a coffee a month. It would be very much appreciated.

Check out my search for signals in everyday life, by following my social media feeds:

.

[payman_azari]

thanks Professor, even if I have some prior knowledge, your videos help me to rewire my brain.

[nawrasv]

Thanks a lot. Loved watching videos of yours, it helps to channelize my thoughts and help in better visualization

[palisthashrestha]

Really appreciate how you show things visually!

[anmolmathur]

Your videos are helping me in my Masters course !!

[andrus]

Thank you Professor. Waiting for more videos about statistical analysis of random processes

[GhulamRaza-lx]

Dear Iain, Your short videos are intuitive. Do you have any video on Random walk?

[khalifi]

More generally, for a stationary process, the joint distribution of X(t1)
and X(t2) is the same as the joint distribution of X(t1+Δ) and X(t2+Δ).
In particular, if a process is stationary, then its analysis is usually simpler as the probabilistic properties do not change by time.

[subrahmanyaswamyperuru]

Thank you for the wonderful videos, professor.

I have a minor comment regarding your note on joint distributions in the description:

The joint distribution w.r.t. any collection of time instants (not necessarily restricted to two time instants) should be invariant to time shifts, right?

[ImranMoezKhan]

If I understood correctly, this means the process PDF does not depend on time? Perhaps "static" random process would have been be a more appropriate when the term was being coined :-).

[pltcmod]

Hello Professor, thanks for the video. I have a question. Is it sufficient to say that a random variable is stationary if it looks like white noise when plotted against time?
Thanks in advance!

[HardMode-cz]

Question: By saying that the PDFs of the two RV are the same that does that mean the variance and the mean are the same? Or does it mean that the PDFs are either both Rayleigh or both Gaussian?

[seslocrit]

Could you do a video on how to construct the PDF? It makes intuitibe sense but I am having a hard time actually making it.

[tuongnguyen]

Does the fact that stationarity happen for short recording time play an important role in DSP ?