filmov
tv
Theorem for limits of composite functions: when conditions aren't met | AP Calculus | Khan Academy

Показать описание
Keep going! Check out the next lesson and practice what you’re learning:
Suppose we are looking for the limit of the composite function f(g(x)) at x=a. This limit would be equal to the value of f(L), where L is the limit of g(x) at x=a, under two conditions. First, that the limit of g(x) at x=a exists (and if so, let's say it equals L). Second, that f is continuous at x=L. If one of these conditions isn't met, we can't assume the limit is f(L).
Suppose we are looking for the limit of the composite function f(g(x)) at x=a. This limit would be equal to the value of f(L), where L is the limit of g(x) at x=a, under two conditions. First, that the limit of g(x) at x=a exists (and if so, let's say it equals L). Second, that f is continuous at x=L. If one of these conditions isn't met, we can't assume the limit is f(L).
Theorem for limits of composite functions | Limits and contiuity | AP Calculus | Khan Academy
Theorem for limits of composite functions: when conditions aren't met | AP Calculus | Khan Acad...
Limits of composite functions | Limits and continuity | AP Calculus AB | Khan Academy
Limit of a Composite Function Theorem: Proof
Limits 070 Composite Function Theorem
Determine the limit of a composite function using the composite function theorem
Properties of Limits
Limits of composite functions: internal limit doesn't exist | AP Calculus | Khan Academy
Squeeze Theorem
Determine the limit of a composite function using the composite function theorem
Infinite Limit vs Limits at Infinity of a Composite Function
This is a very famous limit
Theorem on Limit of a Composite Function
Determine the limit of a composite function using the composite function theorem
Limits of composite functions: external limit doesn't exist | AP Calculus | Khan Academy
Limit of Composite Functions (Theorem) | Calculus: Limits and Continuity
Limits of Oscillating Functions and the Squeeze Theorem
Limits of composite functions - How to find them?
1.3C Limits of Composite Functions and the Squeeze Theorem
2.4 Composite Function Theorem
How To Find The Limit At Infinity
Limits and Continuity
Piecewise Functions - Limits and Continuity | Calculus
Limits of Trigonometric Functions
Комментарии
 0:03:13
0:03:13
 0:03:22
0:03:22
 0:05:11
0:05:11
 0:07:55
0:07:55
 0:02:49
0:02:49
 0:01:37
0:01:37
 0:05:13
0:05:13
 0:04:17
0:04:17
 0:10:43
0:10:43
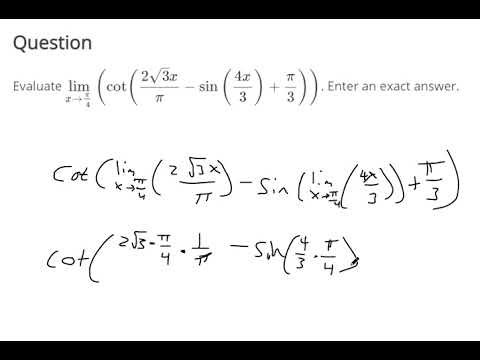 0:04:03
0:04:03
 0:09:50
0:09:50
 0:00:48
0:00:48
 0:09:05
0:09:05
 0:01:19
0:01:19
 0:03:25
0:03:25
 0:11:58
0:11:58
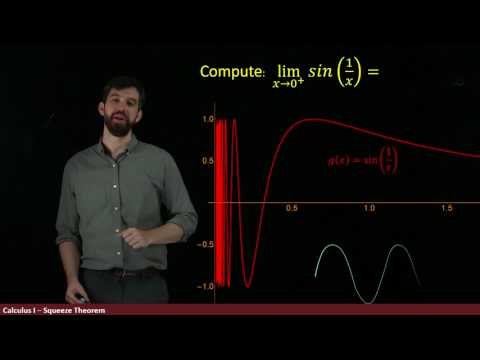 0:06:59
0:06:59
 0:22:06
0:22:06
 0:24:22
0:24:22
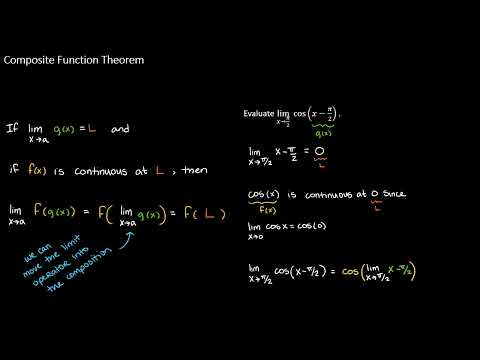 0:06:21
0:06:21
 0:13:14
0:13:14
 0:19:19
0:19:19
 0:10:06
0:10:06
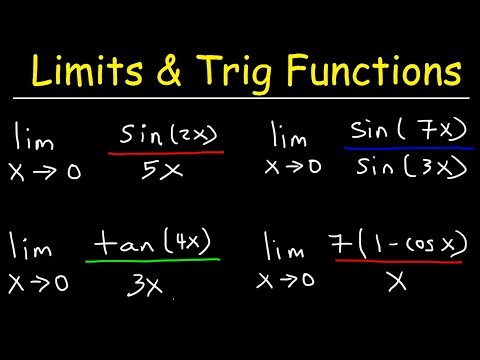 0:15:23
0:15:23