filmov
tv
Integration by completing the square (hard)
Показать описание
blackpenredpen,
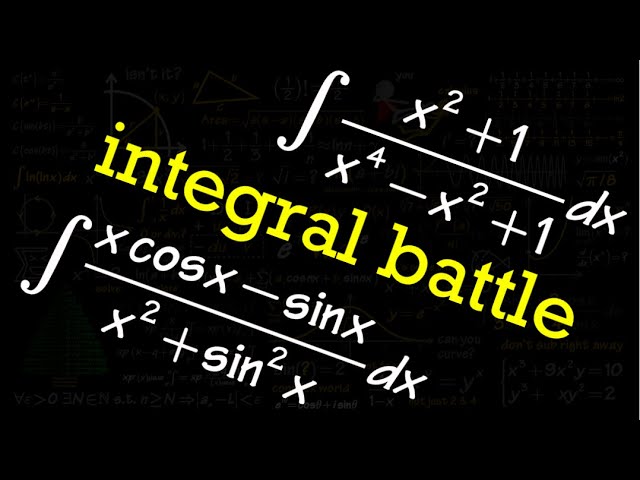
Integral battles!
Integration of Rational Functions By Completing The Square - Calculus
Integral by completing the square, and u sub, calculus 2
Integration by Completing the Square - Calculus AB
Integration using completing the square and the derivative of arctan(x) | Khan Academy
Integration by completing the square (hard)
Integration using completing the squares
Solve by completing the square | Step by Step Technique
Calculus Class: Integral by Completing the Square
INTEGRAL OF QUADRATIC EQUATIONS USING THE STANDARD FORMULAS
Integration by Completing the Square
How to Solve By Completing the Square (NancyPi)
integration with completing the square, Sect 7.4 #29
Integrating Functions by Completing the Square
The FASTEST Way To Complete The Square!! (in 48 seconds)
Integral of rational function by completing the square 2
Integration by Completing the Square
Mathematics N6 Integration - Completing the Square Method Introduction @mathszoneafricanmotives
Integration by Completing Square Method Problem No 2 - Integration - Diploma Maths - 2
Integration by Completing Square Method Problem No 1 - Integration - Diploma Maths - 2
Complete The Square for this Integral! #calculus #calculushelp #integrationshorttricks #mathtricks
5 7 Integrals that Require Completing the Square
Solving Quadratic Equations By Completing The Square
Evaluating a Definite Integral by Completing the Square
INTEGRATION SOLVED by COMPLETING THE SQUARE
Комментарии
 0:26:06
0:26:06
 0:10:13
0:10:13
 0:03:00
0:03:00
 0:05:27
0:05:27
 0:06:53
0:06:53
 0:09:51
0:09:51
 0:05:20
0:05:20
 0:18:43
0:18:43
 0:10:28
0:10:28
 1:19:00
1:19:00
 0:17:33
0:17:33
 0:06:04
0:06:04
 0:10:40
0:10:40
 0:00:49
0:00:49
 0:06:54
0:06:54
 0:08:01
0:08:01
 1:17:44
1:17:44
 0:05:09
0:05:09
 0:04:30
0:04:30
 0:01:01
0:01:01
 0:17:35
0:17:35
 0:07:57
0:07:57
 0:04:03
0:04:03
 0:09:49
0:09:49