filmov
tv
Line integral from vector calculus over a closed curve
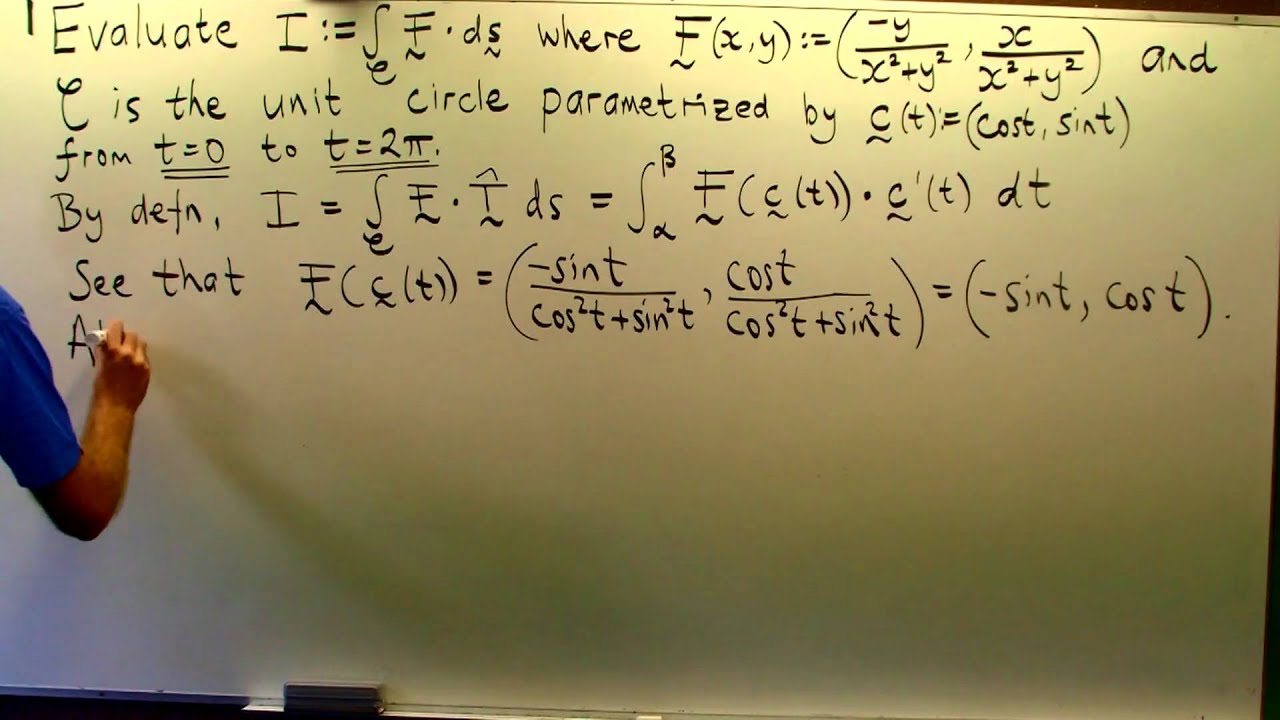
Показать описание
I present an example where I calculate the line integral of a given vector function over a closed curve.. In particular, I the vector function is a
$${\bf F}(x,y) := (-y/(x^2 + y^2), x/(x^2 + y^2)$$
and the closed curve is the unit circle, oriented in the anticlockwise direction.
I solve the problem and discuss the significance of the line integral through the mention of specific applications to engineering and physics.
Such an example is seen in second year university mathematics.
$${\bf F}(x,y) := (-y/(x^2 + y^2), x/(x^2 + y^2)$$
and the closed curve is the unit circle, oriented in the anticlockwise direction.
I solve the problem and discuss the significance of the line integral through the mention of specific applications to engineering and physics.
Such an example is seen in second year university mathematics.
Evaluating Line Integrals
Introduction to the line integral | Multivariable Calculus | Khan Academy
Calculus 3 Lecture 15.3: How to Compute Line Integrals (Over Non-Conservative V.Fields)
Calculus 3: Line Integrals (18 of 44) What is a Line Integral? [(y)dx+(z)dy+(x)dz] Example 6
How to Evaluate the Line Integral of a Vector Field
What is a LINE INTEGRAL? // Big Idea, Derivation & Formula
Line Integrals with respect to x or y // Vector Calculus
Line integrals and vector fields | Multivariable Calculus | Khan Academy
20: Scalar Field Line Integrals - Valuable Vector Calculus
Line Integrals of Vector Fields // Big Idea, Definition & Formula
Line integral example 1 | Line integrals and Green's theorem | Multivariable Calculus | Khan Ac...
The Fundamental Theorem of Line Integrals // Big Idea & Proof // Vector Calculus
Vector Calculus - Line Integrals of Vector Field | Example & Solution
Line Integral | Numerical | Vector Calculus | Maths
Line Integrals Are Simpler Than You Think
Beauty of Line Integral (Calculus) .
Line Integrals - Evaluating a Line Integral
Line Integrals on CONSERVATIVE Vector Fields (Independence of Path): Calculus 3 Lecture 15.4
Mass of a Wire with Line Integral - Vector Calculus Application
Conservative Vector Fields // Vector Calculus
VECTOR INTEGRAL | VECTOR CALCULUS | Line Integral | Lecture 01 | Part 1 | PRADEEP GIRI SIR
line integral of a vector function (KristaKingMath)
Line Integrals Practice Problems
Vector Integration | Line Integration | Vector Calculus 2.O by GP Sir
Комментарии
 0:12:54
0:12:54
 0:18:49
0:18:49
 2:17:24
2:17:24
 0:05:03
0:05:03
 0:06:16
0:06:16
 0:14:02
0:14:02
 0:11:28
0:11:28
 0:16:47
0:16:47
 0:12:47
0:12:47
 0:08:40
0:08:40
 0:13:03
0:13:03
 0:06:38
0:06:38
 0:23:18
0:23:18
 0:15:30
0:15:30
 0:21:02
0:21:02
 0:08:56
0:08:56
 0:08:24
0:08:24
 1:53:58
1:53:58
 0:06:46
0:06:46
 0:06:17
0:06:17
 0:22:32
0:22:32
 0:10:39
0:10:39
 0:13:00
0:13:00
 0:18:49
0:18:49