filmov
tv
Solving sin^2x+cos^3x=7/8
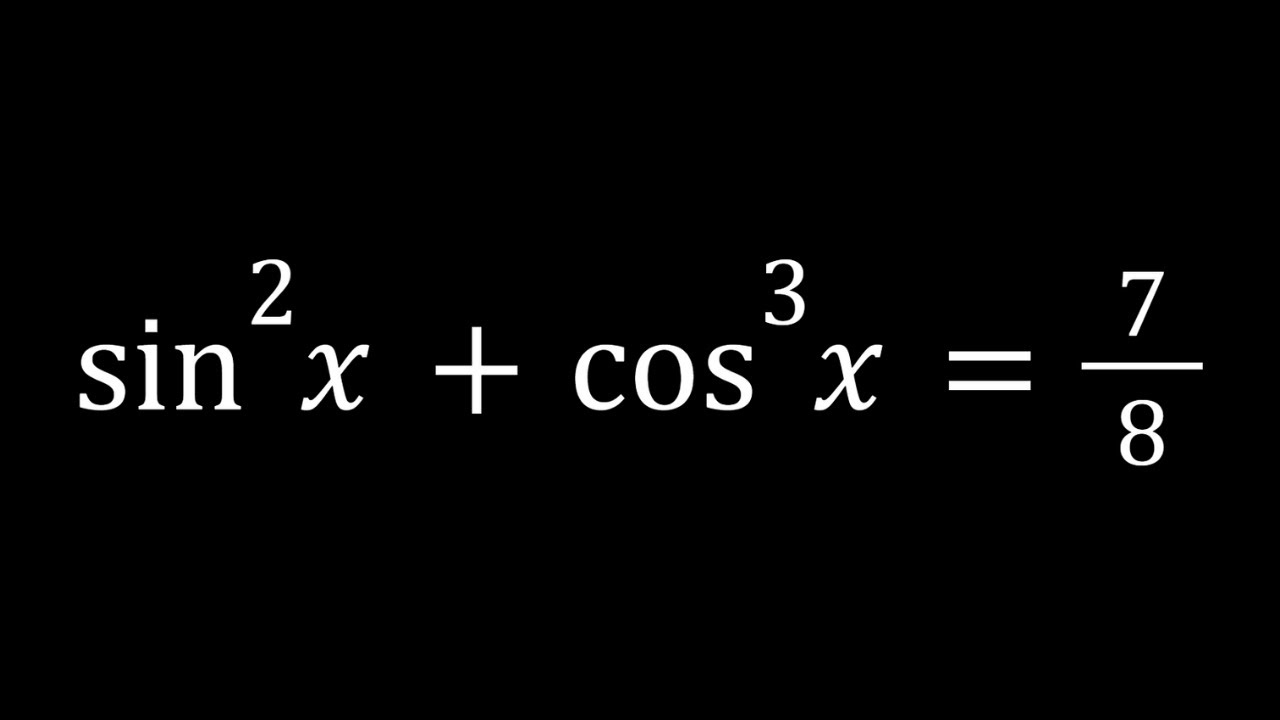
Показать описание
If you need to post a picture of your solution or idea:
#TrigonometryProblems #TrigonometricEquations
PLAYLISTS 🎵 :
#TrigonometryProblems #TrigonometricEquations
PLAYLISTS 🎵 :
Solving sin^2x+cos^3x=7/8
How to solve cos(3x)=sin(2x)
How To Solve Trigonometric Equations With Multiple Angles - Trigonometry
Trogonometric Equation | Sin 2x = Cos(3x-10°) | tan x = ?
NEWYES Calculator VS Casio calculator
When mathematicians get bored (ep1)
How REAL Men Integrate Functions
Find Trigonometric Equation from Solution cos 4x - cos 2x = 7/8
NABTEB 2024 Mathematics Objective questions
#Trigonometry all formulas
Solve sin=2/5 Trigonometric equation
Trigonometric Equation: Solve sin (2x) = cos (x)
Evaluate the integral. sin^(2)x cos^(3)x dx
Find the solutions to a trig equation between 0 and 2pi
Quadratic Equations IIT Questions No 05 ( X Class)
Calculate sin2x cos2x and tan2x for given tanx in Quadrant 2
INTEGRAL OF SIN2X COS3X dx | INTEGRAL OF SIN(2X)COS(3X) | INTEGRATION CLASS 12
Functions IIT Questions NO 11 ( X Class)
∫sin(2x)cos(3x) dx. MIT Integration Bee 2014, Question 6, Qualifying Exam.
Find sin ( 2x ), cos ( 2x ), tan (2x) , given sin ( x ) = 3/4
Finding the Period and Amplitude of a Graph
🤣Modi ji ne to math ki esi taisi kar diye🤣🙆🏻♂️ #shorts #youtubeshorts #viral
Trigonometric Integrals
JEE Aspirants ka Sach 💔 #JEE #JEEMain #Shorts
Комментарии
 0:09:36
0:09:36
 0:05:33
0:05:33
 0:11:34
0:11:34
 0:02:43
0:02:43
 0:00:14
0:00:14
 0:00:37
0:00:37
 0:00:35
0:00:35
 0:06:49
0:06:49
 1:56:19
1:56:19
 0:00:16
0:00:16
 0:04:04
0:04:04
 0:00:55
0:00:55
 0:04:40
0:04:40
 0:03:38
0:03:38
 0:00:57
0:00:57
 0:04:07
0:04:07
 0:04:12
0:04:12
 0:00:48
0:00:48
 0:00:52
0:00:52
 0:12:46
0:12:46
 0:01:59
0:01:59
 0:00:24
0:00:24
 0:31:29
0:31:29
 0:00:12
0:00:12