filmov
tv
Example of Chain Rule 3 - Trig Functions

Показать описание
Calculus: Find the derivative of the following trig functions: (a) f(x) = sin^4(x) + cos(4x), (b) f(x) = sec(x^2 + x + 1), and (c) f(x) = sin(cos(x)).
Example of Chain Rule 3 - Trig Functions
Using the Chain Rule - Harder Example #3
Calculus Chain Rule Example 3
Applying the chain rule twice | Advanced derivatives | AP Calculus AB | Khan Academy
Chain Rule For Finding Derivatives
Derivative using Chain Rule 3 Algebraic Examples 4K
Chain Rule Example 3 and Product Rule Example 5 (KristaKingMath)
The Multi-Variable Chain Rule: Derivatives of Compositions
Chain Rule Method of Differentiation | Derivatives
Chain Rule With Partial Derivatives - Multivariable Calculus
Calculus AB/BC – 3.1 The Chain Rule
Chain rule example using visual information | Differential Calculus | Khan Academy
Understand Chain Rule in 39.97 Seconds!
Derivatives Using the Chain Rule | 3 Examples (Part 1)
How to Differentiate e^(3x) with the Chain Rule | Calculus Derivatives #shorts
Multivariable chain rule
Chain Rule Derivative (Formula with Examples)
The Multivariable Chain Rule
Do You Remember How Partial Derivatives Work? 🤔 #Shorts #calculus #math #maths #mathematics
chain rule#shorts #physics #class11 #calculus #chainrule
Chain rule example using visual function definitions | Differential Calculus | Khan Academy
The Chain Rule... How? When? (NancyPi)
Calculus 3: Partial Derivative (29 of 50) The Chain Rule (Type 3): Example 1
MASTER Derivatives In Less Than A Minute!!
Комментарии
 0:03:05
0:03:05
 0:06:28
0:06:28
 0:01:17
0:01:17
 0:03:10
0:03:10
 0:18:40
0:18:40
 0:29:10
0:29:10
 0:07:49
0:07:49
 0:10:47
0:10:47
 0:06:01
0:06:01
 0:21:10
0:21:10
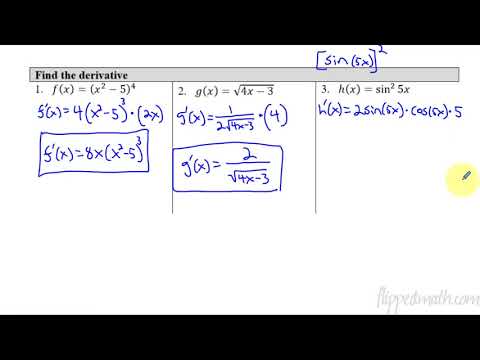 0:15:20
0:15:20
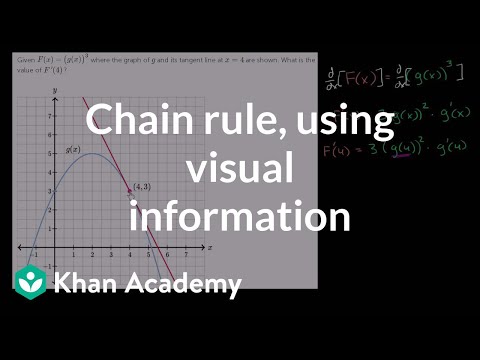 0:03:56
0:03:56
 0:00:42
0:00:42
 0:04:08
0:04:08
 0:00:13
0:00:13
 0:09:33
0:09:33
 0:03:56
0:03:56
 0:14:42
0:14:42
 0:00:26
0:00:26
 0:00:56
0:00:56
 0:05:25
0:05:25
 0:16:36
0:16:36
 0:04:56
0:04:56
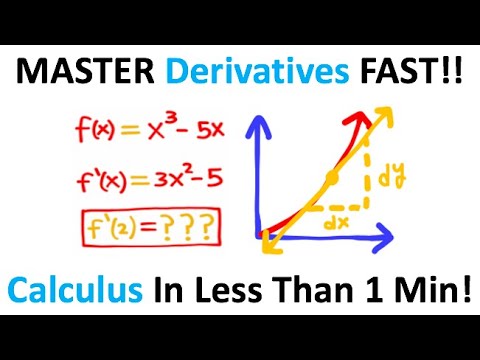 0:00:58
0:00:58