filmov
tv
Understand Chain Rule in 39.97 Seconds!

Показать описание
What is Chain Rule? How to differentiate using the Chain Rule?
The Chain Rule is used for finding the derivative of composite functions. For this example, it is impossible to expand the brackets one by one as the exponent is extremely large. Therefore, the Chain Rule is used here for differentiation. We can separate the function into inner and outer function to make the differentiation easier. First, differentiate the outer function using power rule without modifying the inner function. Then, multiplying it with the derivative of the inner function.
#shorts #differentiation #chainrule #calculus #math #maths #mathematics #additionalmathematics
Chain Rule Full Explanation Video:
The Chain Rule is used for finding the derivative of composite functions. For this example, it is impossible to expand the brackets one by one as the exponent is extremely large. Therefore, the Chain Rule is used here for differentiation. We can separate the function into inner and outer function to make the differentiation easier. First, differentiate the outer function using power rule without modifying the inner function. Then, multiplying it with the derivative of the inner function.
#shorts #differentiation #chainrule #calculus #math #maths #mathematics #additionalmathematics
Chain Rule Full Explanation Video:
Understand Chain Rule in 39.97 Seconds!
Calculus Chain Rule Explained
Human Calculator Solves World’s Longest Math Problem #shorts
Only for a Genius! Connect 1 to 1, 2 to 2 & 3 to 3 without crossing the lines! #math #youtube
💀What's the LAST Backrooms LEVEL!?⚠️☁️ #shorts
#golfswing #fyp #waitforit #followthrough
Attack on Real Life Female Titan!
Crazy tick removal? Or fake?
POV: you’re 6’9” 400 pounds and booked the middle seat
*Exclusive Video* Brutal Cartel Punishment
This trick can make your rubik's cube 2x faster😱🔥#ytshorts#shorts#drcuber
Science experiments that went wrong😳 #shorts
Where is this Shi huahua?😂😂🏞Perfect mismatch c - drama#k - pop
💀What is the RAREST Backrooms LEVEL!?⚠️🔑 #shorts
100 derivatives (in one take)
Everything You Need To Know When Replacing Your Motorcycle’s Chain and Sprockets | The Shop Manual
Tough times Never last 😊✌️ #delhipolice #motivation
Concise Modular Calculus [11/97]: Mean Value Theorem (4/5 on Derivitives)
Inside A Motorcycle Carburetor - Needle Height Tuning Adjustment | MC Garage
Dominik Mysterio steal Rey's mask 😳 #Short
How to use car lights correctly?
Composite Functions
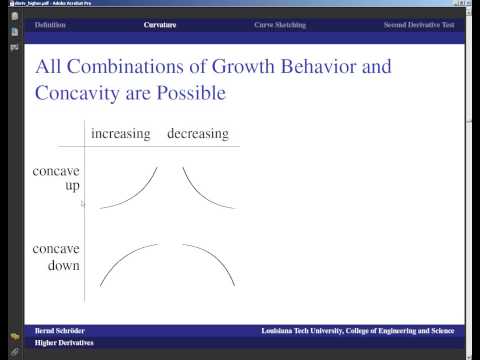
Concise Modular Calculus [12/97]: Higher Derivatives (5/5 on Derivatives)
She Gets A SURPRISE! 😱
Комментарии
 0:00:42
0:00:42
 0:13:54
0:13:54
 0:00:34
0:00:34
 0:00:20
0:00:20
 0:00:19
0:00:19
 0:00:18
0:00:18
 0:00:13
0:00:13
 0:00:11
0:00:11
 0:00:18
0:00:18
 0:00:13
0:00:13
 0:00:16
0:00:16
 0:00:32
0:00:32
 0:00:16
0:00:16
 0:00:15
0:00:15
 6:38:13
6:38:13
 0:07:15
0:07:15
 0:00:22
0:00:22
 0:17:58
0:17:58
 0:03:54
0:03:54
 0:00:15
0:00:15
 0:00:39
0:00:39
 0:05:23
0:05:23
 0:20:38
0:20:38
 0:00:23
0:00:23