Lesson 17: Geometric Distribution Part 1

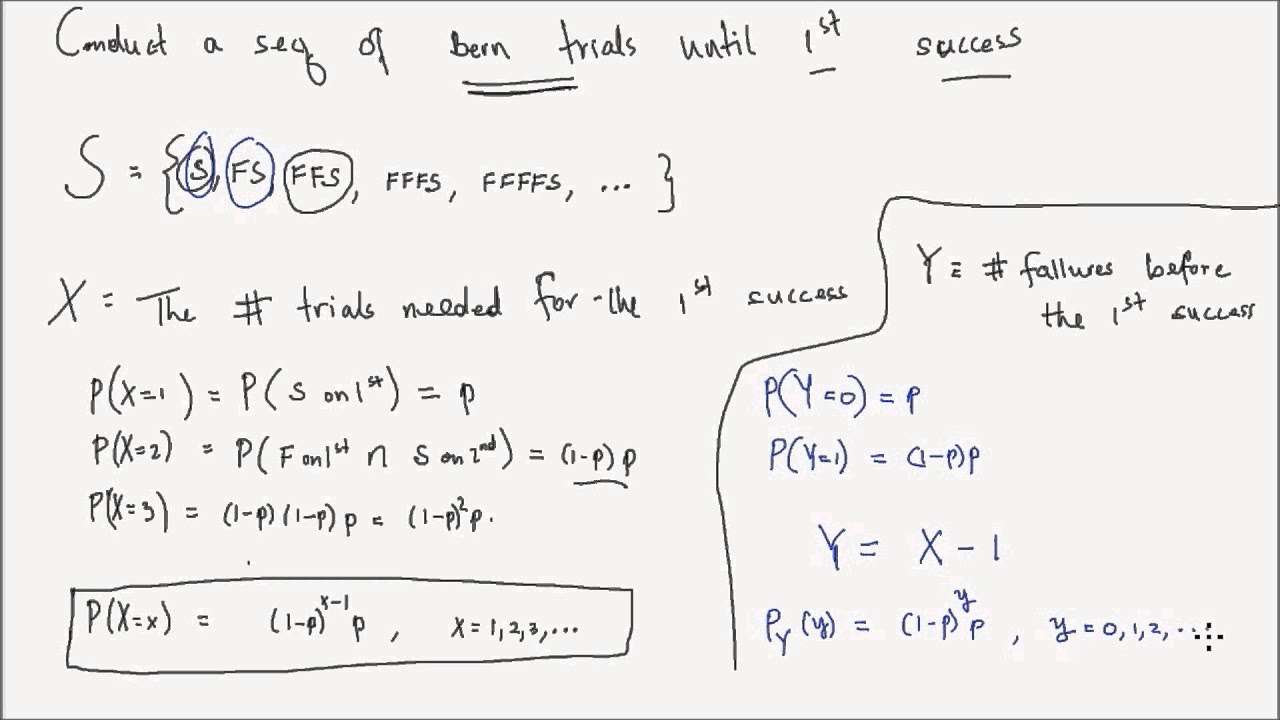
Introduction to Geometric Distribution. We give an intuitive introduction to the geometric random variable, outline its probability mass function, and cumulative distribution function.

[mrboyban]

Thank you so much, it is a great video! 
The only clear explanation on YouTube.

[toastersman]

For the CDF of the Geometric function, there is a faster way.

You can find the sum of a geometric series for r (ratio) between (-1, 1), by using the formula: S_n= a(1-r^n)/1-r where a is the first term of the serie. If you use this formula, it only takes one line of paper.

[danielaubertine]

Never thought I would say this, but I am starting to feel the Bern!

[iBomb]

this video is the tits, gonna credit this guy when im rolling in the dough of the actuarial life

[TheGirlfriendDimension]

thank you for showing the cdf proof this way! I was not getting it with the "shorter" way other videos were using!

[lolo]

I found this series of videos to be very helpful. Am an engineering student by the way :)

[_sona_malhotra]

Thank you so much for this video. I had been looking for the condition where geometric distribution is applied. In every video I watched, they only taught how a problem is solved using geometric distribution. None of them taught when this distribution is applied.
This video has helped me so much. Thank you for this😇

[YairCat]

Px(k) is not suppose to be Pk(k), I am not really sure

[laugernberg]

15:23 this is sometimes called the survivor function O: oh i bet i know what that means...