filmov
tv
Geometric Distributions and The Birthday Paradox: Crash Course Statistics #16

Показать описание
Geometric probabilities, and probabilities in general, allow us to guess how long we'll have to wait for something to happen. Today, we'll discuss how they can be used to figure out how many Bertie Bott's Every Flavour Beans you could eat before getting the dreaded vomit flavored bean, and how they can help us make decisions when there is a little uncertainty - like getting a Pikachu in a pack of Pokémon Cards! We'll finish off this unit on probability by taking a closer look at the Birthday Paradox (or birthday problem) which asks the question: how many people do you think need to be in a room for there to likely be a shared birthday? (It's likely much fewer than you would expect!)
Thanks to the following Patrons for their generous monthly contributions that help keep Crash Course free for everyone forever:
Mark Brouwer, Glenn Elliott, Justin Zingsheim, Jessica Wode, Eric Prestemon, Kathrin Benoit, Tom Trval, Jason Saslow, Nathan Taylor, Divonne Holmes à Court, Brian Thomas Gossett, Khaled El Shalakany, Indika Siriwardena, SR Foxley, Sam Ferguson, Yasenia Cruz, Eric Koslow, Caleb Weeks, Tim Curwick, Evren Türkmenoğlu, D.A. Noe, Shawn Arnold, mark austin, Ruth Perez, Malcolm Callis, Ken Penttinen, Advait Shinde, Cody Carpenter, Annamaria Herrera, William McGraw, Bader AlGhamdi, Vaso, Melissa Briski, Joey Quek, Andrei Krishkevich, Rachel Bright, Alex S, Mayumi Maeda, Kathy & Tim Philip, Montather, Jirat, Eric Kitchen, Moritz Schmidt, Ian Dundore, Chris Peters, Sandra Aft, Steve Marshall
--
Want to find Crash Course elsewhere on the internet?
Thanks to the following Patrons for their generous monthly contributions that help keep Crash Course free for everyone forever:
Mark Brouwer, Glenn Elliott, Justin Zingsheim, Jessica Wode, Eric Prestemon, Kathrin Benoit, Tom Trval, Jason Saslow, Nathan Taylor, Divonne Holmes à Court, Brian Thomas Gossett, Khaled El Shalakany, Indika Siriwardena, SR Foxley, Sam Ferguson, Yasenia Cruz, Eric Koslow, Caleb Weeks, Tim Curwick, Evren Türkmenoğlu, D.A. Noe, Shawn Arnold, mark austin, Ruth Perez, Malcolm Callis, Ken Penttinen, Advait Shinde, Cody Carpenter, Annamaria Herrera, William McGraw, Bader AlGhamdi, Vaso, Melissa Briski, Joey Quek, Andrei Krishkevich, Rachel Bright, Alex S, Mayumi Maeda, Kathy & Tim Philip, Montather, Jirat, Eric Kitchen, Moritz Schmidt, Ian Dundore, Chris Peters, Sandra Aft, Steve Marshall
--
Want to find Crash Course elsewhere on the internet?
Комментарии
 0:10:19
0:10:19
 0:10:48
0:10:48
 0:32:13
0:32:13
 0:04:07
0:04:07
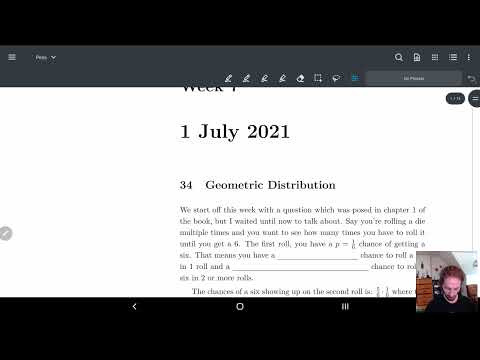 0:12:00
0:12:00
 0:11:04
0:11:04
 0:06:32
0:06:32
 0:06:15
0:06:15
 0:10:26
0:10:26
 0:07:07
0:07:07
 0:12:12
0:12:12
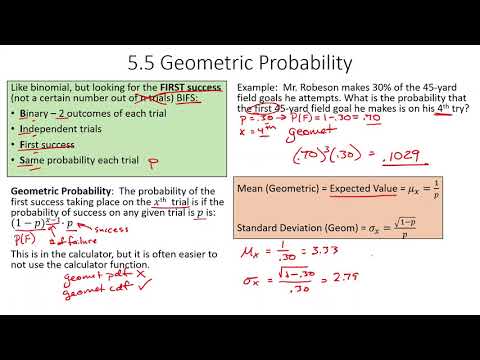 0:12:34
0:12:34
 0:18:21
0:18:21
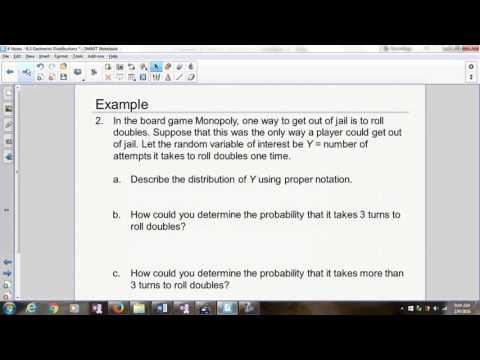 0:22:25
0:22:25
 0:07:19
0:07:19
 0:04:39
0:04:39
 0:05:54
0:05:54
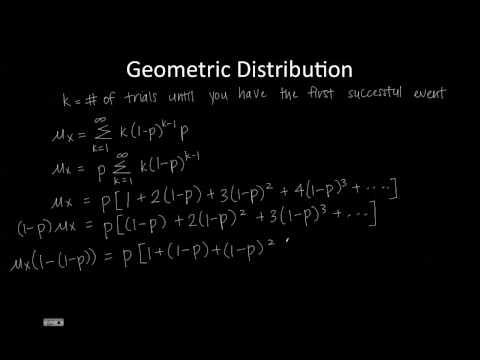 0:05:34
0:05:34
 0:03:33
0:03:33
 0:06:40
0:06:40
 0:04:00
0:04:00
 0:00:20
0:00:20
 0:07:24
0:07:24
 0:00:15
0:00:15