Geosynchronous Orbits are WEIRD

This video is about the physics of geosynchronous and geostationary orbits, why they exist, when they don't, when they're useful for communication/satellite TV, etc.

REFERENCES

Fraction of a sphere that's visible from a given distance

Orbital period

Kepler's third law

Kepler's 3rd law (which can be derived from Newton's law of gravitation and the centripetal force necessary for orbit as mr\omega^2=G\frac{mM}{r^2}, and using \omega=\frac{2\pi}{T}) is
T = 2pi Sqrt(r^3/(GM)) where M is the mass of the central object, G is the gravitational constant. Alternatively, we can solve for r, r = (T^2/(4pi^2) GM)^(1/3) ~ T^(2/3)/M^(1/3) = (T^2/M)^(1/3).

There is a limit (kind of like the Roche limit but for rotations). A rotating solid steel ball or other chunk of metal that has tensile strength (ie that isn't just a pile of stuff held together by gravity like most planets) would be able to spin faster.

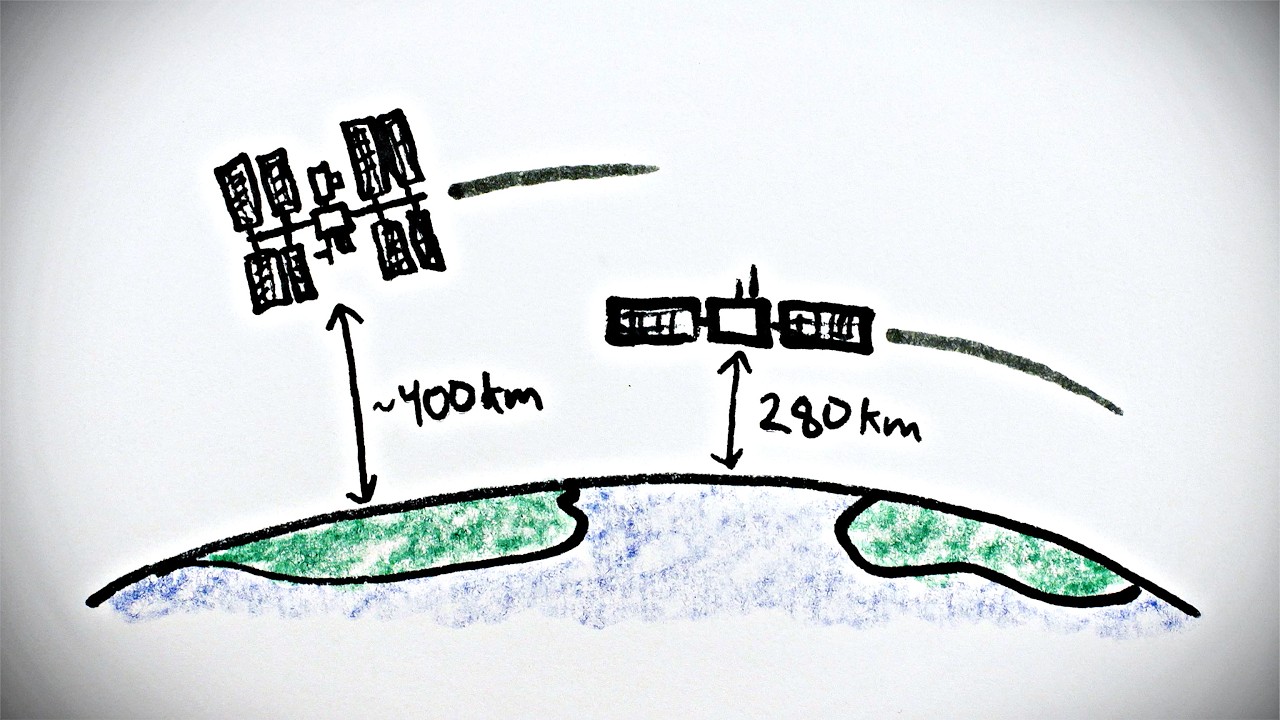
Calculate how much of a planet's surface you can see from a given geosynchronous orbit/radius? (Obviously for lower ones you can see less, etc) - d/(2(R+d)) where d is distance to surface, ie, R is sphere radius, R+d is object radius from sphere center.

Let's plug that in with r being the geostationary orbit radius. That is, we have \frac{1}{2} \left(1- \left(\frac{4 \pi^2 R^3}{T^2 G M }\right)^{1/3}\right)

Average density of a sphere \rho is given by \rho =M/(\frac{4}{3}\pi R^3), ie \rho=\frac{3M}{4 \pi R^3} aka

\frac{M}{R^3}=\frac{4}{3}\pi \rho.

So we can convert the "fraction of planet surface seen" to

\frac{1}{2} \left(1- \left(\frac{3 \pi}{G \rho T^2}\right)^{1/3}\right)

So as either \rho or T\to \infty, the fraction goes to a maximum of \frac{1}{2}. And the point of "singularity" where the orbit coincides with the surface is where G\rho T^2=3\pi, aka \rho=\frac{3\pi}{GT^2}. For a rotation period of 3600s, that corresponds to a density \rho \approx 11000kg/m^3, which is roughly twice the density of the earth. For a rotation period of 5400s, we have \rho\approx 4800kg/m^3, which is basically the density of the earth.

Alternately, if we plug the density of the earth in to an orbit of period 5400s, we get as a fraction of the planet seen:

\frac{1}{2} \left(1- \left(\frac{3 \pi}{G \rho T^2}\right)^{1/3}\right) = 0.02

aka 2\% of the earth's surface.


MinutePhysics is on twitter - @minutephysics

Minute Physics provides an energetic and entertaining view of old and new problems in physics -- all in a minute!

Created by Henry Reich

[Convergant]

One thing excluded from the video: if a body rotates REALLY slowly, you would never be able to have a geosynchronous orbit because of being disrupted by whatever it's orbiting (in technical terms, being outside its Hill Sphere). For example, a stationary orbit around Mercury would have a radius of 656, 231km, but Mercury's influence only extends out to 175, 300km, so even if you placed yourself that far from Mercury, you would be orbiting the Sun instead.

[smartereveryday]

The animations in this video are fantastic.

[iudqwninnaismck]

my favourite thing is that the content hasn't changed in so many years of uploading. The music, length and format are all perfect and work together so well

[brachypelmasmith]

fun fact. Moon doesn't have selenocentric orbit. At the distance one should be, you are no longer bonded to the moon but rather to the earth. Also, most lunar orbits are unstable since moon's gravity is unevenly distributed enough that it interferes with low orbits.

[wavey]

Technically satelite TV wouldn't be too hard to implement even with a long delay of geosync orbit. Since it's just pure upload and download, your TV will simply be delayed by the time it takes to travel. Which isn't a big deal because if you're watching news, does it matter that you got your news 1.5 mins later than someone else? The issue comes when you're talking about communication satellites. Can you imagine having 15, 000ms of latency?

[KitagumaIgen]

Lag-time aren't too problematic for TV use. That is mostly one-way transport of information, and as long as there are no faster channel even the deciding point in a tennis-match doesn't get spoilt. The problem is two-way communication where the lag-time becomes too annoying.

[x]

I wonder if there are other planetary properties for which we aren't in the goldilocks zone, but other planets are

[AlexKnauth]

The other problem with a "Venus-syncronous" orbit would be that it would be unstable: it would need to be so far out as outside Venus's sphere of influence, and so it would start orbiting the Sun instead of Venus.

Maybe some sort of Distant Retrograde Orbit (similar to Artemis 1) could be "synchronous" but it wouldn't be anywhere close to circular, iiuc it would be sort of "bean-shaped"? and more importantly its speed would change radically at different points of the orbit, moving faster on the day side and slower on the night side. And if that's the case I doubt it would be useful for constant communications with regions of Venus.

Edit: Come to think of it, the kind of DRO I'm thinking of being syncronous might not be possible in certain situations. If it's so far out that Venus's gravity isn't a major factor, then it would need to be on an elliptical orbit around the Sun that happens to have the same orbital period as Venus's year. Which would be incompatible with Venus's day unless the ratio between Venus's year/day is just right.

[Blackmark]

"We happen to live on a planet... in the Goldilock zone for satellite TV."
I just learned something this minute.

[jasper]

Wait... for satellite TV, a few extra seconds delay doesn't matter, does it?

(Signal strength might be an issue though, I don't know about that. And things like (video) calls and internet in general are a different story, but it's hard tot argue we're in the goldilocks zone for those.)

[AlexE]

It’s interesting to think of the geostationary radius as the maximum size of earth before chucks of rock get flung off

[extremjulius]

Why wouldn't satellite TV work with a 10 second delay? Live events would have a slight delay but my favorite series could very well be seen as long as the signal is continuous.

[parzingtheasian]

its been so many years and his content has never changed, and i love that

[ThePiachu]

Geostationary and geosynchronous orbits are fun, but you know what's even cooler? Sun-synchronous orbits! *Almost* at 90 degrees they use the hecking fact that Earth is a bit wider around the Equator to give themselves a little bit of rotation each orbit to stay in-sync with Earth moving around the Sun. It's a really weird quirk of orbital mechanics that lets them do that and it's so cool! Would love to see a video about that from you :D.

[GabeMisura]

Would you be weightless if the geosynchronous orbit is at the surface?

[Koisheep]

I need Hourphysics, an extended version where Henry just reads all those side notes you can only catch for a fraction of a second in the cc AND goes full ham into the details

[torazis]

Not only satellite at 280 km altitude would cover 2% of the Earth's surface, but it also would be subjected to atmospheric drag. ISS is constantly pushed up, imagine hundreds of satellites that are even lower.

[IOtheFifth]

You didn't mention the case in which synchronous orbit doesn't exist because a body is spinning so slow the resulting orbit is far outside body's sphere of influence and therefor unstable. Usually the case with tidally locked bodies like the Moon or Mercury.

[adamhlj]

When I was growing up, we had one of those old-school satellite tv dishes that had to physically rotate to be directed at the satellite in orbit. I'm assuming those satellites were geosynchronous because once it pointed in the direction of the satellite, it didn't move, haha. And I got hooked on TV at a young age.

[maxmisterman]

3:39 Corection: satelite tv should be one of the things that works without problem, the delay is between the filming of stuff and the timing of you wathing it and normally not randomly between signals. So you would see the sportsgame next door an hour late, but watching it smoothly. even normal streaming of live tv has delays so that a thing we are already planning around: it would just take longer. Moon missions already have the problem of relativly long delay.