filmov
tv
Linear Transformations: Find where T maps standard basis vector | Linear Algebra
Показать описание
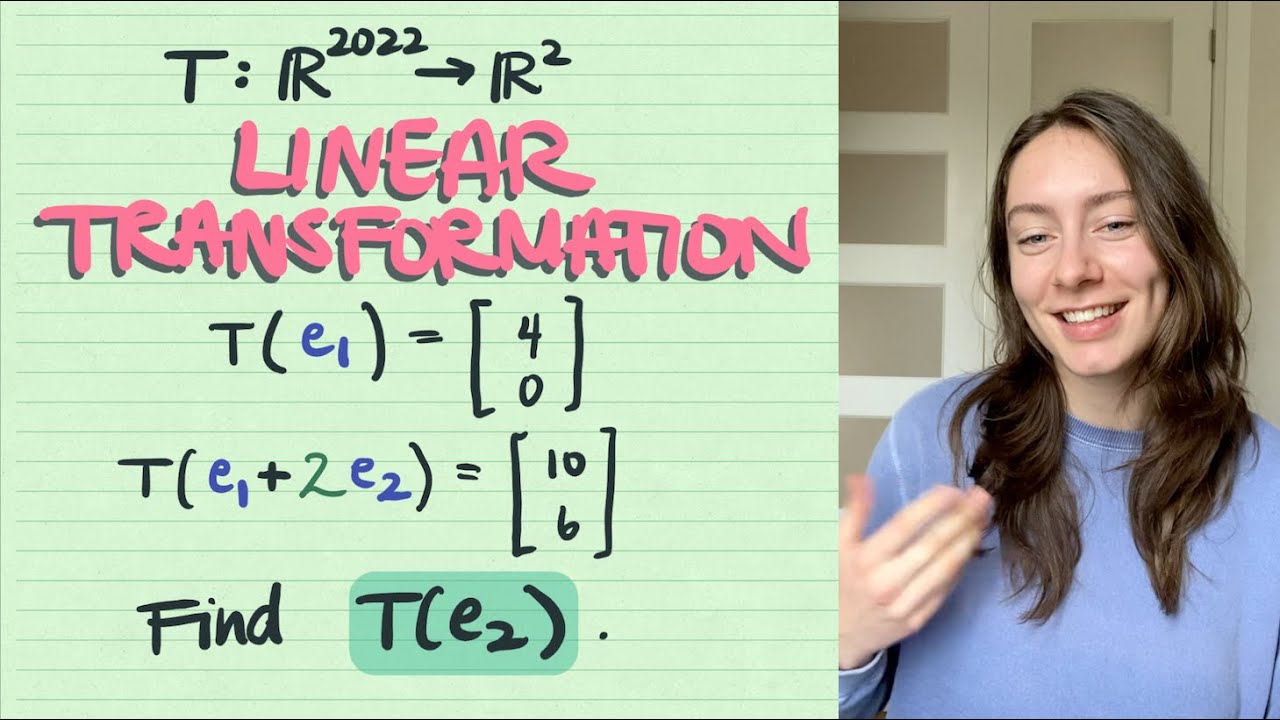
What is a linear transformation? You can think of it as a function from one space to another, that has a special property of being linear. In our case the linear transformation maps from 2022-dimensional space to 2-dimensional space (the plane). We're given two pieces of information about where our transformation takes linear combinations of basis vectors and we need to find where it takes e_2, the second standard basis vector. I explain what linear means and how to find T(e_2) .
If you found this video helpful, check out my other videos on linear algebra:
Good luck with your studying :)
If you found this video helpful, check out my other videos on linear algebra:
Good luck with your studying :)
0:05:19
How to Find the Matrix of a Linear Transformation
0:07:36
Linear Transformations: Find where T maps standard basis vector | Linear Algebra
0:09:06
Linear Transformations , Example 1, Part 1 of 2
0:13:52
Linear transformations | Matrix transformations | Linear Algebra | Khan Academy
0:01:34
Find a Linear Transformation Matrix (Standard Matrix) Given T(e1) and T(e2) (R2 to R3)
0:10:59
Linear transformations and matrices | Chapter 3, Essence of linear algebra
0:05:52
Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #1
0:08:04
Find formula for a linear transformation and find bases for kernel and range of the transformation
0:13:32
Inverse Linear Transformations | Linear Algebra
0:07:40
Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #2
0:06:18
Given a linear transformation, find the matrix of the linear transformation
0:09:11
Linear Transformations on Vector Spaces
0:06:09
Given images of a linear transformation, find another image
0:03:48
Find a Linear Transformation of a Vector Given T(x) and T(y) (R2 to R3)
0:08:25
Given a linear transformation, find the kernel and range
0:06:06
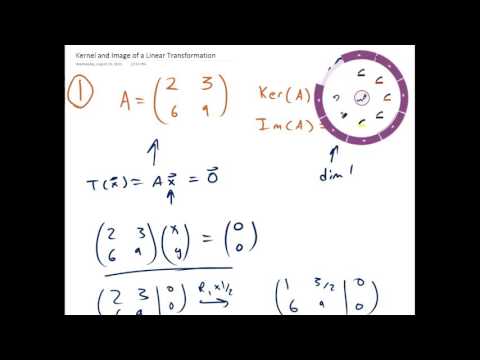
Kernel and Image of a Linear Transformation Example 1 | Linear Algebra | Griti
0:04:27
Given a linear transformation, find an image and a preimage
0:04:09
Standard Matrix - Full Example Explained
0:18:02
Transformation matrix with respect to a basis | Linear Algebra | Khan Academy
0:20:19
Finding Basis of Kernel and Range of a Linear Transformation - Linear Algebra
0:05:24
10. Find the linear transformation for given T(1,1,1)=(1,2) , T(1,2,1)=(3,4) and T(0,0,1)=(1,5)
0:16:37
im(T): Image of a transformation | Matrix transformations | Linear Algebra | Khan Academy
0:08:18
Finding matrices of compositions of linear transformations
0:11:22
Linear Transformations
Комментарии