Binomial Distribution - 10 - To find n, p and q of Binomial Distribution from its Mean and Variance

preview_player
Показать описание
How to find n, p and q of a binomial distribution when its mean and variance or SD are known?

Case / Question:
(A) "For a Binomial Distribution mean is 7 and variance is 11" - Comment.

(B) The mean and variance of a Binomial Distribution are 15 and 6 respectively. Find n, p and q.

#Statistics #Binomial #Distribution #Mean #Variance #Standard #Deviation #Probability

Statistics-GRE-GMAT-CAT-MBA-PGDBA-PGDM-BBA-Bcom -CA-CMA-CS-CWA-CPA - Mcom-Grade 11-Grade 12- FIII - CAIIB - IAS - UPSC - RRB - Competitive Exams - Entrance Exams

  1. PUAAR Academy
  2. Binomial Distribution
  3. Probability Distribution
  4. Probability
  5. Statistics
  6. GRE
Рекомендации по теме
Binomial distributions | 0:12:34

Binomial distributions | Probabilities of probabilities, part 1

A Secret Weapon 0:15:18

A Secret Weapon for Predicting Outcomes: The Binomial Distribution

Finding The Probability 0:20:27

Finding The Probability of a Binomial Distribution Plus Mean & Standard Deviation

Binomial distribution | 0:11:51

Binomial distribution | Probability and Statistics | Khan Academy

Binomial Distribution EXPLAINED 0:13:30

Binomial Distribution EXPLAINED in UNDER 15 MINUTES!

Binomial Distribution EXPLAINED 0:09:08

Binomial Distribution EXPLAINED with Examples

Visualizing the Binomial 0:07:22

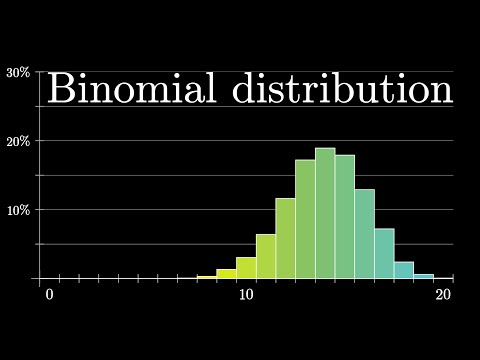
Visualizing the Binomial Distribution (6.6)

Probability using binomial 0:00:05

Probability using binomial distribution

29. Geometric distribution 0:11:56

29. Geometric distribution in statistics #geometricdistribution #probabilitydistribution

Binomial distribution probability 0:07:32

Binomial distribution probability (solve with easy steps)

10. Binomial Distribution 0:07:30

10. Binomial Distribution | Concept and Problem#9

Poisson distribution ,Binomial 0:00:55

Poisson distribution ,Binomial distribution & Probability #binomialdistribution #poissondistribu...

Fun 10: Binomial 0:19:23

Fun 10: Binomial Distribution Probability| Shortcut Trick| Solve in 60Sec

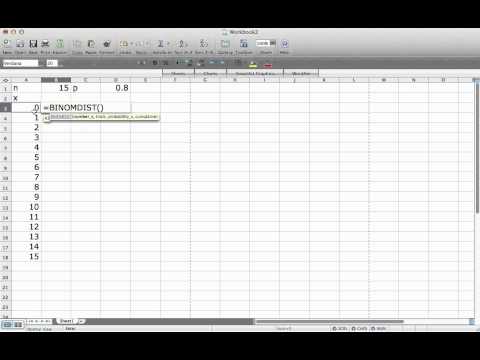
Computing the Binomial 0:03:34

Computing the Binomial Distribution with Excel

[IB Math] Normal 0:34:40

[IB Math] Normal and Binomial distribution questions - AA and AI SL

Probability: Binomial Distribution 0:06:51

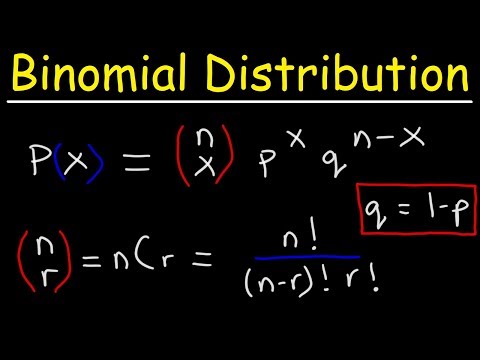
Probability: Binomial Distribution

The Binomial Experiment 0:10:06

The Binomial Experiment and the Binomial Formula (6.5)

Fastest way to 0:01:00

Fastest way to calculate probabilities for Binomial distribution by using fx-570/991ES, fx-570VN

Binomial distributions made 0:05:54

Binomial distributions made easy

The Normal Approximation 0:14:10

The Normal Approximation to the Binomial Distribution

Normal Distribution 10 0:10:32

Normal Distribution 10 • Mix of Binomial and Normal exam question • Stats2 Ex3F • 🎲

Binomial Probability Distribution 0:19:34

Binomial Probability Distribution

Binomial Distribution Problem 0:18:16

Binomial Distribution Problem 10 - Probability Distribution - Engineering Mathematics - 4

Binomial Probability Selecting 0:05:25

Binomial Probability Selecting a Sample with At Leat One Defect

Комментарии
Автор

Thanks very much sir for this video is very helpful for me in exam.

srjvideosnjoy
Автор

I didnt read anywhere about npq/np=q, but it works.

osamakhaan
Автор

Thank you very much sir. Very snappy dresser if i dare say so, sir.

legolad
Автор

plz solve this
Create the mathematical model of the following communication system problem and compute the desired result
using MATLAB software.
There are 100 people who want to connect to a certain communication system through k channels. The
probability that a person wants to connect to this system is 0.80. A channel can only be used by one person for
communication purpose at a given time. A connection failure occurs if a person wants to connect to the system
but all the channels are occupied by other users. What is the minimal value of variable k if the probability for
connection failure is less than 1%? (Hint: Random variable X follows Binomial distribution in this problem)

usmanshaukat
Автор

How to find p and n when mean and standard deviation is given

Kidsworld
Автор

plz solve
Create the mathematical model of the following communication system problem and compute the desired result
using MATLAB software.
There are 100 people who want to connect to a certain communication system through k channels. The
probability that a person wants to connect to this system is 0.80. A channel can only be used by one person for
communication purpose at a given time. A connection failure occurs if a person wants to connect to the system
but all the channels are occupied by other users. What is the minimal value of variable k if the probability for
connection failure is less than 1%? (Hint: Random variable X follows Binomial distribution in this problem)

usmanshaukat