Applying Sacred Geometry to Music

**UPDATE**
HEY! Thank you for all the comments and votes, if you want to see the rest of my notebook in another video, please share this video and donate to the cause! Venmo @smirkstudios

By the way, I read everything and am prepared to bring you another video soon! Please check back.
***
Original post:
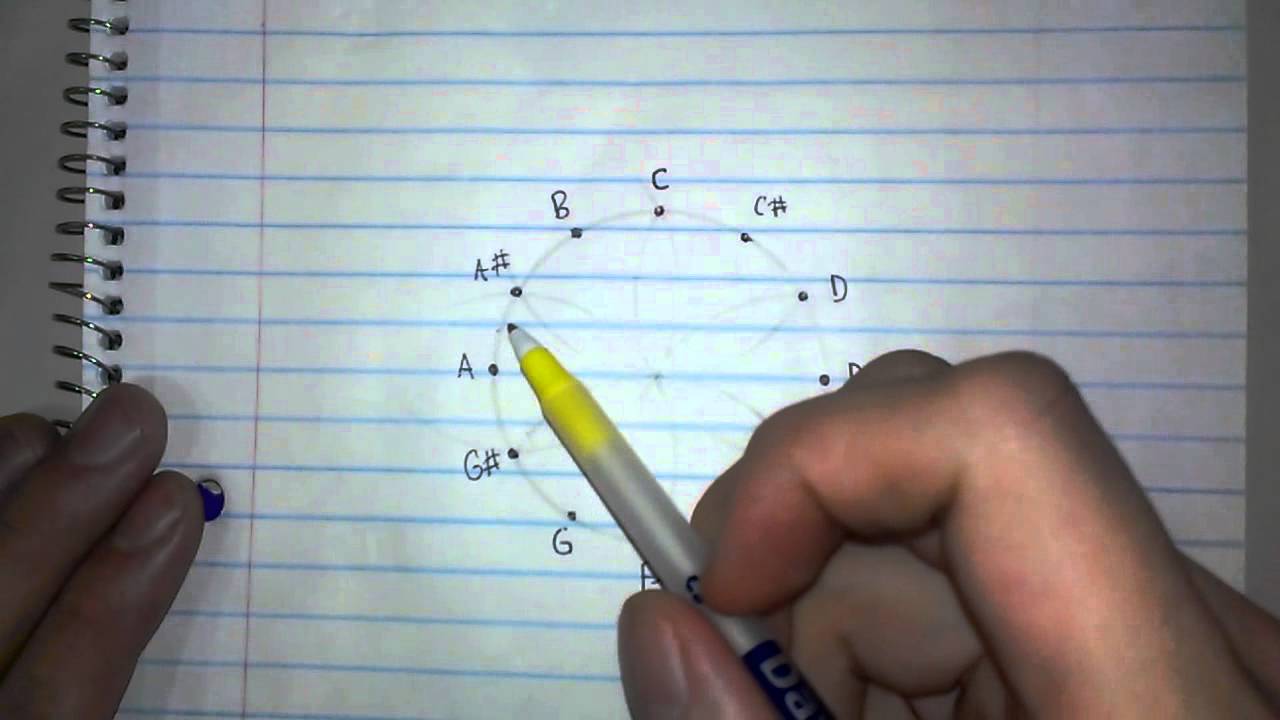
Here is an outline of how you can relate the 12 note Chromatic Scale of Western Music to the fundamentals of Sacred Geometry and the Flower of Life!

Sorry for the buzzing noise, I recorded this with my phone and that noise is my notifications vibrating... I'll turn it on Airplane Mode next time!

[etimruiz]

Hexagon in the circle in music theory is known as a 'whole tone scale' For examples check Debussy, in jazz it was part of Thelonious Monk's vibe

[HylanWeddington]

"I found this ruler on the side of the road, little beat but it's still good."
Gold.

[roflcakes]

I liked your video, this is a topic i find fascinating! 
Another fun fact that i have noticed is that  if you follow one side of the flower pedal and cross over onto the other side in the middle as you go across, it makes a Sine wave, which is the fundamental note in a chord, and if you implement the other sine waves, then you start adding harmonics and making chords, very cool stuff, thanks for sharing!

[erikgallegos]

I have been deeply interested in the concept of viewing our world in higher dimensions, so I tried to bring the concept of shapes in music. Then I tried to bring the concept of perceiving different shapes in "different dimensions" towards music. Then it brought me towards this concept. A buddy of mine and I discussed this for weeks and then I finally remembered sacred geometry and the fact that we have the internet, then I found this video, haha. All I can say is thank you. This basic explanation has become a tool that I'm going to use in so many ways that I never imagined being able to grasp. Excellent creativity.

[RandyLeeMcMillan]

I wrote an essay on this (20+ years ago) called Hermetic Music for the 21st Century Musician...I'll have to post links here when I get a chance. I created a whole system for "Pentacles of Aural Symetry" and "Septacles of Aural Symetry" with different species...

There is a lot more to this...for instance...the different species I defined wound have different complimentary characteristics to each other. So you can create different textures by mixing the penticles etc...

[zacdagypsy]

The CDEF#G#A# scale is a whole tone scale, best examples in DeBussy's work, jazz music ... Star Trek teleport sound FX features a whole tone scale. Sacred doodling ...

[spacevspitch]

I'm glad you mentioned the potential of other tunings. I was gonna say, the 12 note western equal tempered ("tampered" 😛) scale is actually an unnatural bastardization of the natural harmonic series.

So, I'd say this is just making awesome patterns with 12 equidistant points around a circle.

[djheatherbatlanta]

Dude this is really really awesome great explanation easy to follow I love it this is exactly how I compose music and how I bounce around the music notes. I can literally just see a sacred geometry figure in my head and then use that as my progression in scale and don’t have to count or remember what timing I am using because as long as I can see the shape in my head I always know exactly to break and change things up. I am extremely simply use this method for gigs played out. Awesome job!

[adriasorensen]

The edges of two circles connect in the center of a third circle in between the two, and the edges of the third circle are the centers of the first two circles drawn side by side (almost like a figure 8 aideways. ♾ or perhaps something that looks like this 00 or ⭕⭕ with the two circles side bynside). The centers go to the edge, and the edges go to the center in sacred geometry when adding to it. Just like with music, when you add a sharp or flat to a scale, it contains half the scale of the scale with one less flat and half the scale of the scale that has one more sharp.
(Notes for scale with one flat)
F G A Bb - CDEF

You take the second half of the scale above for the scale without any flats or sharps which is C major.
CdEF.

Then you take the first half of G major scale (which is the scale with an added sharp or one sharp) to use aa the last half of the C majpr scale.

(Last half of scale with one flat= CDEF

CdEF. - GABC

GABC = first half of scale with one sharp (which is GABC - D E F# G).

So the center notes of C major scale F and G become the ends or beginning points or notes of next scale going both ways ( to add a sharp or a flat for F scale and G scale).
Does that make sense. This correlates with the sacred geometry where the centers of a circle becomes the edges of 2 circles going out both ways fron each side of the first circle.

[HunnitAcreWoods]

have you made any interesting melodies/chord progressions or is this really just a model for memorizing notes of a given scale??

[munciemusiccenter]

11:39 i would like to know why you chose the points you chose for those scales

[BassdropTheory]

thank you so much for taking the time to make this video. my music appreciates it :)

[DemonFang]

This video got me into a pretty big music theory monologue, long post ahead:
This is definitely a cool experiment because of of how tonality is linear as much as it is circular, which allows geometry and math to intersect with music theory in interesting ways as you've demonstrated via this exploration of polygons in relation to the 12 half-step scale represented as a circle.

You also made note that this representation is similar to, but also different from, the circle of fifths. While it still adheres to the chromatic 12 semitone scale, the note increment and double circle band design of the circle of fifths was structured under the observation that all tones are actually accompanied by an infinite collection of fainter, higher, frequencies centered around the root frequency. These are just other frequencies that are basically "produced" by the presence of a fundamental/frequency alone (many can be isolated/heard, look up the harmonic/overtone series demonstration by Leonard Bernstein) and are what define the very science of what musically sounds "right" and "wrong" to the human ear.

This is because all sounds in nature produce harmonic frequencies (sometimes called overtones); the only way to produce a tone without harmonic frequencies occurring is via sine waves (more math)!

When a sound has seemingly no structure between the root frequency and the harmonic frequencies around it, it sounds like noise to our ears.

If the relationship (tonal distance) between the root frequency and its harmonic frequencies are in the right ratios (known as stable/natural relationships) then they sound like "actual musical tones."

The easiest way to understand why harmonic frequencies are always present and what makes them "natural" is to look at how sound is produced on a material level with anything vibration-related such as strings on a guitar.

The properties of how a string vibrates after being plucked is actually kind of complex:
When you pluck an open string, let's say the low E string, the string only "ripples" down the its /entire/ length once before the wave energy doubles back from both ends of the string and is now rippling/vibrating in two equal halves (an octave apart/of the root) of the string length, then rippling into a ratio of equal thirds of the string length (this is the first "different" overtone present, known as the dominant/5th note in a major scale), then into a ratio of fourths of the string length (another octave of the root), then into fifths of the string length (known as the 3rd in major scales), then into sixths and etc.- all of which are producing sound along with the root tone but just fainter and much higher as they are using geometrically smaller and smaller pieces of the original length of the plucked string.

This entire concept is the basis of how the major scale sounds "right" (it uses frequency ratios that match the harmonic frequencies of stable musical tones), why the dominant is known as such since they are naturally related to its root and play a core role in defining the tonal center of anything, and why the circle of fifths is incremented the way it is. When going clockwise you advance by 5ths (in scale interval terms) and go down by 4ths counter-clockwise.

The inner circle is made up of the notes that are the "relative key" the outer-ring's collection of major root notes. Meaning, that when using the major "note selection pattern" (whole and half steps, i.e. WWHWWWH) and start with C, then you would also "choose" the same notes using the minor "note selection pattern" (WHWWHWW) and starting on A. This is why A minor (Am) is below C on the circle of fifths (they are essentially the same key with just different center points), and since the majority of music is in a major/minor key, designing it this way allows us shortcuts:

When you take any 3x2 chunk of the circle of fifths (any 3 connected increments of the outer ring on top of the 3 connected increments directly below it), your middle note out of the connected group of 3 in the outer ring will be your major tonal center (let's stick with C) and the middle note below in the inner ring (Am) is the minor tonal center.
From this chunk of 6 notes you are now looking at 6/7 of the primary chords (excludes the diminished 7th chord) for both C major and A minor!
-C Major, F Major, G Major, A Minor, D Minor, E Minor
-The above chords/notes are also the same ones found D Dorian, and E Phrygian, and F Lydian, and G Mixolydian, as well! (This selections excludes the Locrian mode)



Slide the 3x2 "box" to any other note as the center point to quickly discover/remember at a glance all of the chords that belong to the minor and major keys of that root- this is just one of the many powerful secrets of the circle of fifths- but obviously the design comes at the cost of sacrificing geometric lineart aesthetics, lol

/monologue

[IntelligentAnimal-gz]

Any resource material for ancient sacred symbols drawable on this?

[kvsuprise]

As a musician myself currently studying jazz/ contemporary theory, this has opened my eyes to a whole new world. Would love to have a beer with you and look through that notebook...

[chrisbatson]

Great to see you using sacred geometry to music. I studied with Pat Martino for many years and that’s his whole approach to the theory of guitar when written out is sacred geometry, great work!

[riverofstarsastrologystudy]

can you explain why you transfered G on your C#, and A on your D#, etc...
Many thanks

[michaelscott]

Did you try and graph the golden ratio? Fib numbers divided by the previos number so 1, 2, 3/2, 5/3, 8/5, 13/8?

[GwenApMannanan]

Really wonderful video. I've played various instruments and composed for years but only recently began learning about sacred geometry and music. I can see how some people might be having a hard time understanding what you're talking about, but with a solid background in music and the flower of life I get what you're talking about and I am really thankful for the extra peek in your notebook because that gives me some great ideas for my own experimentation. So, thanks so very much for sharing your ideas! (Also, like Roby King I would be very interested in seeing a pdf of your notebook.) Really great work! =D

[oswald]

check giussepe verdi tuning in XIX century A4=432 hz C0=16 hz C1=32 C2=64 C3=128 C4=256 hz C5=512 and so on.... all exact multiple of 8 (that is why we call them octaves) :)