Total vs partial derivatives

preview_player
Показать описание
Partial derivatives involve only individual components. Total derivatives are the derivatives of an objective or constraint with respect to design variables.

0:00 - Intro
0:40 - What are partial derivatives?
1:40 - What are total derivatives?
2:44 - Totals can be computed from a mix of partials
5:32 - Conclusion

Accompanying course page:

TODO: add linked lectures as they're developed
  1. OpenMDAO
Рекомендации по теме
Difference Between Partial 0:01:44

Difference Between Partial and Total Derivative

Total vs partial 0:06:38

Total vs partial derivatives

Partial Derivatives - 1:00:33

Partial Derivatives - Multivariable Calculus

Ambiguity With Partial 0:09:39

Ambiguity With Partial ∂ Notation, and How to Resolve It

But what is 0:17:39

But what is a partial differential equation? | DE2

Introduction to the 0:02:38

Introduction to the Total Differential

Partial derivatives, introduction 0:10:56

Partial derivatives, introduction

Continuity vs Partial 0:09:11

Continuity vs Partial Derivatives vs Differentiability | My Favorite Multivariable Function

Heuristic Derivation of 0:18:34

Heuristic Derivation of Path Integrals from Classical Field Theory (including Schwinger Principle)

Full derivative vs 0:10:17

Full derivative vs partial derivative

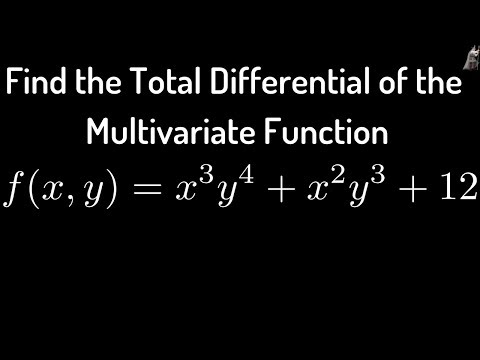
Total Differential of 0:01:59

Total Differential of Multivariate Function f(x, y) = x^3y^4 + x^2y^3 + 12

Chain Rule With 0:21:10

Chain Rule With Partial Derivatives - Multivariable Calculus

Oxford Calculus: Partial 0:18:22

Oxford Calculus: Partial Differentiation Explained with Examples

Partial Derivatives and 0:10:57

Partial Derivatives and the Gradient of a Function

Difference between partial 0:16:30

Difference between partial and total derivative | Partial derivative equations | Total derivative

How to find 0:06:15

How to find Partial Derivative of a function

Partial Derivatives and 0:47:28

Partial Derivatives and Total Differentials

The Multi-Variable Chain 0:10:47

The Multi-Variable Chain Rule: Derivatives of Compositions

Partial Derivatives: Total 0:10:39

Partial Derivatives: Total Differentials

Multivariable Calculus 5 0:11:24

Multivariable Calculus 5 | Total Derivative

Calculus 3 Lecture 2:28:53

Calculus 3 Lecture 13.3: Partial Derivatives (Derivatives of Multivariable Functions)

1. Partial Differentiation 0:08:09

1. Partial Differentiation - Small changes 1

Unit 3-Partial derivative 0:04:57

Unit 3-Partial derivative | What is the difference between partial & total derivative

Higher Order Partial 0:05:48

Higher Order Partial Derivatives

Комментарии
Автор

your intro just earned you a sub right of the bat

brickie
Автор

How would you recommend developing these partials and handing them over to OpenMDAO? Would you suggest a symbolci python package to compute them?

Equally, I have run a few loops in OpenMDAO without informing the partials at all, and it still solves the problem. Is it developing partials for me automatically?

Thanks!

AeroStark