filmov
tv
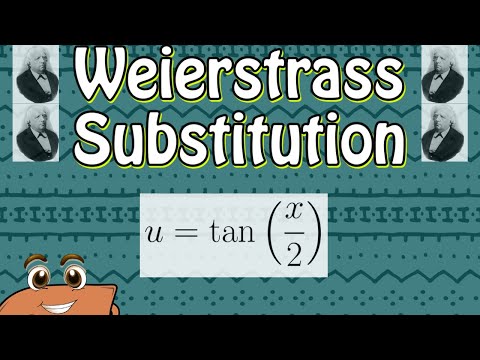
Introduction to Weierstrass Substitution
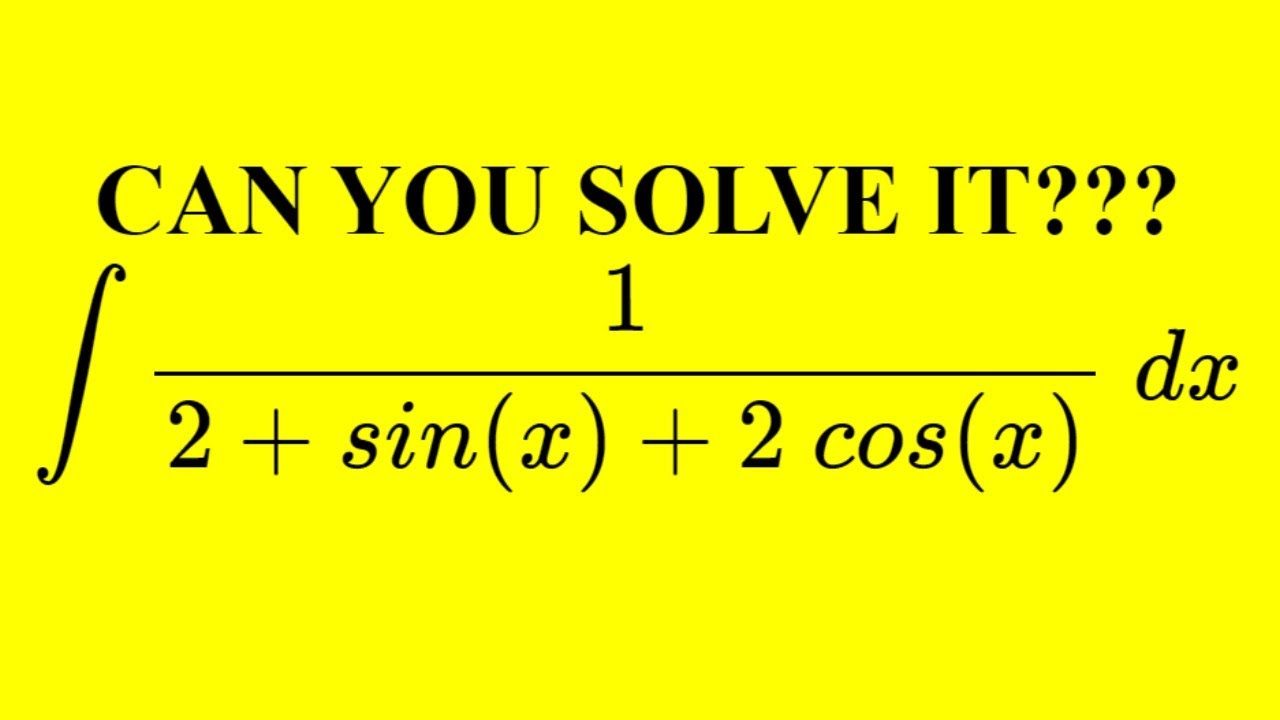
Показать описание
Practice problems
Website:
Check out my other channel OWLS SCHOOL OF MATH!
For more great math videos check out Maths Plus!
#integrationtechniques
#integrals
#mathcompetition
#weierstrass
Website:
Check out my other channel OWLS SCHOOL OF MATH!
For more great math videos check out Maths Plus!
#integrationtechniques
#integrals
#mathcompetition
#weierstrass
Introduction to Weierstrass Substitution
weierstrass substitution for integrations, intro
The Weierstrass Substitution (Introduction) | ExamSolutions
Weierstrass Substitution - Introduction (The Art of Integration)
Introduction to Weierstrass Substitution
Introduction to Weierstrass Substitution
Introduction to Weierstrass substitution and examples
Weierstrass Substitution introduction
Introduction to the Weierstrass Substitution
Integrating using t=tan(x/2) substitution - [The Weierstrass substitution]
Integrating by Weierstrass Substitution (visual proof)
(SCx23c) The Weierstrass Substitution
Weierstrass substitution for integrations : Introduction Part 1
Weierstrass Substitution and Examples
Integral of 1dx using Weierstrass Substitution (The Art of Integration)
Integrating Using Weierstrass Substitution
Weierstrass Substitution Problem 1 (The Art of Integration)
The Weierstrass Substitution (part 2) | ExamSolutions
Weierstraß substitution: strange, overpowered and very hard to pronounce
The function that solves every Integration question | Weierstrass Substitution | tan(x/2)
Integration - HOW TO: Integration Using t-Substitution (The Weierstrass Substitution)
Weierstrass Substitution for integrals #calculus #integration
integral of 1/(2+cos(x)) , Weierstrass substitution
How to use the Weierstrass Substitution to solve Trig Integrals!
Комментарии
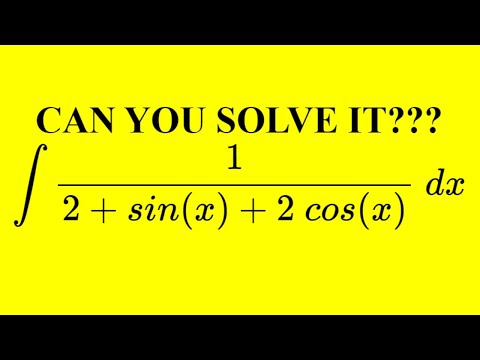 0:07:12
0:07:12
 0:07:17
0:07:17
 0:10:24
0:10:24
 0:16:22
0:16:22
 0:05:06
0:05:06
 0:08:32
0:08:32
 0:04:55
0:04:55
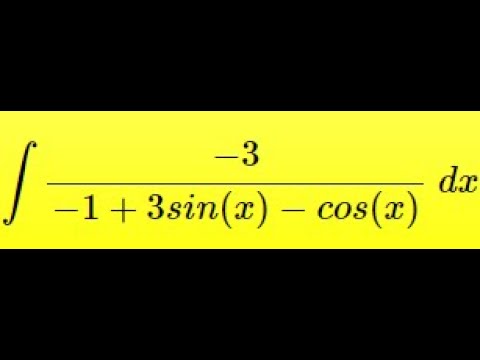 0:13:45
0:13:45
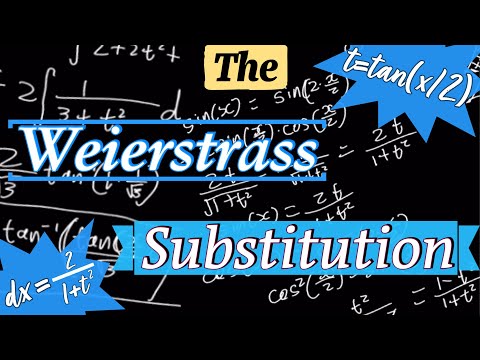 0:10:21
0:10:21
 0:13:13
0:13:13
 0:04:46
0:04:46
 0:13:36
0:13:36
 0:35:43
0:35:43
 0:23:41
0:23:41
 0:01:36
0:01:36
 0:10:05
0:10:05
 0:08:11
0:08:11
 0:06:00
0:06:00
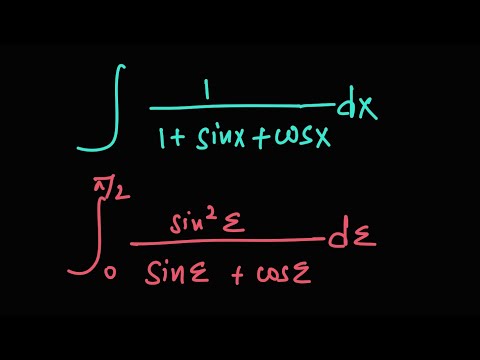 0:07:23
0:07:23
 0:06:08
0:06:08
 0:09:43
0:09:43
 0:19:11
0:19:11
 0:06:01
0:06:01
 0:10:04
0:10:04