filmov
tv
Algebra 96 Exponential Functions and Compound Interest
Показать описание
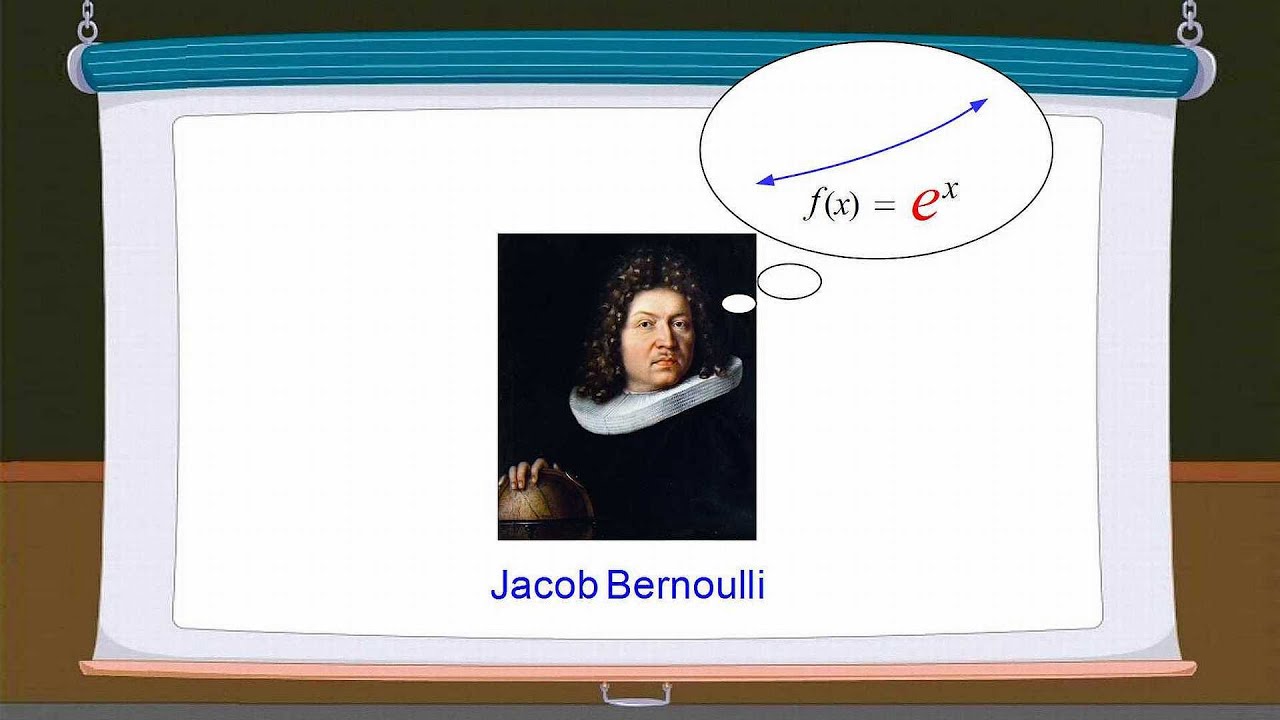
Exponential functions were first explored by the Swiss mathematician Jacob Bernoulli in sixteen-eighty-three, as a way of computing "continuous compound interest". When computing accruing interest and principal with continuous compounding, the compounding periods can be thought of as being infinitely short, with the increase in principal approaching the theoretical upper limit. In Bernoulli's quest to determine this upper limit, his research led to the development of the exponential function whose base is the constant "e", also known as "Euler's number". In this lecture, we use algebra to calculate compound interest with increasing shorter compounding periods, and show how this upper limit is approached.
Algebra 96 Exponential Functions and Compound Interest
Math 96: Exponential Functions
Math 96: Exponential Natural Base e
Math 96: Graphing Exponential Functions
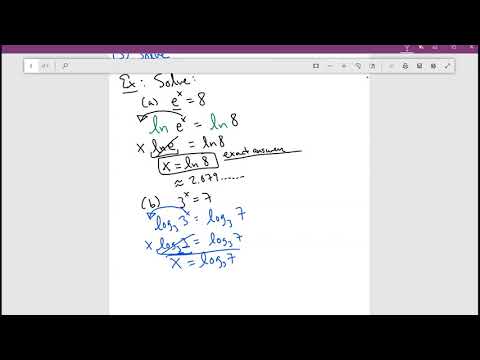
Math 96: Solving Exponential Equations
Exponential Functions
Math 96: Graphing Exponentials
Algebra I - Exponential Functions - 3Q Project
If You Can Solve This, You Are A Genius | An Impossible Exponential Problem
Math 1500 - 2.2 Exponential Functions and Models
Exponential growth and decay word problems | Algebra II | Khan Academy
QUESTION 6: FUNCTIONS (EXPONENTIAL) GRADE 11 NOV 2023
X.1 Evaluate an exponential function [D6H]
MCR3U - Create Exponential Function - Grade 11 Functions
exponential equation | #maths #mathtricks
Solving Exponential Equation
Algebra 2 Sections 8.3-8.4 Exponential Functions
Analyzing tables of exponential functions | High School Math | Khan Academy
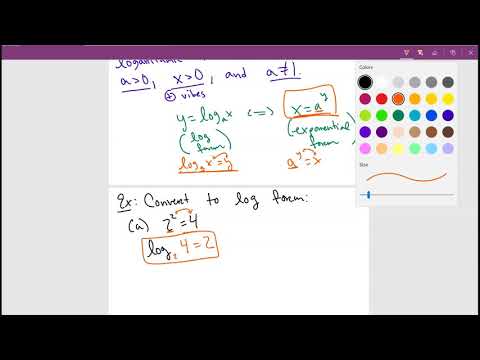
Math 96: Logarithmic Functions
free topics 96 Inverse functions defined by an exponential equation
A Nice Exponential Algebra Maths Problem @mathslearningwithsaki6636
Section 1.5 - Exponential Functions
Intensive Math (Algebra 1) - IXL Section X.1 - Evaluate an exponential function
Algebra 9-8: Geometric Sequences as Exponential Functions
Комментарии
 0:26:29
0:26:29
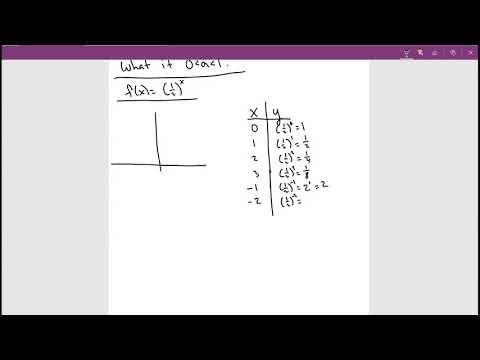 0:12:35
0:12:35
 0:11:04
0:11:04
 0:15:06
0:15:06
 0:10:37
0:10:37
 0:43:46
0:43:46
 0:09:10
0:09:10
 0:14:29
0:14:29
 0:12:02
0:12:02
 0:58:50
0:58:50
 0:07:21
0:07:21
 0:16:40
0:16:40
 0:10:34
0:10:34
 0:03:07
0:03:07
 0:00:17
0:00:17
 0:00:58
0:00:58
 0:31:13
0:31:13
 0:07:19
0:07:19
 0:09:30
0:09:30
 0:01:00
0:01:00
 0:06:13
0:06:13
 0:42:24
0:42:24
 0:11:29
0:11:29
 0:09:16
0:09:16