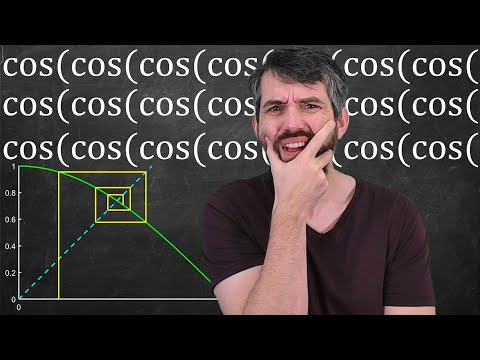
What is cos( cos( cos( cos( cos( cos( cos( cos( cos( cos( cos( cos(…?? // Banach Fixed Point Theorem

This weird expression of taking cos over and over again is just a sequence x_n=cos(x_{n-1}). There is a very cool theorem called the Banach Fixed Point Theorem that let's figure out the limit of sequences like this and depends on cos(x) being a so called contractible mapping.

0:00 The sequence
1:17 Cos(x) Graphically
3:25 Spiral Geometric View
7:02 Banach Fixed Point Theorem

COURSE PLAYLISTS:

OTHER PLAYLISTS:
► Learning Math Series
►Cool Math Series:

BECOME A MEMBER:

SOCIALS:

[DrTrefor]

🚨🚨Correction!🚨🚨
1) The proper definition of a contraction is that |f(y)-f(x)| <=k|y-x| for k in [0, 1). I was hoping to avoid explain the k, and so tried to "simplify" by just using k=1 and a strict inequality. But this isn't the same thing! That has points getting closer together, but you can construct examples where they get closer together slower and slower which means it doesn't work for the Banach fixed point theorem. So my definition is slightly too weak.
2) Apparently the solution to cos(x)=x has a name! It is called the Dottie number!
Thanks to my amazing commenters for pointing out both things, you are all the best!

[jameshenner]

0.739 radians is equivalent to 42 degrees. Therefore the Hitchhiker's Guide to the Galaxy was right in saying that 42 is the answer to everything.

[ronanshanley]

This really demonstrates just how beautiful math is. When I looked at the thumbnail I immediately thought "wow I have no idea what is it, " but in hindsight the answer of cos(x) = x is incredibly intuitive. Its amazing how something so complicated can be become so simple

[jessstuart]

Every 9th grade math student has evaluated this on their scientific calculator by pressing the cos button repeatedly.

[zygoloid]

At University I learned this in limerick form:

If A's a complete metric space,
And nonempty we know it's the case
That if F's a contraction
Then under its action
Just one point remains in its place

[mihirsanghvi]

The point x=0.739 does have a name its called Dotties number/Dotties Constant

[manpreet]

I remember noticing the convergence when I was 12-13 years old. I had bought my first scientific calculator and was so fascinated that I would try all the functions on it for hours. I would calculate repeated operations like this. I calculated cos(cos( .. 23 times)) and found that it converges, although I had no understanding of limits. BTW 23 was the stack size of the calculator operations and I could only repeat cos 23 times beyond which the calculator would report ‘stack error’

[RedPardo]

It's nice to be a software engineer, but be a math enthusiast and watch those kind of videos explaining interesting behaviors. Keep up this work, it's excelent and joyful!

[tipoima]

Really interesting.
I tried doing the sin(cos(sin(cos(...))) and it has two points it jumps between, those being x1=sin(cos(x1)) and x2=cos(sin(x2)) with them obviously x1=sin(x2) and x2=cos(x1)

[covidiotseverywhere]

Banach Fixed Point theorem is the reason for convergence in most traditional reinforcement learning algorithms and that is basically the reason for a lot of advances in robotics

[angeldude]

cos(x) definitely has one of the more interesting fixed points. I decided to check some other functions, and it seem that if the graph doesn't intersect the x=y graph, then it obviously doesn't have a fixed point (ln(x) diverges to the complex plane, and e^x diverges to infinity). sin(x) has a pretty obvious fixed point of 0, but sqrt(x) and x^2 had some interesting behavior where they both have 2 fixed points at 0 and 1, but for sqrt(x), 0 is an _unstable_ fixed point, and 1 is _stable, _ while for x^2, the relation is flipped. In addition, the fixed point for x^2 is only reached if the starting point is between -1 and 1, otherwise it diverges (away from the unstable fixed point 1) towards infinity. 1/x _neither_ diverges, _nor_ converges to a fixed point, always oscillating between two values depending on the input. (-x would have the same behavior since they're both their own inverses, though both do have fixed points in the form of 1 and -1 for 1/x, and 0 for -x.) tan(x) has _infinitely many_ fixed points, including at 0, but all of them are unstable, but not necessarily diverging to infinity when not at them, instead looking like it would have a chaotic behavior.

[JHaas]

im still trying to get my head around all of the calc sequence but it seems its true that there is always more to learn / discover. thanks for videos like these it helps to reinvigorate a desire for learning math.

[dackid]

Another thing I'd like to point out is that questions like this are classically solved with the Intermediate Value Theorem. Although Banach's fixed point theorem is nice, IVT is a much easier way to go about this one.

[cipherxen]

When I was a kid. I used to play with scientific calculator. I like to see numbers converge by pressing "cos" over and over. It was no fun in "deg" and "grad" mode though.

[francescoghizzo]

Banach fixed point theorem is also related to the Picard-Lindelof theorem and determining the existence of the solution for the cauchy problem y' = f(x, y)

[jorgelenny]

Your average high school student has gotten bored in class and decided to do cos(ans) on the calculator 1000 times to see what happens

[ngruhn]

I’m not sure if this is a valid argument but I think you could also recognize: if there are infinitely many cos

x = cos(cos(…cos(z)…))

Then removing one cos gives the same expression. Or said differently, the inner term is the same as the whole term (independent of z) so:

x = cos(x)

and we instantly arrive at the same conclusion.

[aquilazyy]

This is great. I’m learning about fixed point iteration recently in my numerical algorithm course, and I always have a hard time picturing these process in my head. The spiral graph is a really helpful perspective!

[bidish]

At first I didn't understand the spiralling but when I tinkered it with pen copy for sometime and understood it was just amazing how they boiled down that iteration to such an intuitive visual spiral.

[drmonty]

MASSIVE thank you for the calculus playlists! Great interesting video making my love for math become stronger