filmov
tv
Volume of Solids of Revolution | Cartesian & Parametric Form BY GP Sir

Показать описание
📒⏩Comment Below If This Video Helped You 💯
Like 👍 & Share With Your Classmates - ALL THE BEST 🔥
This video lecture " Volume of Solid of Revolution will help Engineering and Basic Science students to understand following topic of of Mathematics:
1. What is Volume Solids when it is revolve about X-axis & Y-axis
2. Working rule For Finding Volume of Solid of Revolution (Cartesian & Parametric Form)
3. What is volume of Sphere/Loop/Astroid When it is revolve about axis.
4. This is part of Integral Calculus
#VolumeOfSolids #Revolution #IntegralCalculus #GATE #JAM
This Concept is very important in Engineering & Basic Science Students. This video is very useful for B.Sc./B.Tech students also preparing NET, GATE and JAM Aspirants.
Find Online Engineering Math 2018 Online Solutions Of Volume of Solids of RevolutionCartesian & Parametric Form BY GP Sir Gajendra Purohit.
Do Like & Share this Video with your Friends. If you are watching for the first time then Subscribe to our Channel and stay updated for more videos around Mathematics.
Time Stamp
An introduction - 0:00
Formula of Volume of solid of Revolution - 1:13
Example 1 - 1:39
Example 2 - 3:57
Example 3 - 7:38
Example 4 - 10:08
Example 5 - 13:26
Conclusion of video - 17:21
Detailed about old videos - 17:43
📚 Our Book Publications
🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹
👤 My Social Media Handles
✅Our YouTube Channels
📚 Important Course Playlists
📚 Following Topics Are Also Available
📷 My Equipment & Gear
Thanks For Watching My Video 🙏🏻
Like, Share & Subscribe 💯
Dr.Gajendra Purohit
Like 👍 & Share With Your Classmates - ALL THE BEST 🔥
This video lecture " Volume of Solid of Revolution will help Engineering and Basic Science students to understand following topic of of Mathematics:
1. What is Volume Solids when it is revolve about X-axis & Y-axis
2. Working rule For Finding Volume of Solid of Revolution (Cartesian & Parametric Form)
3. What is volume of Sphere/Loop/Astroid When it is revolve about axis.
4. This is part of Integral Calculus
#VolumeOfSolids #Revolution #IntegralCalculus #GATE #JAM
This Concept is very important in Engineering & Basic Science Students. This video is very useful for B.Sc./B.Tech students also preparing NET, GATE and JAM Aspirants.
Find Online Engineering Math 2018 Online Solutions Of Volume of Solids of RevolutionCartesian & Parametric Form BY GP Sir Gajendra Purohit.
Do Like & Share this Video with your Friends. If you are watching for the first time then Subscribe to our Channel and stay updated for more videos around Mathematics.
Time Stamp
An introduction - 0:00
Formula of Volume of solid of Revolution - 1:13
Example 1 - 1:39
Example 2 - 3:57
Example 3 - 7:38
Example 4 - 10:08
Example 5 - 13:26
Conclusion of video - 17:21
Detailed about old videos - 17:43
📚 Our Book Publications
🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹🔸🔹
👤 My Social Media Handles
✅Our YouTube Channels
📚 Important Course Playlists
📚 Following Topics Are Also Available
📷 My Equipment & Gear
Thanks For Watching My Video 🙏🏻
Like, Share & Subscribe 💯
Dr.Gajendra Purohit
Комментарии
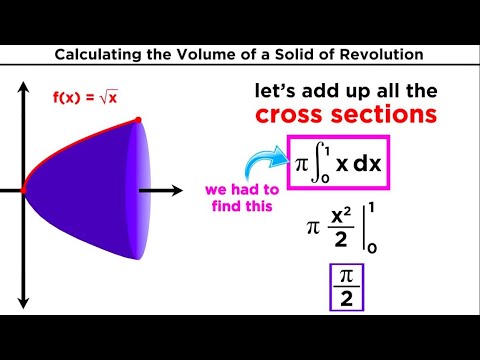 0:11:20
0:11:20
 0:20:00
0:20:00
 0:27:53
0:27:53
 2:47:49
2:47:49
 0:06:23
0:06:23
 0:12:20
0:12:20
 0:08:10
0:08:10
 0:18:32
0:18:32
 0:08:32
0:08:32
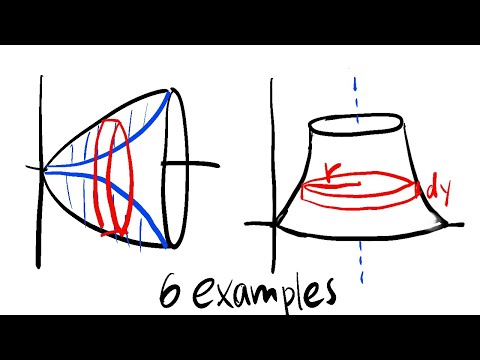 0:28:08
0:28:08
 0:35:17
0:35:17
 1:05:07
1:05:07
 0:08:10
0:08:10
 0:38:22
0:38:22
 0:08:17
0:08:17
 0:10:32
0:10:32
 0:23:07
0:23:07
 0:39:39
0:39:39
 1:44:10
1:44:10
 0:05:38
0:05:38
 0:07:40
0:07:40
 0:13:11
0:13:11
 0:18:03
0:18:03
 0:32:48
0:32:48