Where Does Math Begin? The 9 AXIOMS of Math

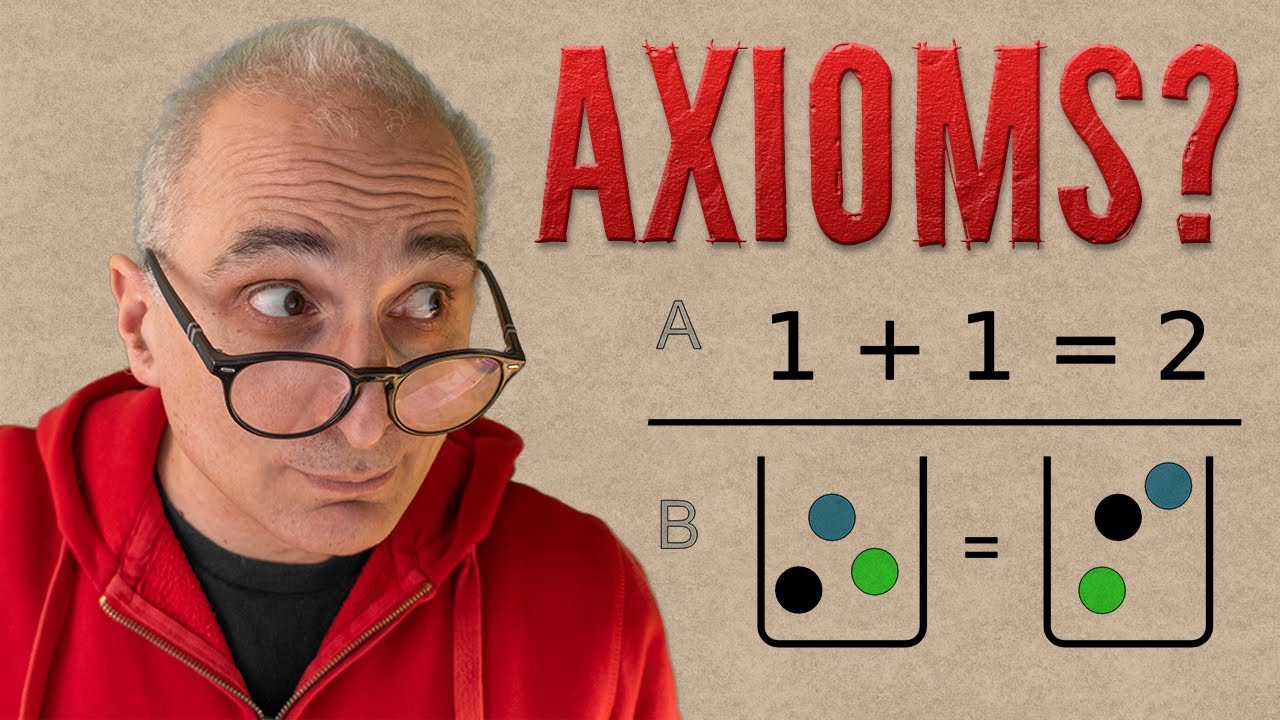
In this video we talk about 9 facts
that math does not prove and whose truth
we take for granted. These are called axioms
and everything else in math is based upon them.

New Puzzles every Sunday and Thursday.
I post math puzzles and their solutions with animations.

Videos in:

🌟My Links🌟

[brandonzx]

your videos looks like that old tv shows for children with interviews

great!

[JerichoDeGuzman-rmkd]

As a self taught. Finally I found the answers I am looking for
Muchas gracias

[hollybancroft]

This video was super engaging, thank you! The concept of axioms is so cool

[somecreeep]

Is there a standard "canonical" set of axioms you're going with for this series? I know that in most axiomatic systems, one can often interchange some of the axioms with some of the most basic theorems and get an equivalent axiomatic system, so how do you decide which basic things you're going to call axioms in this series? For example, I notice you have the axiom of choice as axiom #9, but as I understand it, you could have chosen Zorn's lemma instead for that one.

[choutycoh]

axiom is what we can't do any better

[emmanuelnwafor]

Awesome video. What's the name of the city @3:04? I absolutely adore the buildings behind those people.

[angeldude]

It's possible to prove that 1 + 1 = 2 from simpler axioms, but is it possible to do the same for successor(1) = 2?

[GSandSDS]

Why is there a second "if and only if" in the first axiom? Wouldn't an "and" be enough? C has to be in A "and" also be in B for every C. Btw. since all nine axioms listet in the video are actually the axioms of ZFC set theory, it would be interesting to hear why this perticular set theory is so special that it can be used for math as a hole. There are for example other set theories that for example have no axiom of foundation.

[dominiqueubersfeld]

Don't forget Terrence Howard's axiom that states : 1x1 = 2

[markwrede]

But is congruence identical to equality?

[oversquare]

uh no. The first axiom of math is that contradiction is forbidden. It is in fact the only axiom of math, but you could argue that it practically requires a second axiom where-in definitions must have precise agreement before evaluation. This video is a testament to how easily a person can confuse themselves when they don't understand these axioms.

They are so blatantly taken for granted that this video goes straight to the idea of a proof without noticing that the definition of a proof itself relies first on excluding contradiction. The example given to random people of balls in a jar exploit the 2nd axiom - that definitions must be agreed to precisely in advance. The presenter merely asserts without agreement that the position of the balls for example is irrelevant - that is not true in every case. As a result, the entire video is an exercise in absurdity.

However, once the presenter decides to describe the balls as a mathematical set, through social contract one can reasonably argue the definition has been ascribed to exclude position as irrelevant. It is incorrect to try to force it or assume it however and that is a dramatically bad blunder.

Importantly, math is a symbolic abstraction just like any other language. Therefore the first example 1+1=2 has already been rendered into the proper symbology and the balls have not; they invoke a physical reality and certainly have not been defined into a widely known symbolic abstraction fit for a true/false evaluation. Finally though, no - set theory is obviously not the foundation of math neither are its axioms. The axioms of set theory are just the axioms of set theory. The axioms of category theory are just the axioms of category theory. If you want to argue either is "the foundation of math" you would be on equal footing with either set theory or category theory. The resulting contradiction necessarily excludes both as not the foundation of math.

[bookofproofs]

Is this a footage of a ghost 😮behind GuzMat at 2:04?

[mathslover]

1st is Axiom of Extension. Halmos, Naive Set Theory.

[sabotagedgamerz]

Mate I get what you’re trying to say but I gotta slightly disagree with you. The axiom you name is this video is more of a definition of what it means for 2 sets to be equal. Also, the jar thing, we have no idea whether the elements of a jar are ordered or have specific coordinates, so the equality symbol there needs extra definition.

But I appreciate the attempt to teach rigorous logic to the world.

[waelelsherif]

It is really weird that there are mathematicians do not believe in the existance of the creator !

[JustAThought]

**ATTN: 😌😉This comment is equal to a rant from a novice mathematician who desperately wants and LONGS to know and understand mathematics.😔😉😁**

Question: if math and the basic foundations for math are so hard for people to comprehend—as your video has displayed—could it be the mathematical: i.e., theorems and axioms; system being taught is flawed???

Honestly, using logic, it is a known (“proven”) fact that a vast majority of people, globally, have issues trying to understand mathematics as they have been taught.

Therefore, could it be the commonly used Greek adapted mathematical, now called the Algebraic system, is grossly flawed???

How many more centuries must mankind endure this dated, and, in my opinion, flawed and grossly hard to comprehend Greek philosophical interpreted version of ancient (Egyptian and Babylonian) “earth measurements, ” which is now commonly known as the globally accepted Grecian term, geometry (Merriam-Webster: Geometry- Etymology…. from Greek geōmetria, from geōmetrein to measure the earth)?🤔🧮 +🧐📚👨‍💻=😁🧑🏻‍🎓!

[Dawid-knmv]

Way to long video for so little content. You could fit all axioms in one video instead inconvieniently dividing one simple topic on several videos.

[sumdumbmick]

1+1=2 is axiomatic per Peano's Axioms. the alleged proof which appears in Principia Mathematica is a circular application of this, and thus is unsound.

if 1+1=2 then there would be no need for algebra, and unit conversions would be impossible. consider, for instance:
1 foot + 1 yard = 48 inches
1 dog + 1 quail = 6 legs
1C water + 1C dirt = some mud
1 frog + 1 pond = 1 pond

you can thus soundly prove that a pair of vectors with comensurable units and magnitudes of 1 sum to one vector in the same unit with a magnitude of 2. but the number 1 cannot actually even be added to anything, and attempting to do so won't yield a number, let alone the number 2.

[djantebe]

Such a boring and repetitive narration. Entire video could be well condensed, and I cringed hard from the public polling

[freddielittle]

Binary code 0110111001(0-9) starts over again don't pass (10)