filmov
tv
Amos Nevo: Representation theory, effective ergodic theorems, and applications - Lecture 1
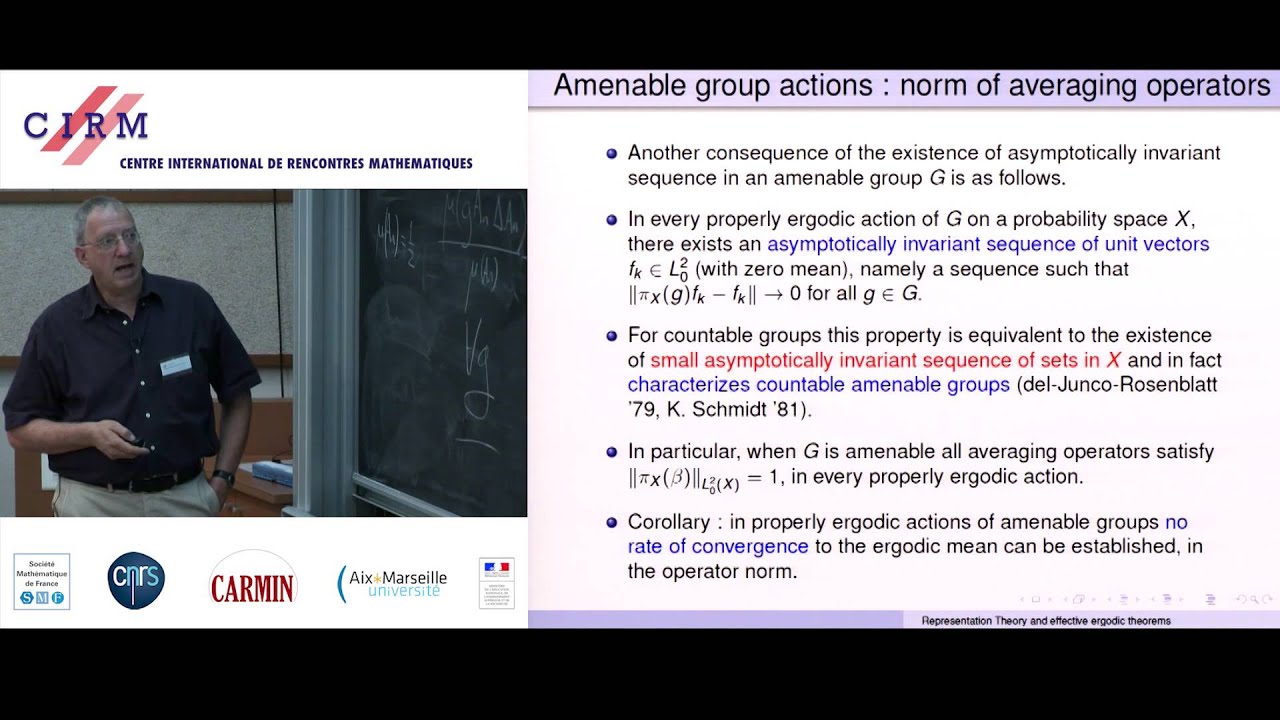
Показать описание
- Chapter markers and keywords to watch the parts of your choice in the video
- Videos enriched with abstracts, bibliographies, Mathematics Subject Classification
- Multi-criteria search by author, title, tags, mathematical area
Our first purpose is to show how aspects of the representation theory of (non-amenable) algebraic groups can be utilized to derive effective ergodic theorems for their actions. Our second purpose is to demonstrate some the many interesting applications that ergodic theorems with a rate of convergence have in a variety of problems. We will start by a discussion of property T and show how to extend the spectral estimates it provides considerably beyond their usual formulations. We will also show how to derive best possible spectral estimates via representation theory in some cases. In turn, such spectral estimates will be used to derive effective ergodic theorems. Finally we will show how the rate of convergence in the ergodic theorem implies effective solutions in a host of natural problems, including the non-Euclidean lattice point counting problem, fast equidistribution of lattice orbits on homogenous spaces, and best possible exponents of Diophantine approximation on homogeneous algebraic varieties.
Recording during the thematic meeting: "Group representations in dynamical systems and geometry" the June 29, 2015 at the Centre International de Rencontres Mathématiques (Marseille, France)
Filmmaker: Guillaume Hennenfent
- Videos enriched with abstracts, bibliographies, Mathematics Subject Classification
- Multi-criteria search by author, title, tags, mathematical area
Our first purpose is to show how aspects of the representation theory of (non-amenable) algebraic groups can be utilized to derive effective ergodic theorems for their actions. Our second purpose is to demonstrate some the many interesting applications that ergodic theorems with a rate of convergence have in a variety of problems. We will start by a discussion of property T and show how to extend the spectral estimates it provides considerably beyond their usual formulations. We will also show how to derive best possible spectral estimates via representation theory in some cases. In turn, such spectral estimates will be used to derive effective ergodic theorems. Finally we will show how the rate of convergence in the ergodic theorem implies effective solutions in a host of natural problems, including the non-Euclidean lattice point counting problem, fast equidistribution of lattice orbits on homogenous spaces, and best possible exponents of Diophantine approximation on homogeneous algebraic varieties.
Recording during the thematic meeting: "Group representations in dynamical systems and geometry" the June 29, 2015 at the Centre International de Rencontres Mathématiques (Marseille, France)
Filmmaker: Guillaume Hennenfent
 0:54:21
0:54:21
 1:06:44
1:06:44
 1:02:52
1:02:52
 1:00:17
1:00:17
 1:04:11
1:04:11
 0:53:51
0:53:51
 0:57:41
0:57:41
 1:04:51
1:04:51
 0:59:13
0:59:13
 0:56:40
0:56:40
 0:55:49
0:55:49
 0:15:00
0:15:00
 0:10:16
0:10:16
 0:59:29
0:59:29
 0:47:41
0:47:41
 0:48:02
0:48:02
 0:55:10
0:55:10
 0:53:03
0:53:03
 0:43:34
0:43:34
 0:53:53
0:53:53
 0:31:16
0:31:16
 1:04:39
1:04:39
 0:10:00
0:10:00
 1:31:45
1:31:45