filmov
tv
Hamilton-Jacobi Theory: Finding the Best Canonical Transformation + Examples | Lecture 9
Показать описание
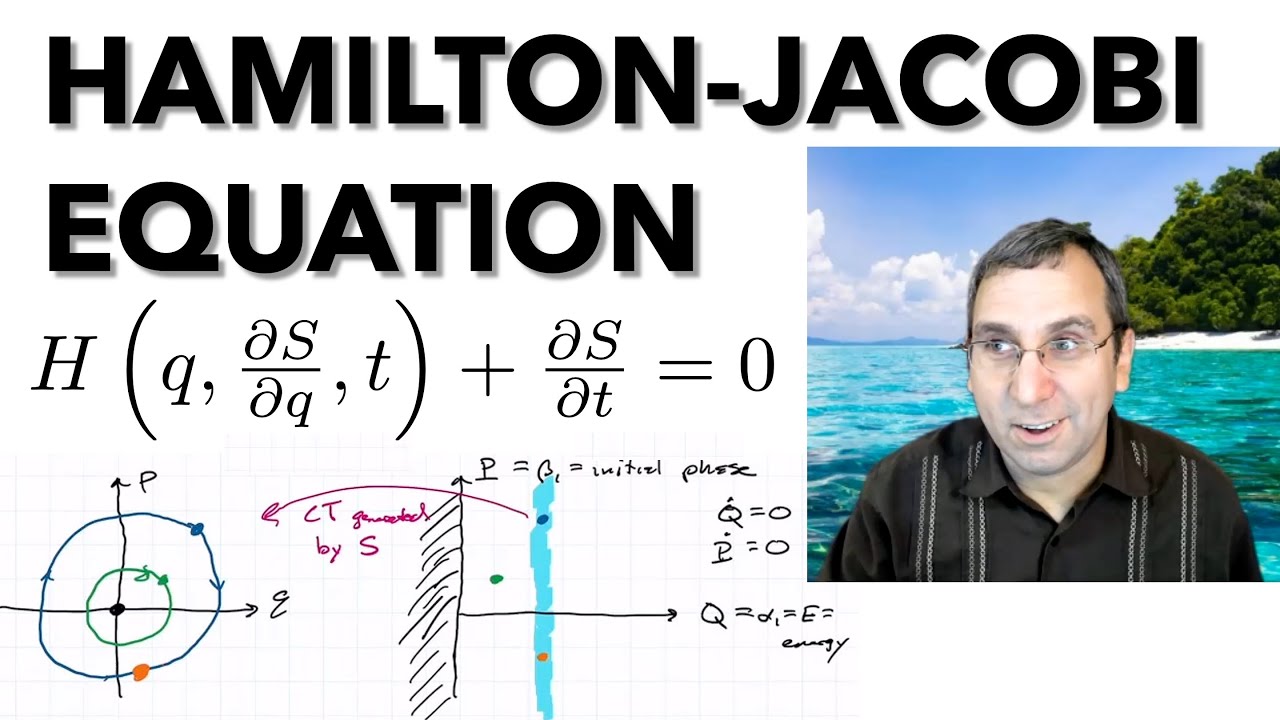
Lecture 9, course on Hamiltonian and nonlinear dynamics. Hamilton-Jacobi theory for finding the best canonical transformation to solve the Hamilton's equations. Hamilton-Jacobi equation, a partial differential equation for the Hamilton's principal function S with n+1 variables and n parameters. Common simplification if original H is independent of time, solving for W, Hamilton's characteristic function. Geometric interpretation: solutions in original coordinates map to equilibria in the Hamilton-Jacobi coordinates. S is also the action integral. Examples: simple harmonic oscillator and central force problem, e.g., Kepler's 2-body problem.
► Next: Action-Angle Variables in Hamiltonian Systems | Visualizing Tori and Spheres in N Dimensions
► Previous, Hamiltonian Flow is a Canonical Transformation | Strange Non-Intuitive Momenta
► Dr. Shane Ross, Virginia Tech professor (Caltech PhD)
► New lectures posted regularly
► Follow me on Twitter
► Courses and Playlists by Dr. Ross
📚Attitude Dynamics and Control
📚Nonlinear Dynamics and Chaos
📚Hamiltonian Dynamics
📚Lagrangian and 3D Rigid Body Dynamics
📚Center Manifolds, Normal Forms, and Bifurcations
► Chapters
0:00 Hamilton-Jacobi theory introduction
4:18 Every point in phase space is an equilibrium point
5:51 Derivation of Hamilton-Jacobi equation
13:06 Example: Hamilton-Jacobi for simple harmonic oscillator
31:00 Simplification: if Hamiltonian is time-independent
34:23 Hamilton's Principal function S is the action integral
39:00 Example: Hamilton-Jacobi for Kepler problem
50:07 Simplification: if Hamiltonian is separable
► Class notes in PDF form
► in OneNote form
► See the entire playlist for this online course:
Advanced Dynamics - Hamiltonian Systems and Nonlinear Dynamics
This course gives the student advanced theoretical and semi-analytical tools for analysis of dynamical systems, particularly mechanical systems (e.g., particles, rigid bodies, continuum systems). We discuss methods for writing equations of motion and the mathematical structure they represent at a more sophisticated level than previous engineering dynamics courses. We consider the sets of possible motion of mechanical systems (trajectories in phase space), which leads to topics of Hamiltonian systems (canonical and non-canonical), nonlinear dynamics, periodic & quasi-periodic orbits, driven nonlinear oscillators, resonance, stability / instability, invariant manifolds, energy surfaces, chaos, Poisson brackets, basins of attraction, etc.
► Background on Lagrangian systems, which have their own lecture series, 'Analytical Dynamics'
► Continuation of this course on a related topic
Center manifolds, normal forms, and bifurcations
► A simple introductory course on Nonlinear Dynamics and Chaos
► References
The class will largely be based on the instructor’s notes.
In addition, references are:
A Student’s Guide to Lagrangians and Hamiltonians by Hamill
Analytical Dynamics by Hand & Finch
Classical Dynamics: A Contemporary Approach by José & Saletan
Classical Mechanics, 3rd Edition by Goldstein, Poole, & Safko
Lecture 2021-07-15
action angle cyclic variables in classical mechanics statistical physics quasiperiodic online course Hamilton Hamilton-Jacobi theory three-body problem orbital mechanics Symplectic Geometry topology
#Hamiltonian #HamiltonJacobi #CanonicalTransformation #HamiltonJacobiEquation #Momentum #GeneratingFunction #EulerLagrange #PrincipleLeastAction #LeastAction #Brachistochrone #HamiltonsPrinciple #CanonicalTransformation #CyclicCoordinates #DynamicalSystems #IgnorableCoordinate #SymplecticGeometry #IntegralInvariant #PoincareCartan #CyclicVariable #Uncertainty #Legendre #NonlinearDynamics #DynamicalSystems #Lagrangian #mathematics #Dynamics #ChaoticDynamics #Canonical #Poisson #OptimalControl #Poincare #Lagrange #Hamilton #HamiltonJacobi #ThreeBody #PoincareMap #chaos #Lyapunov #Oscillators #NonlinearOscillators #Oscillations #VectorFields #topology #geometry #EnergyConservation #SkewGradient #FixedPoint #DifferentialEquations #DifferentialEquations #PhaseSpace #EquilibriumPoints #LinearStabilityAnalysis #StabilityAnalysis #VectorField #TwoDimensional #Functions #GradientSystem #GradientVectorField #Cylinder #Pendulum #Newton #LawOfMotion #dynamics #mathematicians #maths #mathstudents #mathematician #mathfacts #mathskills #mathtricks #KAMtori #Arnold
► Next: Action-Angle Variables in Hamiltonian Systems | Visualizing Tori and Spheres in N Dimensions
► Previous, Hamiltonian Flow is a Canonical Transformation | Strange Non-Intuitive Momenta
► Dr. Shane Ross, Virginia Tech professor (Caltech PhD)
► New lectures posted regularly
► Follow me on Twitter
► Courses and Playlists by Dr. Ross
📚Attitude Dynamics and Control
📚Nonlinear Dynamics and Chaos
📚Hamiltonian Dynamics
📚Lagrangian and 3D Rigid Body Dynamics
📚Center Manifolds, Normal Forms, and Bifurcations
► Chapters
0:00 Hamilton-Jacobi theory introduction
4:18 Every point in phase space is an equilibrium point
5:51 Derivation of Hamilton-Jacobi equation
13:06 Example: Hamilton-Jacobi for simple harmonic oscillator
31:00 Simplification: if Hamiltonian is time-independent
34:23 Hamilton's Principal function S is the action integral
39:00 Example: Hamilton-Jacobi for Kepler problem
50:07 Simplification: if Hamiltonian is separable
► Class notes in PDF form
► in OneNote form
► See the entire playlist for this online course:
Advanced Dynamics - Hamiltonian Systems and Nonlinear Dynamics
This course gives the student advanced theoretical and semi-analytical tools for analysis of dynamical systems, particularly mechanical systems (e.g., particles, rigid bodies, continuum systems). We discuss methods for writing equations of motion and the mathematical structure they represent at a more sophisticated level than previous engineering dynamics courses. We consider the sets of possible motion of mechanical systems (trajectories in phase space), which leads to topics of Hamiltonian systems (canonical and non-canonical), nonlinear dynamics, periodic & quasi-periodic orbits, driven nonlinear oscillators, resonance, stability / instability, invariant manifolds, energy surfaces, chaos, Poisson brackets, basins of attraction, etc.
► Background on Lagrangian systems, which have their own lecture series, 'Analytical Dynamics'
► Continuation of this course on a related topic
Center manifolds, normal forms, and bifurcations
► A simple introductory course on Nonlinear Dynamics and Chaos
► References
The class will largely be based on the instructor’s notes.
In addition, references are:
A Student’s Guide to Lagrangians and Hamiltonians by Hamill
Analytical Dynamics by Hand & Finch
Classical Dynamics: A Contemporary Approach by José & Saletan
Classical Mechanics, 3rd Edition by Goldstein, Poole, & Safko
Lecture 2021-07-15
action angle cyclic variables in classical mechanics statistical physics quasiperiodic online course Hamilton Hamilton-Jacobi theory three-body problem orbital mechanics Symplectic Geometry topology
#Hamiltonian #HamiltonJacobi #CanonicalTransformation #HamiltonJacobiEquation #Momentum #GeneratingFunction #EulerLagrange #PrincipleLeastAction #LeastAction #Brachistochrone #HamiltonsPrinciple #CanonicalTransformation #CyclicCoordinates #DynamicalSystems #IgnorableCoordinate #SymplecticGeometry #IntegralInvariant #PoincareCartan #CyclicVariable #Uncertainty #Legendre #NonlinearDynamics #DynamicalSystems #Lagrangian #mathematics #Dynamics #ChaoticDynamics #Canonical #Poisson #OptimalControl #Poincare #Lagrange #Hamilton #HamiltonJacobi #ThreeBody #PoincareMap #chaos #Lyapunov #Oscillators #NonlinearOscillators #Oscillations #VectorFields #topology #geometry #EnergyConservation #SkewGradient #FixedPoint #DifferentialEquations #DifferentialEquations #PhaseSpace #EquilibriumPoints #LinearStabilityAnalysis #StabilityAnalysis #VectorField #TwoDimensional #Functions #GradientSystem #GradientVectorField #Cylinder #Pendulum #Newton #LawOfMotion #dynamics #mathematicians #maths #mathstudents #mathematician #mathfacts #mathskills #mathtricks #KAMtori #Arnold
Комментарии
 0:53:51
0:53:51
 0:18:24
0:18:24
 0:57:31
0:57:31
 0:50:11
0:50:11
 0:17:39
0:17:39
 0:52:04
0:52:04
 0:07:14
0:07:14
 1:02:33
1:02:33
 0:54:39
0:54:39
 1:35:30
1:35:30
 0:48:46
0:48:46
 1:01:47
1:01:47
 0:14:43
0:14:43
 1:20:57
1:20:57
 0:49:10
0:49:10
 0:46:23
0:46:23
 0:44:35
0:44:35
 0:54:02
0:54:02
 0:01:08
0:01:08
 0:45:40
0:45:40
 0:18:33
0:18:33
 0:50:36
0:50:36
 0:58:12
0:58:12
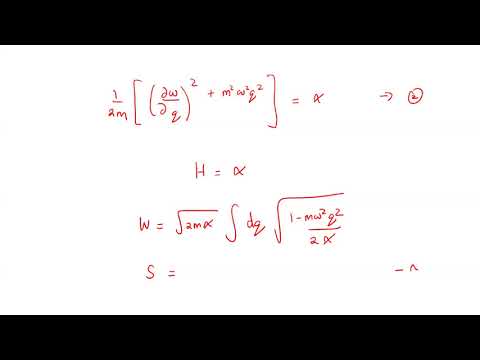 0:13:49
0:13:49