filmov
tv
Group theory 17: Finite abelian groups

Показать описание
This lecture is part of a mathematics course on group theory. It shows that every finitely generated abelian group is a sum of cyclic groups.
Correction: At 9:22 the generators should be g, h+ng not g, g+nh
Correction: At 9:22 the generators should be g, h+ng not g, g+nh
Group theory 17: Finite abelian groups
Group Theory-9 || Problems of Finite Abelian Group (Part-1)
Abstract Algebra II: fundamental theorem of finitely generated Abelian groups example, 4-5-17
Lec -17 Finite Abelian Groups with Klein four Group | IIT JAM | CSIR UGC NET | GATE MA | B Sc
Fundamental Theorem of Finitely Generated Abelian Groups -- Abstract Algebra Examples 16
Fundamental Theorem of Abelian Groups -- Abstract Algebra 16
Group Theory: Finite abelian groups
Modern Algebra Module 17 Fundamental Theorem of Finite Abelian Groups
Fundamental Theorem of Finite Abelian Groups
Group Theory: Finite abelian groups
The Fundamental Theorem of Finite Abelian Groups | Modern Algebra I
Finite Abelian Groups
Classify All Abelian Groups of Order 216
Cauchy's Theorem for Finite Abelian Groups
Lec MCQ 17 SUBGROUP, ABELIAN GROUP, cyclic group, properties of finite abelian groups #csir_net
Finite Abelian Groups: Classification step 2
Visual Group Theory, Lecture 4.4: Finitely generated abelian groups
Group Theory 48, Fundamental Theorem of Finite Abelian Groups
Fundamental Theorem of finite abelian Groups Statement and Example
GT22. The Fundamental Theorem of Finite Abelian Groups
Abstract Algebra 64: Fundamental theorem of finite abelian groups, Part I
Abstract Algebra 11.1: Fundamental Theorem of Finite Abelian Groups
Abstract Algebra 65: Fundamental theorem of finite abelian groups, Part II
Collin Roberts - The cohomology ring of a finite abelian group
Комментарии
 0:25:42
0:25:42
 0:19:22
0:19:22
 0:53:17
0:53:17
 0:13:28
0:13:28
 0:15:48
0:15:48
 0:59:02
0:59:02
 0:30:31
0:30:31
 0:23:35
0:23:35
 0:06:56
0:06:56
 0:47:46
0:47:46
 1:42:07
1:42:07
 0:07:57
0:07:57
 0:08:04
0:08:04
 0:06:49
0:06:49
 0:19:31
0:19:31
 0:19:59
0:19:59
 0:24:47
0:24:47
 0:18:49
0:18:49
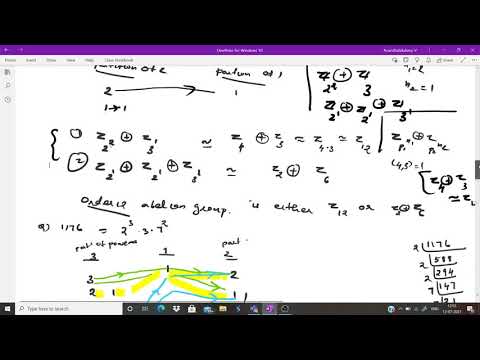 0:12:15
0:12:15
 0:08:57
0:08:57
 0:07:23
0:07:23
 0:06:44
0:06:44
 0:09:14
0:09:14
 0:03:03
0:03:03