GT22. The Fundamental Theorem of Finite Abelian Groups

Показать описание

Edit for 5:45: Proof of FTFAG needs more steps as follows (thanks to Jack Shotton for the example in the comments):

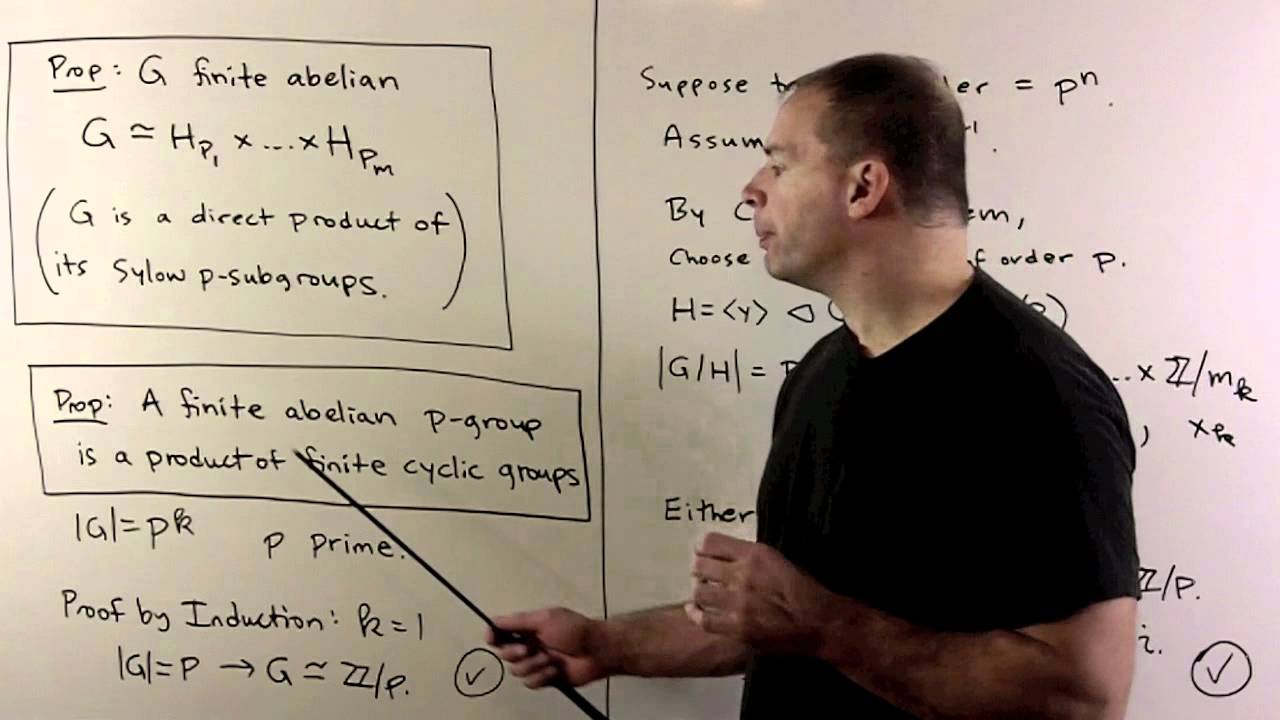
Case 3: First note, if some [xi] does not contain y, then it maps isomorphically to Z/mi in G/H. One can then show that G= [xi] x Gi, where Gi is generated by the other xi's. Then the induction step finishes the proof.

Since [z] does not contain y, using the first note, this completes the general induction proof.

Abstract Algebra: We state and prove the Fundamental Theorem of Finite Abelian Groups. We apply internal direct products to Sylow subgroups in this case. Steps include showing the result for finite abelian p-groups and using the combination rule for cyclic groups with relatively prime order.

Рекомендации по теме

Комментарии

@MathDoctorBob I'm not sure that this proof (of FTFAG) is quite right. Specifically, case 2 in the bottom right of the board at 5:45. If G = Z/p^2 x Z/p, H = <(p, 0)>, x_1 = (1, 1), x_2 = (1, 2), then x_1, x_2 is a basis for G/H, but H is contained in the subgroup generated by x_1, and that generated by x_2.

cadgenottosh

I'm a big fan! Your proof is way quicker than others!

shenanigwyns

Your welcome! My version of Uh/Um is either Now or So. It's more noticeable on the early videos, but mostly trained out by now.

MathDoctorBob

Excellent stuff. Thanks for the video.

tkcannon

Bob, your lectures are truly amazing! I have noticed that you never even say "Um.." or "Uh..". Very well executed! Thank you. Looking forward to watching all of your group theory lectures (I found this particular one while searching for explanation of Fundamental Theorem of Finite Abelian Groups).

ShamLikesCandle

Great lectures. Keep up the good work!

spramodh

In this case, the product has only one factor - Z/4. You are correct: Z/2 x Z/2 and Z/4 are not isomorphic since the first group has no element of order 4.

Here's the next thing to worry about: Z/2 x Z/3 is isomorphic to Z/6 (and then there is only one abelian group of order 6). We can combine when the factors are relatively prime.

MathDoctorBob

Hi dr., how r u ? I have the following question : show that the invariant factors of Zm + Zn are the greatest common divisors and the least common multiple, if (m, n) is greater than one and mn if (m, n) = 1 . I appreciate u r help alot .

memequbbaj

GT22. The Fundamental Theorem of Finite Abelian Groups

GT22. The Fundamental Theorem of Finite Abelian Groups

Fundamental Theorem of Abelian Groups

Fundamental Theorem of Finite Abelian Groups

Abstract Algebra - 11.1 Fundamental Theorem of Finite Abelian Groups

302.S13B: Fundamental Theorem of Algebra -- Proof

Abst Alg 22: First Isomorphism Theorem, Fundamental Theorem of Finite Abelian Groups, Sylow Theorems

The Fundamental Theorem of Finite Abelian Groups

Fundamental Theorem of Finite Abelian Groups, Proof 1

Fundamental Theorem of Finite Abelian Groups, Proof 4

Abstract Algebra Subgroups Fundamental Theorem of Cyclic Groups

Group Theory 48, Fundamental Theorem of Finite Abelian Groups

Fundamental Theorem of Finitely Generated Abelian Groups -- Abstract Algebra Examples 16

Fundamental Theorem of Abelian Groups -- Abstract Algebra 16

Abstract Algebra 64: Fundamental theorem of finite abelian groups, Part I

Fundamental Theorem of Finite Abelian Groups, Proof 2

301.11D The Fundamental Theorem of Finite Abelian Groups

Fundamental Theorem of Finite Abelian Groups, Proof 3

Abstract Algebra 11.1: Fundamental Theorem of Finite Abelian Groups

ZPEM3301 - Fundamental Theorem of Finite Abelian Groups

M-04. The fundamental theorem of finite abelian groups: existence

Proof of the Fundamental Theorem of Finite Abelian Groups (Algebra 1: Lecture 20 Video 3)

Abstract Algebra 4.5: Fundamental Theorem of Cyclic Groups

301.11A Fundamental Theorem of Finite Abelian Groups - Goals

Abstr Alg: Finite Group Theory Classification Theorems, Fundamental Theorem of Finite Abelian Groups