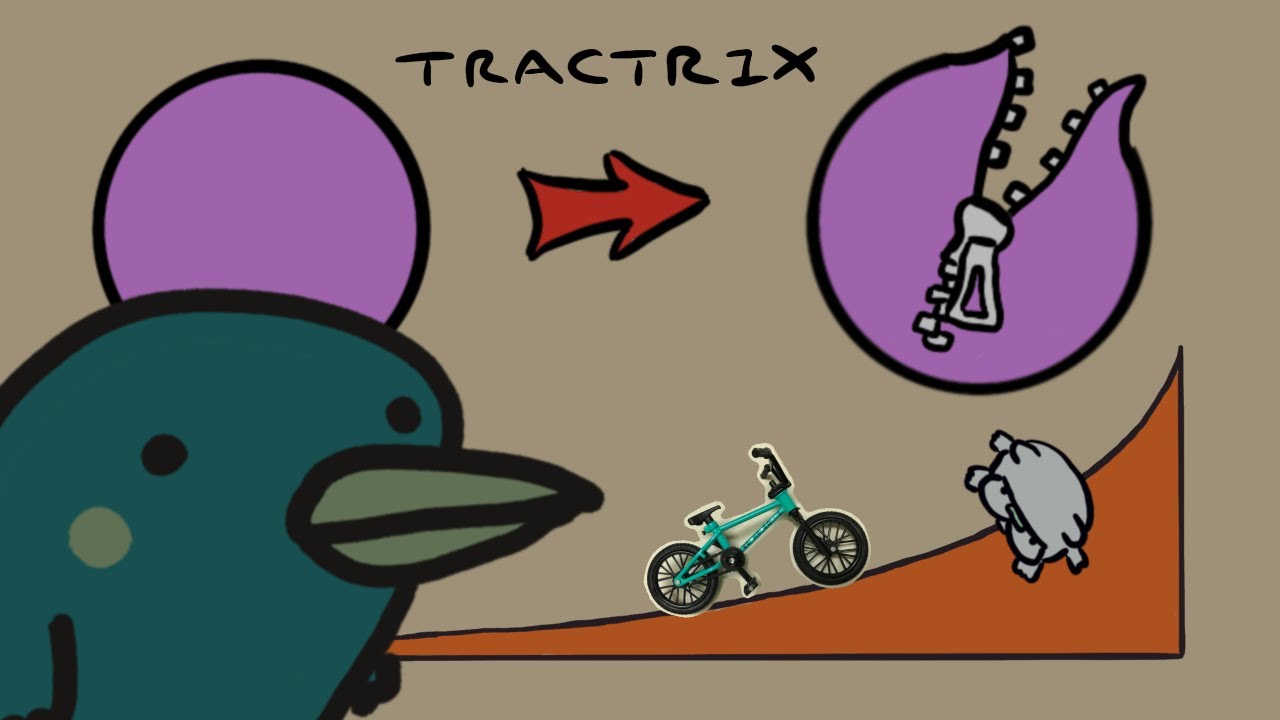
How to turn a circle inside out (Visual Calculus and the Tractrix)

The shape that a dog walks in when pulled by its owner shows up in more places than you might think. This video explores a curve called the tractrix and introduces visual calculus, a method for calculating integrals without any calculations.

0:00 Dog on a leash, intro
1:23 Deriving the tractrix
3:17 Visual calculus
6:01 Area between bike tracks
8:04 Conclusion

Gaussian curvature of the pseudosphere: Manfredo P. do Carmo, "Differential Geometry of Curves and Surfaces", p.168-169

[lexinwonderland]

your presentation is absolutely adorable, your explanation is satisfying but accessible, please please please keep making content!!

[not_David]

I'm floored by how good your videos are, please keep making more!

[yumnuska]

I’m just flabbergasted at how good your videos are. Please keep going.

First, your casual style really minimizes completely in a beautiful way. It’s just so simple!! Meaning that you introduce complexity in such a natural way that it doesn’t feel complex at all.

Second, and following from the first, I just feel so happily, jubilantly surprised when you use a simple example to describe a complex result. The bike path metaphor leading to 2pi just shocked me in the best way. I love it.

As a casual math geek, I just love how you present complex ideas without getting too deep in the details. I don’t care about the details, I care about the joy of the relationships, and you do that just so wonderfully.

[iamstickfigure]

The factoid about area traced out while riding a bike is delightful. And your illustrations to support the idea were perfect! It really made it click for me, and I think the idea will stick with me every time I ride a bike

[cougar]

I have a physics PhD and I never heard of the tractrix. I’m glad you made this video because I learned something. Very well done and please keep it up!

[josephblattert]

This channel better blow up soon. You deserve to be so much bigger with this kind of quality.

[katarixy]

This was beautifully done. As a math teacher myself I cannot help but thank you for the brilliant perspective and detailed presentation.

[andrewpappas]

Well done. I was enthralled through the entire presentation. Please, continue to make videos.

[AJMansfield]

That trick with the area between a bicycle's tracks is also (with some coordinate substitutions) the way a planimeter works.

[sulfurx]

I didn’t realise this channel only had 9k subs until a couple videos in. With this level of quality you’ll definitely have a bunch more in no time.

[drkirka]

I'm in SHOCK that you don't have as much subscribers as i thought you would. Your visuals, audio, script and overall production quality are incredible. Keep up the work!!!! Love it. 💕

[jojoanggono]

I love Calculus. Dog will be infinitely close to owner path but never touches. Your visual method of explaining is very appealing, I imagine it would be interesting for young audience.

[micahsilverman]

You are my new favorite YouTube channel! I feel lucky that YouTube reccomended you!

[flavioryu]

I got a couple of recent videos recommended and now im in a deepdive of all the other videos. I love this channel

[fable]

Love your videos, so entertaining and still super informative.
Hopefully you get a bit more attention in the future!

[congruentcrib]

The whole analogy with the bike is actually used in safety measures in cars. We always suggest you have your best tires in the back; even if you have a front wheel drive car.
The reason is if you hydroplane in the read, but not the front, the rear tires new angle (total toe/ thrust angle) will dictate where you go. On the other hand if your front tires hydroplane your steer ahead will change and this is much easier to recover. Along with that, if you step on the brakes and your rear tires hydroplane, your rear end will be pushed forward making your thrust angle exponentially greater. If your front tires hydroplane and you brake, the front end will pull on the vehicle which will not put you into a uncontrolled drift of over 45 degrees. A 45 degree spin out is much easier than a 360 spin out. These both are extremes, but we’ve seen you guys drive… I’m amazed anyone is alive to be honest.
If you want more details, I’d love to share them. I’m alignment certified by Hunter Engineering, and that’s why I may use fancy terms like “total toe, steer ahead, thrust angle, etc.” and if you need me to explain, I’m happy to. I just will say that it’s easier to show than it is to tell. After all, it is geometry… and physics. Stuff is hard to explain with visuals, but is much harder using only words.

[bhavin_ch]

Isn't this an Irodov problem? This feels like an Irodov problem! This is like bringing back some massive nostalgia from my college entrance prep days ❤

[mcloudyoutube]

I’ve been slowly going through your videos this past week, and I absolutely love all of them. Please keep up your content, I love how you focus on explaining through the theoretical/contextual lens rather than force feeding all of the practical information and hard numbers

[nenben]

When you first posed the question, I imagined the dog making a perfect circular orbit around the owner

[derpaderp]

Top tier content. This is genuinely enjoyable.