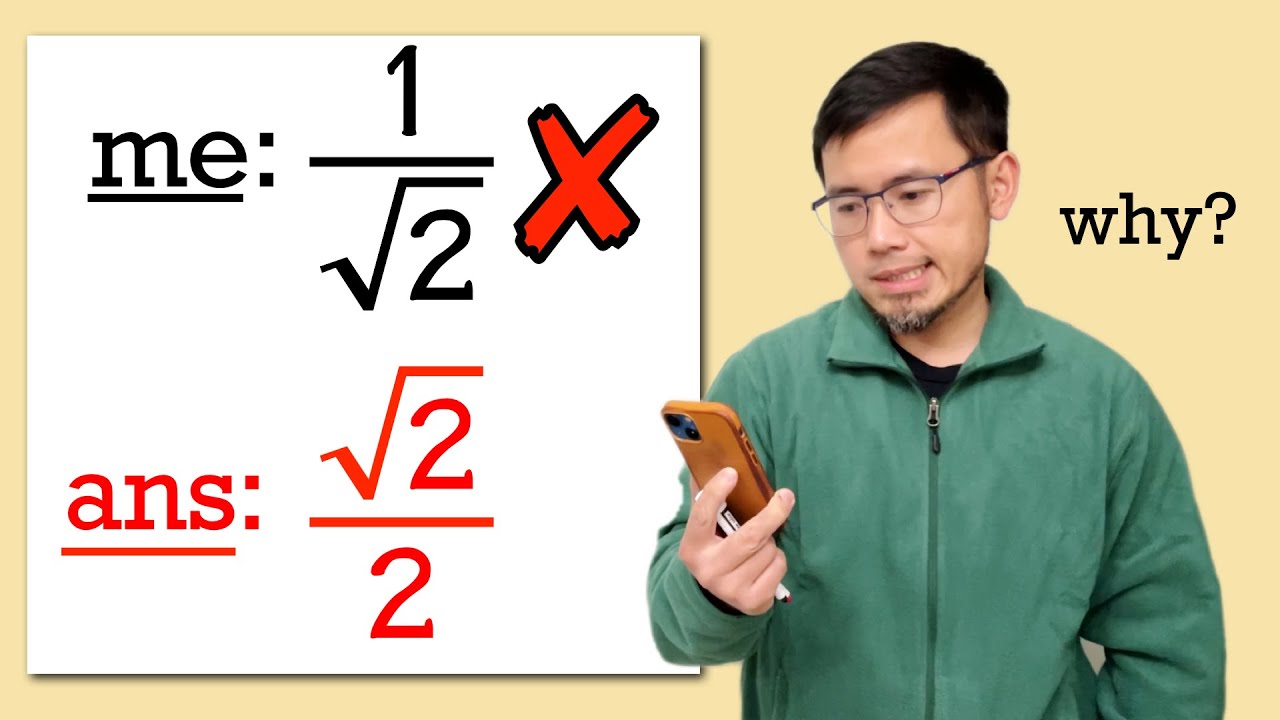
Algebra teachers always want us to 'rationalize the denominator', but why?

Learn why we have to rationalize the denominator when we are in our algebra class. We will go over the example that 1/sqrt(2) should be multiplied by sqrt(2)/sqrt(2) to "rationalize the denominator" but what's the reason for us to do that?

-----------------------------
-----------------------------
#math #algebra #mathbasics

[aomacd]

“I can do it, but I don’t want to do it” is the best lesson here

[BBQsquirrel]

As a math teacher, I always emphasise that a fraction is valid whether or not the denominator is rational. Having a rational denominator, however, allows for some further manipulations e.g. splitting a fraction to identify the real and imaginary parts of a complex number.

[jannegrey]

Important thing is to know when to do it in more complex math. Sometimes it's at the end to have a "better" answer. Sometimes you do it, because it helps you solve something in the middle of calculations - though just as often you don't do it, because then calculation might be easier. Multiplying things by 1 (so (sqrt(2)/sqrt(2)) in this case) is practically almost always allowed as far as I know.

[johnwalker]

I always wondered why math teachers seemed so insistent that we "rationalize the denominator, " but this made so much sense in explaining the "why" and not just the "because I'm the teacher and I said so" that is so pervasive in education.

I wish I could like this more than once and thank you for making this video!

[malvoliosf]

You missed my favorite thing about 1/√2 : a company was designing a new product and that number, 1/√2, kept showing up in the engineering calculations, so much so they decided to name the product the 707. It was so popular that the company, Boeing, now names the whole product line that way: the 707, 727, 737, 747, 777, 787.

[stephenlesliebrown]

"When we talk about money it's easier, right?" Classic comment! Hard to imagine a pre-20th Century world without computers or calculators, but it did exist. Yet high precision was necessary for astronomy and other scientific stuff. Here's a similar idea: Give students the choice of working out one of two problems by hand. Either 456789/258637 or 456789-258637. They will probably prefer the subtraction. Then explain that the division problem can be solved by subtraction if there is a big book of base 10 logarithms in the library to use. (If asked why logarithms are still around in this century you can say they're handy for bringing an unknown exponent down to the level of the equal sign when solving an algebraic equation.) Best wishes to all 🙂

[YonatanZunger]

Honestly, though, as a former theoretical physicist - we never did this, nor did we ever normalize "improper" fractions. For anything other than extracting decimal digits, it's far cleaner to leave them as-is. And if you need decimal digits, you can either do all of this, or learn to do fast approximate division in your head when all you need is a ballpark answer.

[JeffreyLByrd]

I rationalize denominators when applicable, but I must say, in this day in age where we carry powerful computers in our pockets, I don’t find “It’s hard to divide it by hand” to be a compelling argument for continuing the practice. When I was teaching and tutoring, my policy was always that the correct answer was correct regardless of form, but that starts to be a problem when you’re talking about integrals whose solutions can take wildly different forms based on how you handled the integration.

[goes_by_santi]

I like this explanation better than what my algebra teach gave us way back in the day, which basically amounted to "irrational denominators are wrong". Missed opportunity to educate, or perhaps she actually didn't know herself. The real lesson here is that it's not enough to just know the rules, but it's also important to know why because then we can understand when and where the rules might not apply. Good stuff, and thank you.

[gary-williams]

I always thought of the rules "rationalize the denominator" and "normalize improper fractions" as ways of "canonicalizing" a value. It's easier to grade assignments when students' answers are required to be in a particular form, for example, as the grader won't have to evaluate as many different expressions to check for equivalency.

[JHamron]

"square root of 2 is the most famous irrational number"
> pi has entered the chat

[TheJakeSweede]

Thinking of it as sqrt(2)/2 also helped me with getting better at trigonometry and the unit circle, as the sine of many of the common angles 90, 60, 45, 30 (or radian equivalent pi/2, pi/3 etc) can be remembered as +sqrt(4)/2, +sqrt(3)/2, +sqrt(2)/2, +sqrt(1)/2, but then easily simplified

[__christopher__]

In quantum information, I often used exactly that value (as well as other inverse square roots), and I never rationalized the denominator because what I really cared about was that it cancelled another square root of 2 when calculating the norm of a vector (in other words, I wanted the vector to be normalized). That fact would have been obscured by rationalizing the denominator.

The bottom line is: There is rarely a universally best representation, only one that is best for a certain purpose.

[PhillipRhodes]

Ya know, I totally get the idea here, and can appreciate that in an algebra class or whatever it makes sense to require students to rationalize. But what annoys me is when some presenters treat it as though an answer like (1 / sqrt(2) ) is "wrong". As in, like, there's actually something mathematically incorrect about it. But there's not. It's just more awkward to work with... if you're doing the long division by hand.

But approximately nobody (except teachers and poor, suffering, math students) does long division by hand. In the real world, anybody working with something that results in such an expression is eventually going to need the decimal approximation, and they're going to use a computer to work it out.

[lanzji]

Fun fact: the teachers I learned that stuff from preferred 1/sqrt(2) over sqrt(2)/2 as sort of being more reduced ... impossible to reduce even more ... some justfication like this. I'm too old to remember exactly.

[Aristothink]

Thank you. I never knew why to rationalize the denominator. I know it's ugly to leave the denominator with a root and gets in the way if we want to add or subtract another fraction. But I have never thought about your explanation. Very good. Thank you for the marvelous video, as always very simple and informative. 👍👍👍👍👍

[Wltrwllyngaeiou]

Write it as 2^-0.5

Don’t have to rationalize the denominator if there is no denominator

[realdealsd]

You can use the difference of squares when your denominator is something in the form of a + √b. Multiply the numerator and denominator by a - √b because (a+√b)(a-√b) = a²-b.

[zerohz]

in pure mathematics it shouldn’t matter but in applied mathematics it is very convenient to rationalise

[birneytitus]

One historical reason for rationalizing the denominator may be that hand calculations are easier that way. For example, it's easier to divide 2 into sqrt(2) than to divide sqrt(2) into one.