filmov
tv
Eigenstates of ANY 1D Potential in PYTHON
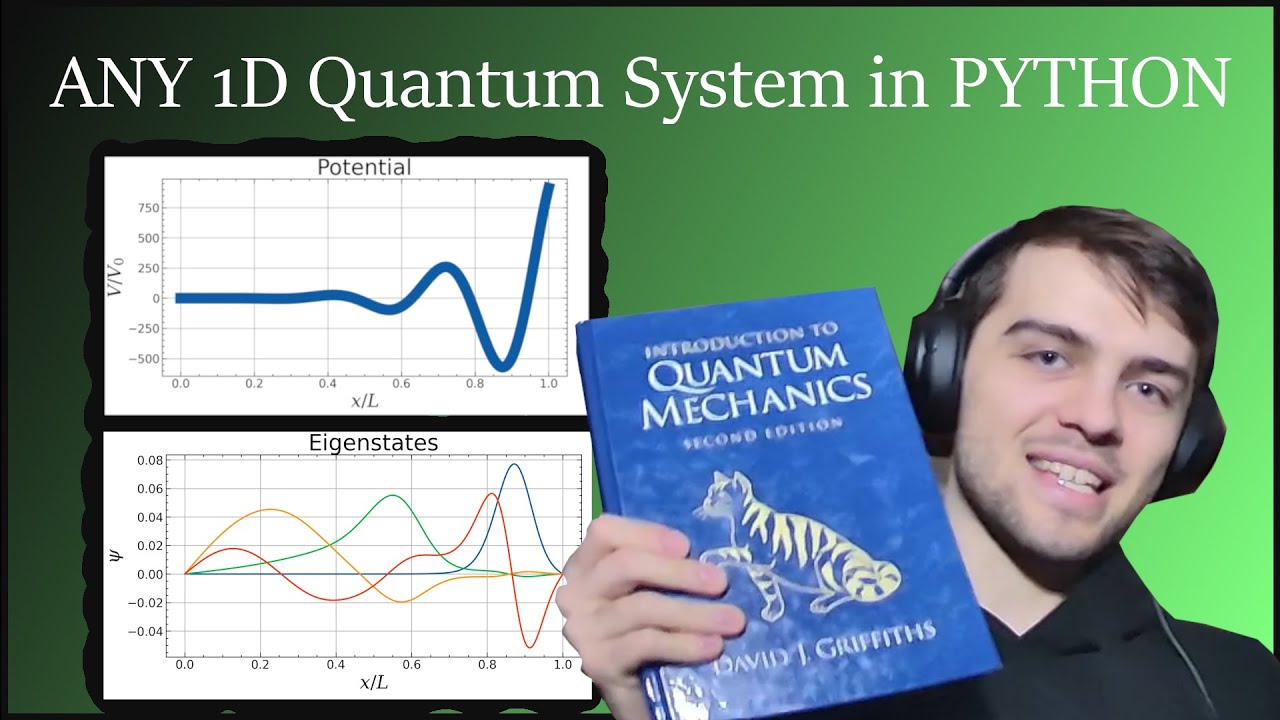
Показать описание
Remember having to solve problems analytically? What a pain. With python you can solve for any potential you want.
Code located in the link below. Go to "Python Metaphysics tutorials" and then "Vid 3"
Code located in the link below. Go to "Python Metaphysics tutorials" and then "Vid 3"
Eigenstates of ANY 1D Potential in PYTHON
Infinite square well energy eigenstates
Obatining the Eigenstates for ANY Potential (1D) // Exact Diagonalization Method
particle in a box (quantum mechanics)
Particle in a Box Part 1: Solving the Schrödinger Equation
Example Potentials in 1D
1D Quantum Gaussian Well | Numerical Solution to Schödinger Equation
Quantum Mechanics: Quantum physics in 1D Potentials | MITx on edX
1D Square Well with Step Potential. Finite Difference Solution
Deriving the Bloch's theorem
Particle in a box chopped eigenstate evolution (1D)
Solving the Energy Eigenvalue for a Free Particle
The Quantum Barrier Potential Part 1: Quantum Tunneling
Derivation - A Particle in a One Dimensional Box
Quantum Particle in 1D Potential Box
Particle in one Dimension box Potential well quantum mechanics Schrodinger wave equation application
Quantum Finite Potential 1D Eigenstates
Wave function for a 1D infinite square well
Particle in an Infinite Potential Well (QUANTUM MECHANICS)
Finite square well energy eigenstates
The Quantum Harmonic Oscillator Part 2: Solving the Schrödinger Equation
Introduction to Quantum Mechanics: Infinite square-well potential in 1D. A walkthrough
Visualizing the 3D Schrödinger Equation: Quantum Eigenstates of a Particle Confined in 3D Wells
One Dimensional (1D) Infinite Deep Potential Well [Energy and Momentum EigenValue Equation] -Part-3
Комментарии
 0:19:41
0:19:41
 0:13:13
0:13:13
 0:01:33
0:01:33
 0:14:47
0:14:47
 0:16:35
0:16:35
 0:05:05
0:05:05
 0:04:10
0:04:10
 0:01:04
0:01:04
 0:28:13
0:28:13
 0:11:43
0:11:43
 0:01:07
0:01:07
 0:08:29
0:08:29
 0:21:35
0:21:35
 0:11:03
0:11:03
 0:44:07
0:44:07
 0:29:47
0:29:47
 0:01:00
0:01:00
 0:33:51
0:33:51
 0:43:40
0:43:40
 0:10:39
0:10:39
 0:23:59
0:23:59
 0:17:31
0:17:31
 0:06:36
0:06:36
 0:42:30
0:42:30