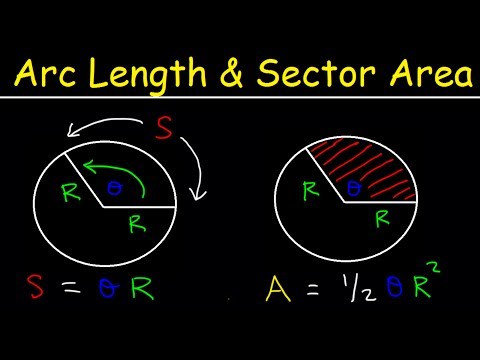
area and arc length

Check out my site & social media

Thank you for supporting! You're awesome and I know it!
blackpenredpen | 曹老師

[paulangus]

I love the dramatic sound whenever hyperbolic functions are mentioned. Was never able to memorise what they're all about.

[MrQwefty]

If you take the negative root of the equation you just get cosh(-x+c) which is the same!
It's great because this is exactly the use of the properties of the hyperbolic functions :D

[JivanPal]

The constant function y = 1 is a trivial solution; You can see this from graphical inspection, or by realising that the equations shown in the video ( that is, y = √( 1+ (dy/dx)^2 ) and the equivalent equation dy/dx = √(y^2 - 1) ) have a valid solution when dy/dx = 0; namely, y = 1.

[AndDiracisHisProphet]

of course. you can always moce the function up or down to achieve that

[JimmyXOR]

Cosh and Sinh are just sums of exponential functions, cosh(x)=(e^x+e^-x)/2 and sinh(x)=(e^x-e^-x)/2.
He could easily write the answer of the last integral as (e^5-e^-5-e^2+e^-2)/2 instead of 70.576.
Also, he divided away the trivial solution where y is a constant (y=1).

[quahntasy]

Dirac Delta function has Area= 1. But Arc length nearly Equal to 0.

This is an interesting video. Loved it.

[mokoufujiwara]

Doing it negative:
y = cosh(-x+C)
and cosh(-x) = cosh(x)
We can simply write y=cosh(x+C)

[mokoufujiwara]

y=1 seems works, take a range from x=a to x=b, area under y=1 = line segment length = b-a
But, be careful, line segment is different from arc.
But, should line segment be a subset of arc? This is a good question to discuss.

[asadyamin]

I'll just put down y, and you'll see y (why) lol 1:20

[williamadams]

Next Question : Find a function g(x) such that the perimeter of the region bounded by the x-axis, x = a, x = b and g(x) equals the area bounded by the perimeter.

[omgopet]

You can do it without solving a DE "the normal way". You want a function f(x) such that, when some other function g(x) is applied to it's derivative, returns the original function, i.e. g(f'(x))=f(x). If there is a solution, it's going to be an exponential function. As others have mentioned 1=exp(0) is the most obvious solution. Then if you examine what g(x) actually does, it looks awfully like a distance function, or something out of a Pythagorean theorem. I actually paused the video and tried doing it in my head, but I misremembered the formula for arc length and guessed y=sin(x) or y=cos(x). That didn't make sense because the arc length is strictly increasing and trig function integrals go to zero after each period... The point is, if it was 1-f'(x)^2, the solution would be obvious to anyone right away. Since you have a plus, you want a function that changes it to a minus, so you need to add an imaginary unit somewhere in that function. You want a real function, so something like i*sin(x) is out of the question, but you can put an imaginary unit in the function and the derivative will bring it out, but that's the same as using hyperbolic trig functions. From there, it's easy to find y=cos(i*x)=cosh(x), because sinh(x) is negative for x<0, and arc length is not negative.

Not saying you did anything wrong here, but if you don't recognize the derivative of arccosh(x) in the last step right away, the problem becomes a pain in the ass to integrate. On the other hand, I found that having good intuitions about exponential functions (and by extension trigonometric functions) is crucial for my students, otherwise they focus too much on how to solve DEs instead of trying to understand what these equations and solutions actually mean (talking about physics here, I guess it's different for math students).

[BigDBrian]

Of course you can. Take a reasonable arc length(if it's too steep it might not work.), e.g. the shape in the video. Now slide it up and down. It won't change the arc length, but it will change the area. So just slide it down or up until the values are equal.

[John-qmmw]

You can get

Y=1/sqrt{1-e^{2x+c}}


if you use trigonometrical substitution instead of the hypervololic cosine
.
Please do not ignore me :)

[islandcave]

To clear up a bit, the d from the c and d at the end should not be confused with the d in dx and dy. The d from the dx and dy is actually the lower case delta.

[djgulston]

Judging from the music, this is a great video to watching on Halloween! 😂

[jacoboribilik]

I am in doubt about this part 2:03 . If two definite integrals are the same, does that necessarily imply both integrands are the same too? I mean, it could be a solution but why can't there be other solutions to that equation? Someone mentioned below another solution could be 1. How do we know there are not more function that satisfy the equality? My knowledge in differential equations is little.

[duggydo]

Very nice video. Only problem is lighting glare on whiteboard in some spots.

[khbye]

At what point in calculus will you need/find it convenient to know and remember hyperbolic trig functions?

[chandankar]

Thank you so much sir...
Please upload these type of concept clearing topics...❤❤❤from india

[MA-qpzx]

That background music....just wow....You are a mathegangster sir....awesome 😉👨‍🏫