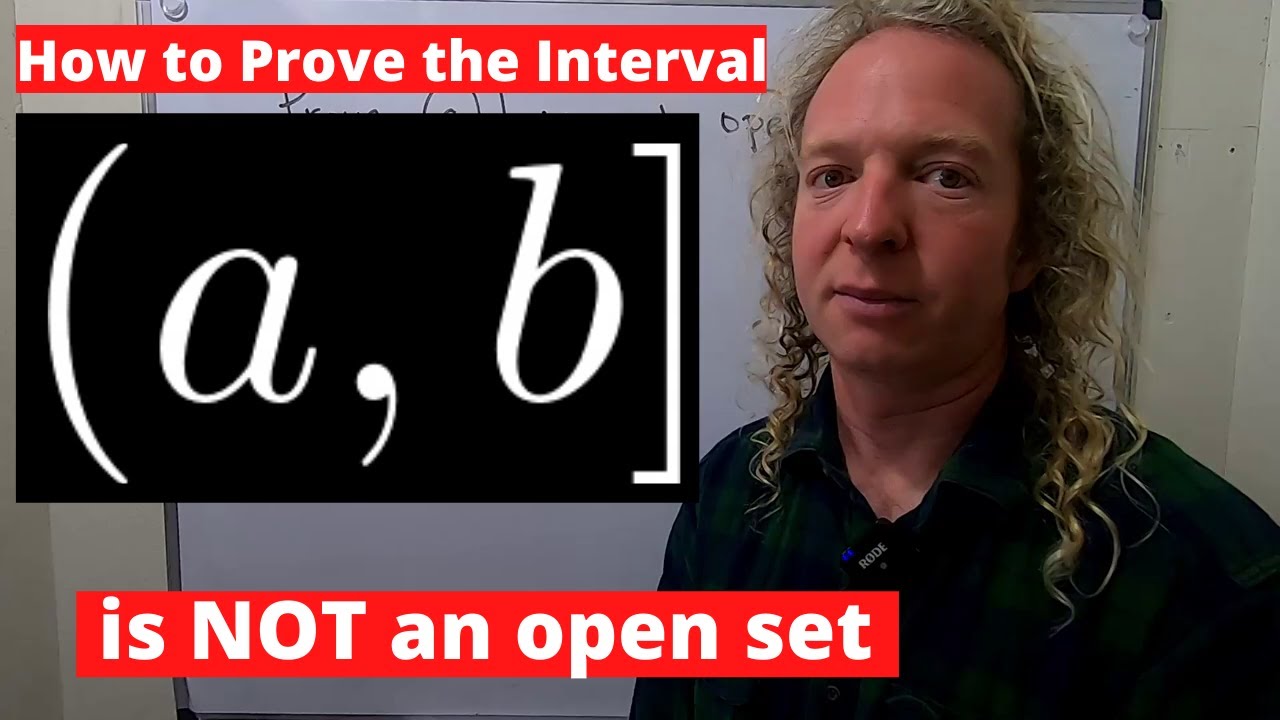
How to Prove that the Interval (a, b] is Not an Open Set

Показать описание

In this video I will show you how to prove that the interval (a, b] is not an open set. I do a proof by contradiction and I go over all of the steps very carefully. I hope this video helps someone.

If you enjoyed this video please consider liking, sharing, and subscribing.

You can also help support my channel by becoming a member

Thank you:)

Рекомендации по теме

Комментарии

Was just literally studying about this exact definition for multivariable analysis. Amazing how you always upload stuff I'm working on

liftsu

Real analysis 1 brought me here and man am I so grateful for your videos. This stuff CAN be simple so long as its explained well! Thanks for doin what you do I am almost certain you have saved many a mathematics student's grade!

nullbrain

Perfect timing, my upcoming test has this topic

BrettWoodPiano

I like the way you make calculus seem easy

thierrry.k

Thank you, I have a midterm tm and this has helped so much. Thank YOU!

MiguelMoreno-qcip

thanks for this vid. i always have hard time proving these kind of obv. stuff. would love more analysis content.

turokg

Learned a new symbol ( c with line below it ) thx

__.__-_.

Who said mathematicians can't be cool😁 Thanks for the video

kunalbarua

does not open imply closed or do you have to prove closedness separately?

pawpatrol

I am trying to figure out why it is necessary to go to the trouble of bringing ε/2 into the argument rather than just using ε. The only difference appears to be the assertion that b+ε/2 is an element of (b-ε, b+ε)⊆ (a, b] and therefore b+ε/2 is an element of (a, b] and therefore b+ε/2 is <= b. The implication that I can not reconcile is that somehow (b-ε, b+ε) ⊆ (a, b] does not imply b+ε is an element of (a, b], for if it did it would not be necessary to use ε/2, as we could simply use ε to directly contradict in similar manner to ε/2. So what is the key realization that necessitates use of ε/2 ? My mind remains troubled by thinking it valid that (b-ε, b+ε) ⊆ (a, b] implies b+ε is an element of (a, b] and therefore b+ε is <= b setting up a more direct contradiction.

dvashunz

Is there a video like this, showing that the set is neither closed?

MrCentrax

YES! topology and ... we get to stay home because of corona. What better to do than study math.

maxpercer

I sense a little bit of snark in the proof of contradiction LOL XD

yiuminghuynh

How to prove that set of rational numbers is not closed??

masoomparwej

How to Prove that the Interval (a, b] is Not an Open Set

How to Prove Trigonometric Identities (and how not to)

How To Prove The Quran Has Been Preserved Accurately

How to Prove a Function is Injective(one-to-one) Using the Definition

How to Prove Math Theorems | 1st Ex: Even + Odd = Odd

GCSE Maths - How to Prove Algebraic Identities - Proof Part 2 #63

How to prove that the limit does not exist (KristaKingMath)

How to Prove That a Quadrilateral Is a Parallelogram With Diagonals : Parallelograms & Math

How to Prove that the Interval (a, b] is Not an Open Set

How to Prove that a Matrix is Positive Definite

Learn how to use mathematical induction to prove a formula

How To Prove That Jesus Is God To A Muslim

How to Prove that the Natural Logarithm is an Onto Function

how to prove that log_2(3) is irrational by using contradiction

Symmetric Difference of Sets||How to prove that: A∆B=(AUB)–(A∩B)

How to Prove that the Derivative of a Constant is Zero(0) - Calculus

Proof 1+1=11 || Prove that 1+1=11 || How to prove 1+1=11 || Funny math proof

Learn How To Prove A Trigonometry Question & Apply Trig Identities Effectively

How to Prove that the Limit of (2n + 1)/(3n + 7) as n approaches infinity is 2/3

Prove that the Harmonic Series Diverges

Connected Classrooms: How to Prove That 1 = 2 with Mathematician James Tanton

Only U.S. President to prove a theorem

How to prove that the empty set is a subset of any set

Prove that 2=3 || How To Proof 2=3

How to Prove that a Quadratic Function is Always Positive (or Negative)