filmov
tv
A Very Interesting Exponential Equation | iˣ = -1
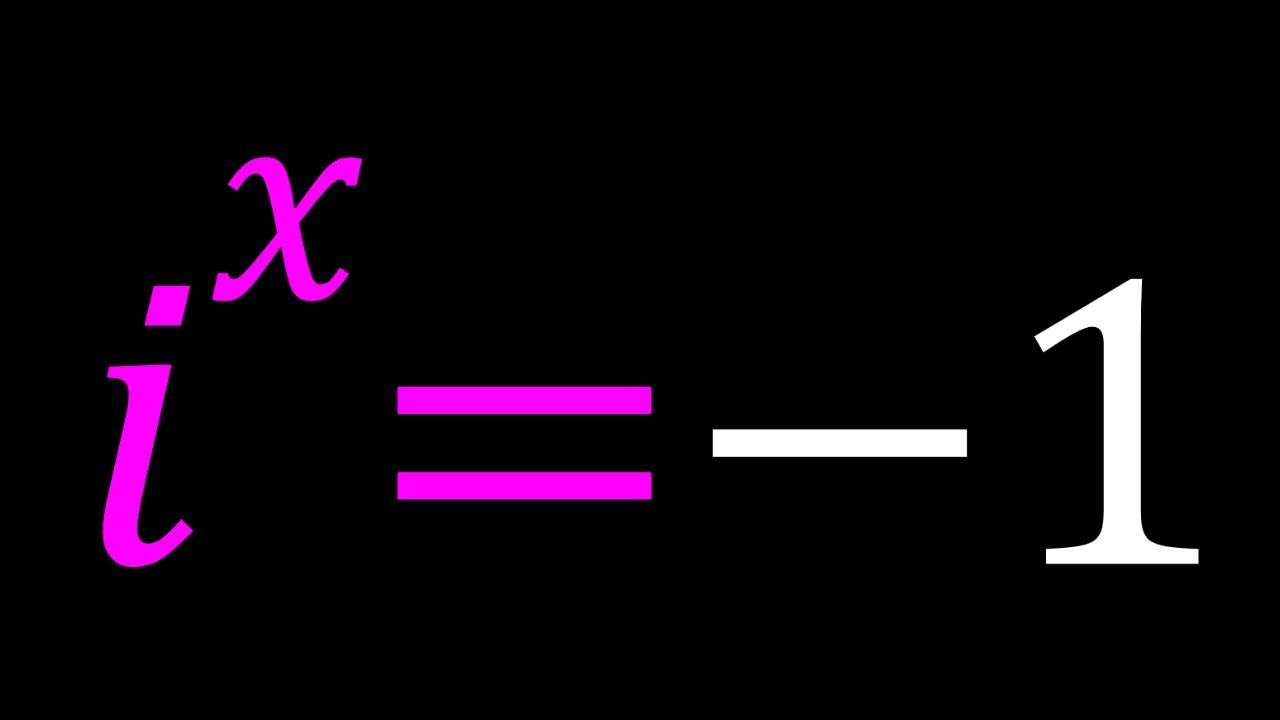
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
i^x=-1 iˣ = -1
#ChallengingMathProblems #ExponentialEquations #ComplexNumbers
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
i^x=-1 iˣ = -1
#ChallengingMathProblems #ExponentialEquations #ComplexNumbers
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
An Interesting Exponential Equation
A Very Interesting Exponential Equation | 1ˣ = -1
A Very Interesting Exponential Equation | iˣ = -1
A Very Interesting Exponential Equation | 1ˣ = -1
Interesting exponential equation. #mathshorts #exponential
Solving an Interesting Exponential Equation
Solving A Homemade Exponential Equation
An Interesting Exponential Equation
USA Nice Olympiad Exponential Equation: solve for a!
An Interesting Exponential Equation
An Interesting Exponential Equation with x^x^x
An Interesting Exponential Equation Challenge | Math Olympiad Prep!
An Interesting Exponential Equation | eˣ = -1
An Interesting Exponential Equation
An Interesting Exponential Equation | Problem 230
An Interesting Exponential Equation | Olympiad Prep | Algebra
5 simple unsolvable equations
An Interesting Exponential Equation
Solving an Interesting Exponential Equation
Exponential Equation #maths #mathstricks #shorts
Solving Exponential Equations
An Interesting Exponential Equation From Russia
A Cool Exponential Equation | Math Olympiads
How to solve an exponential equation with unknowns on both sides
Комментарии
 0:08:40
0:08:40
 0:08:58
0:08:58
 0:11:14
0:11:14
 0:01:00
0:01:00
 0:00:28
0:00:28
 0:08:28
0:08:28
 0:08:46
0:08:46
 0:08:44
0:08:44
 0:08:05
0:08:05
 0:08:17
0:08:17
 0:06:14
0:06:14
 0:08:57
0:08:57
 0:00:55
0:00:55
 0:04:46
0:04:46
 0:09:18
0:09:18
 0:12:26
0:12:26
 0:00:50
0:00:50
 0:13:20
0:13:20
 0:08:45
0:08:45
 0:00:57
0:00:57
 0:16:36
0:16:36
 0:04:52
0:04:52
 0:08:18
0:08:18
 0:04:00
0:04:00