filmov
tv
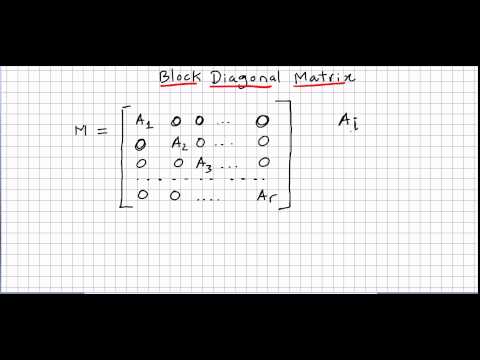
Block Diagonal Matrices

Показать описание
Every operator on a finite-dimensional complex vector space has a matrix (with respect to some basis of the vector space) that is a block diagonal matrix, with each block itself an upper-triangular matrix that contains only one eigenvalue on the diagonal.
Block Diagonal Matrices
Linear Algebra 101, Block Diagonal Matrix
Linear Algebra 17h: Easy Eigenvalues - The Block Diagonal Structure
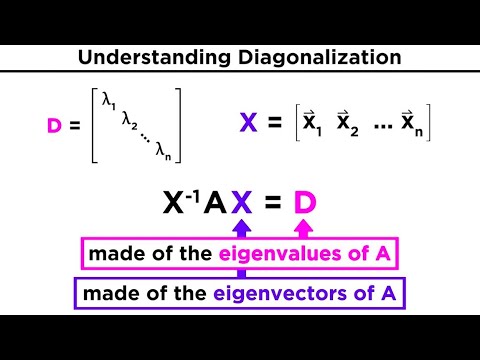
Diagonalization
BLOCK DIAGONAL MATRIX in Hindi ||Best Explanation|| block matrix|| smith normal form ||
Everything about Block Diagonal Matrix
Diagonalizing a Matrix
Minimal Polynomial of Block diagonal Matrix| Characteristic polynomial |Linear Algebra|Mathematics
LA 2.4 Partitioned Matrices
Eigen Values | Determinant, Diagonalizability Of Block Matrix | MSQ Series By GP SIR
Jordan Cannonical Form - 1 (Jordan Block, Block Diagonal Matrix, Block Matrix, JC Form with Example)
Block Diagonal Matrix #shorts #matrix #zero #square #mathematics #diagonal #square #study #maths
Block-Diagonal Matrix Representation of CNOT and Toffoli (CCX) Gates, Pauli Matrix Tensor Products
Block Diagonal matrix
4. Block matrix, triangular Block matrix and diagonal Block matrix | Linear algebra
Linear Algebra 17a: Easy Eigenvalues - Diagonal Matrix
Characteristic Polynomial Of A Matrix In 10 Seconds | Matrices 4x4 & 5x5 @gajendrapurohit
Systems of Differential Equations: Diagonalization and Jordan Canonical Form
Block Diagonal Matrices in Tensorflow
What is a block matrix
csir net june 2017 linear algebra characteristic polynomial of block diagonal matrix
Block matrices | Linear Algebra | CSIR NET
Math 240 - Chapter 3 - Determinants (Block Matrix Shortcut) - The Plug and Chug Series #7
2.2. Blocked Matrix Multiplication
Комментарии
 0:04:32
0:04:32
 0:05:53
0:05:53
 0:06:42
0:06:42
 0:08:43
0:08:43
 0:05:56
0:05:56
 0:08:04
0:08:04
 0:11:37
0:11:37
 0:06:33
0:06:33
 0:09:13
0:09:13
 0:13:59
0:13:59
 0:19:22
0:19:22
 0:00:55
0:00:55
 0:10:10
0:10:10
 0:00:16
0:00:16
 0:10:56
0:10:56
 0:05:19
0:05:19
 0:06:14
0:06:14
 0:34:25
0:34:25
 0:01:21
0:01:21
 0:02:13
0:02:13
 0:03:19
0:03:19
 0:09:46
0:09:46
 0:08:09
0:08:09
 0:03:53
0:03:53