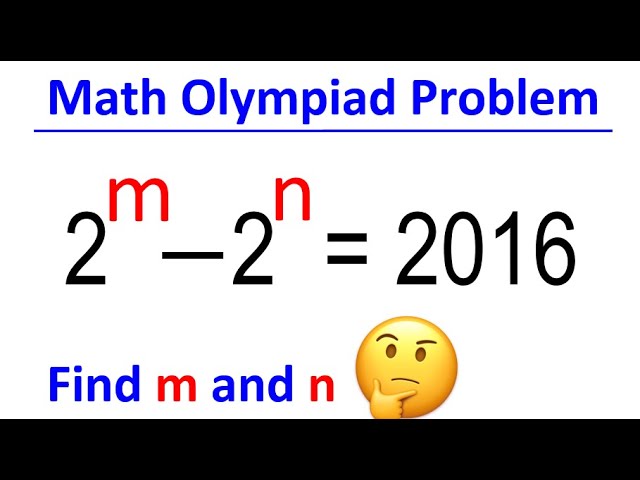
Math Olympiad Problem | Challenging Algebra Problem |How to solve System of Equation | 2^m-2^n=2016

In this video, I will be teaching how to solve this equation in math Olympiad problem. I will be solving this algebraic problem step-by-step so that you won't miss any step. The problem is find m and n from 2^m-2^n=2016

A video course for students taking the GCSE Maths exam

Disclaimar:

Affiliate Links:
The writing Tablet I use for my videos
Veikk A15 Pro
Buy Now👇👇👇

Wacom Writing Tablet

BOOKS
Math Olympiad

Other Videos
Check this problem too: X^x=X

3^x +9^x =27^x
Video link

X^(x^2-5x+6)=1
Video link

Playlist For Math Olympiad Problems

If you are new to my channel, please subscribe, like, comment and share.

[ratilaljarsania]

There is even easier method of trial and error method to solve this. As 2^m is greater than 2016. So nearest and greater than 2016 as well as it is some power of 2. Thus it is 11. So 2^11 is 2048. Then 2^n must be 2048 - 2016 = 32. Thus 2^5=32. So by trial and error m=11 & n=5. So simple. Ratilal Jarsania.

[unnwn]

i did it in a slightly different way but it was intuitive,
2^m-2^n = 2016
2^m - 2^n = 2^5 * 63
2^m - 2^n = 2^5 * (2^6 - 2^0)
2^m - 2^n = 2^11 - 2^5

thus,
m = 11
n = 5

[Nikioko]

The closest 2ⁿ > 2016 is 2¹¹ = 2048.
2048 - 2016 = 32 = 2⁵.
And voila: m = 11, n = 5. Solved in 3 seconds.

[italixgaming]

My solution (of course way faster):
2^m-2^n>0 => m>n.
The minimum value of 2^m-2^n where n<m is 2^(m-1) (for n=m-1) and the maximum value is 2^m-1 (for m=0) then 2016 is in the range [2^(m-1);2^m[.
Since 1024=2^10<2016<2^11=2048 m must be equal to 11.
2^n=2048-2016=32 then n=5.

[bingh]

2^m= 2016+2^n

Binary of 2016 =

You’ll need to add 1 at 5th position (from right) to reset all 1s and then to set a 1 before all newly reset positions. Since you add 1 at position 5, n=5. Since you reset all 6 bits before getting a new 1, m=6+5=11

[garvgupta]

The inspection method helps solve these questions splendidly and quickly. Here we notice that 2016= 2048-32 or 2^11-2^5
Therefore m=11 and n=5.

The above proof clearly explains the reasoning and method to solve this question but such questions are ought to be done by The Inspection Method to save time.

[ministryoftruth]

I really wanted to know how to solve this algebraically, so rather than using the trial and error method, I watched until the m = n + k substitution method was introduced, then the path to the solution became so crystal clear. Understanding is a dynamic quality; thus, my understanding of algebraic manipulation has incrementally advanced. Thank you, sir for the insight.

[blableu]

i did this by guesswork:

since 2016 is equal to 2048 - 32, and both are results of exponents of 2, i need to find the log of 2 of 2048 and 32:

log_2(2048) = 11
log_2(32) = 5

so,
in the equation:
2^m - 2^n = 2016,
m = 11 and n = 5

[danielflynn]

Can do this using binary representation. 2^m - 2^n is represented as (m-n) 1's followed by n 0's. 2016 is meaning n is 5 and m-n is 6 therefore m is 11.

[Dickinsonhk]

My first impression would have been to write 2016 = 2048 - 32 = 2^11- 2^5, so m=11 and n=5.

[austin]

This is an easy one to guess and check: the smallest power of 2 that is larger than 2016 is 2048. Then check to make sure that 2048 - 2016 is also a power of 2. Of course in general this method is bad for producing solutions because you don’t know how many cases you have to check before you arrive at a solution, but it’s good for this problem because literally the first case works.

[theeternalswrd]

I didn't bother with another variable, I just factored 2^n * (2^(m-n) - 1). Figured out n = 5 from factoring 2016, then added 32 to 2016 to get to 2048, then solved for m by counting the number of times multiplying 2 until reaching 2048. I work with multiples of 2 a significant amount from computer programming, so I found that easier to do in my head than the substitution.

[marsvandeplaneet]

As programmer/electronic engineer, instantly I thought; 2048 is just above 2016. 2048-2016=32. And DONE!

[ganeshdas]

2^m - 2^n = 2016 = 2^5( 2^6 -1)
or, 2^m - 2^n = 2^11 - 2^5
Now on comparing, taking m greater than n, m= 11 & n = 5

[laurentthais]

m=11 and n=5 obvious solution if you know powers of 2. No other possible solutions if m and n are integers given that the second term 2^n is growing slower than the first one (n<m).

[rajatpandey]

Solution is nicely presented. Now letus try differently. 2'm -2'n =2016. 2016 mod 9 =0. Now power of 2 mod 9 has a pattern. 2 to power 0, 1, mod 9 shall give us 1, 2, 4, 8, 7, 5, 1, 2, 4, 8, 7, 5 and so on. so m and n should be such that their mod value on LHS comes to 0. Example. It could be 2048-32. 2048 mod 9 is 5 and 32 mod 9 is 5. Mod LHS =MOD RHS =00.

[murdock]

Amazing challenge, excellent way to solve it, many thanks.
Here another approach, a bit faster: 2^11 = 2048 →
2048 - 2016 = 32 = 2^5 → m = 11 → n = 5 🙂

[davidwhite]

This is why we memorize powers tables. I knew off the top of my head that 2048 was 2 raised to a certain power, and then quickly saw the difference between that and 2016 was 32, another 2-power.

[oskaraltamiranov]

Step1)
If you factorize 2^n then you will get:
2^n (2^(m-n) -1)= 2016

Step2)
If you take 2016 and break it down into factors you will found:

2016= 2^5 x 63 = 2^5 x (64-1)=2^5 x(2^6-1)

Step3)
Establishes equality between both sides:

2^n (2^(m-n) -1) = 2^5 ( 2^6 - 1)
From here:
n=5 and m-n=6, so its mean that m=11. Nice

[Z-add]

This is the first time im seeing comparison of even and odd on both sides of the equation.