Solutions of Divergence and Curl Numerical Problems

In this video I have discussed about the solution of divergence and curl numerical problems. In the first case the divergence, we apply the divergence and curl operators on the vector field functions. A field is known as a scalar and vector according to the physical quantity that develop the field. A scalar quantity develops the scalar field and vector quantity develop the vector field.
So, in case of divergence, we operate divergence operator on the vector field function and as a result we find only the magnitude at a particular point. Divergence is use to measure the magnitude of a vector field at a particular point. When the divergence of any field is zero it means that field is solenoidal. SUGGESTED BOOK:
1. Introduction to Electrodynamics by David J. Griffiths (Author)
2. Classical Electrodynamics by John David Jackson (Author}
3.Classical Electrodynamics by S. P. Puri (Author)
4. Introduction to Electrodynamics Paperback – 2011 by Griffiths David J. (Author}
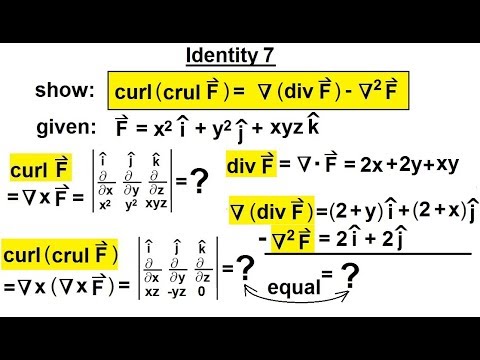
To solve the divergence put the value of del operator and vector field function and find the result of scalar product by doing simply differentiation with respect to x, y and z.Result of divergence is always a scalar quantity, we measure the magnitude only through it.

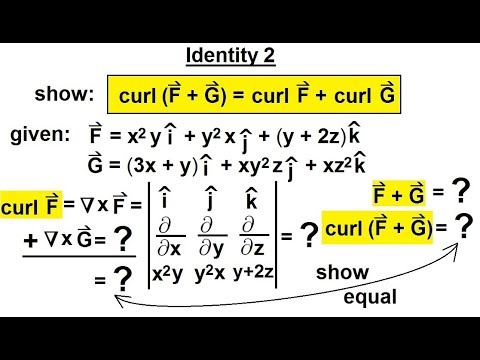
The curl of a vector field function is solved by expanding the determinant with respect to unit vectors i, j and k. A field is known irrotational if there is no rotation. So no rate of rotation means curl is zero.

[vyomhansjournals]

SUGGESTED BOOK:
1. Introduction to Electrodynamics by David J. Griffiths (Author)
2. Classical Electrodynamics by John David Jackson (Author}
3.Classical Electrodynamics by S. P. Puri (Author)
4. Introduction to Electrodynamics Paperback – 2011 by Griffiths David J. (Author}