Differential Geometry: Lecture 28: applicationa of Gauss Bonnet

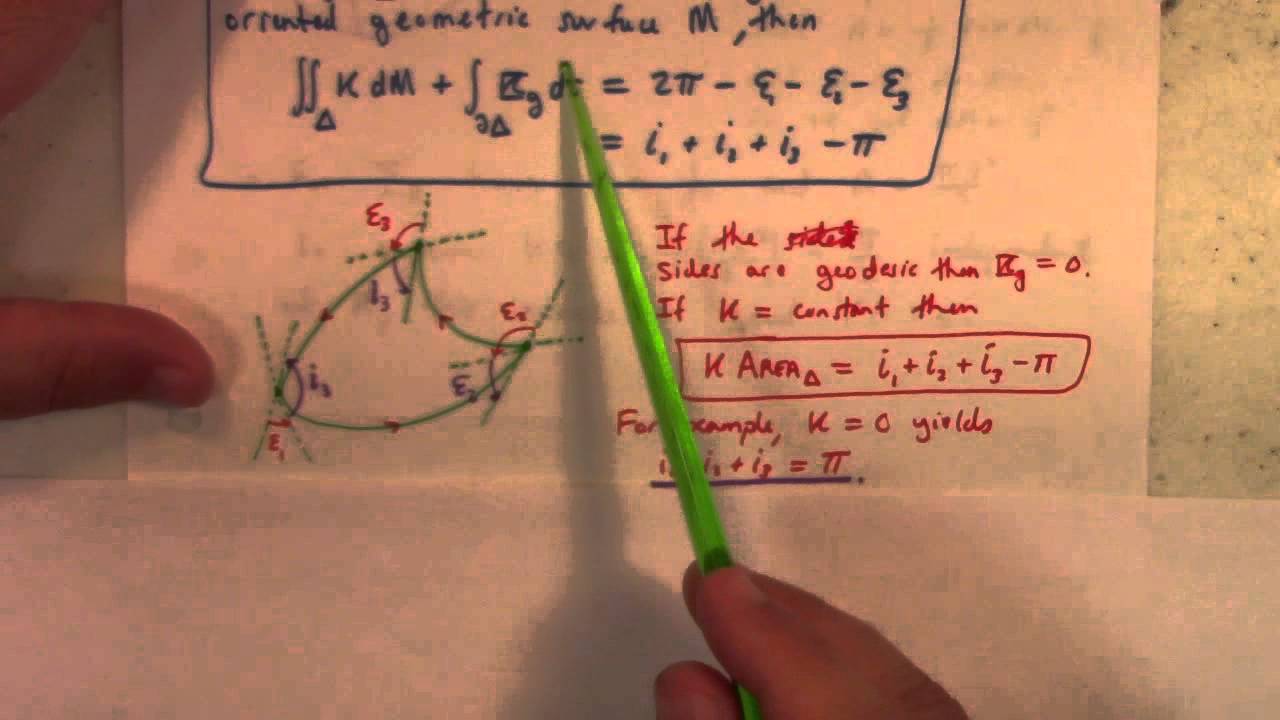
here we connect topology and geometry in a few standard examples. The vanishing Euler characteristic of the Torus implies zero total Gaussian curvature. Also, we see why non-vanishing tangent fields can exist for a torus, but cannot for a sphere. Finally, the angle defect theorems for geodesic triangles in spaces of constant curvature are discussed. Next we move to Kuhnel's text to discuss I, II and III fundamental forms and other calculational schemes absent from O'neill's text.