filmov
tv
How To Solve This Crazy Equation. Ramanujan's Radical Brain Teaser
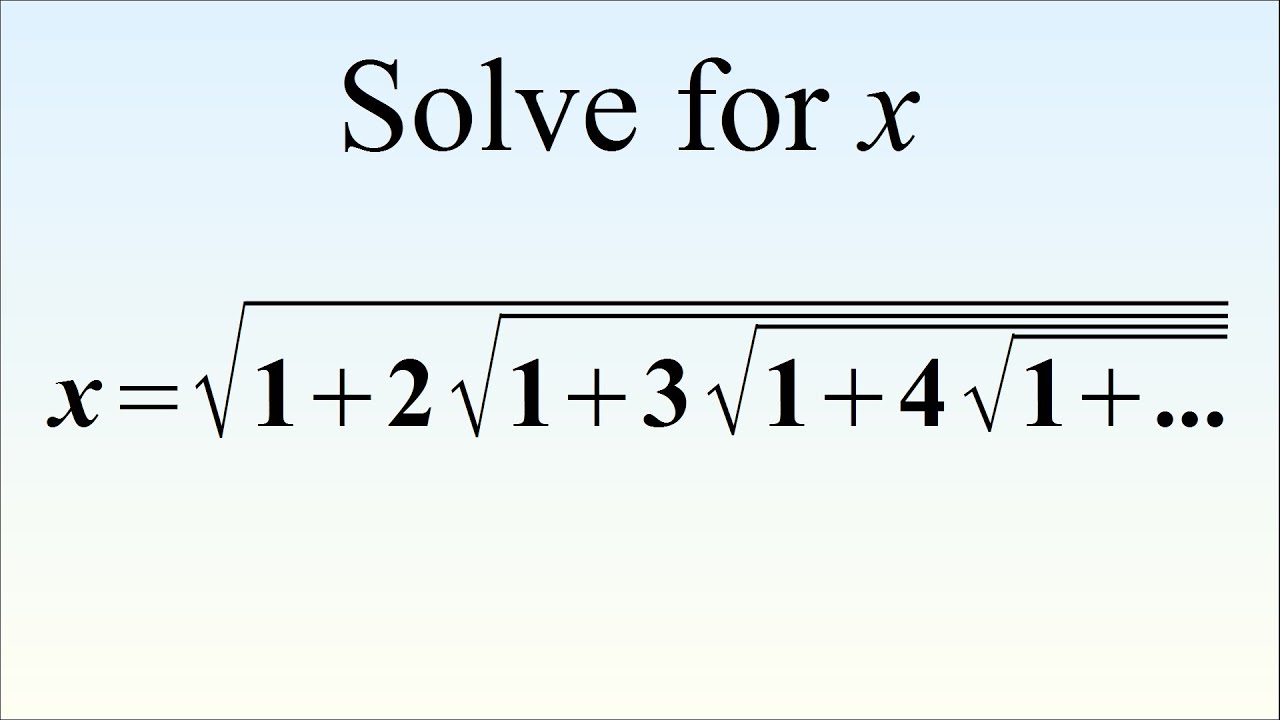
Показать описание
In 1911, Srinivasa Ramanujan posed this problem involving an infinite nested radical (a never-ending expression that has square roots inside of square roots). Can you figure out how to simplify the equation? The video presents an intuitive solution. Technically it is necessary to prove the equation has a solution (the nested radical converges) because crazy things can happen with infinite expressions. The technical proof is included in a link below.
Blog post (text/image explanation). I also justify why the pattern continues for the first solution:
Technical proof
StackExchange discussion
Thanks to Patrons!
Kyle
Alberto Nishikawa
Brian M. Mooney
If you like my videos, you can support me at Patreon:
Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.
If you buy from the links below I may receive a commission for sales. This has no effect on the price for you.
My Books
"The Joy of Game Theory" shows how you can use math to out-think your competition. (rated 3.8/5 stars on 31 reviews)
"The Irrationality Illusion: How To Make Smart Decisions And Overcome Bias" is a handbook that explains the many ways we are biased about decision-making and offers techniques to make smart decisions. (rated 4.6/5 stars on 3 reviews)
"Math Puzzles Volume 1" features classic brain teasers and riddles with complete solutions for problems in counting, geometry, probability, and game theory. Volume 1 is rated 4.4/5 stars on 13 reviews.
"Math Puzzles Volume 2" is a sequel book with more great problems. (rated 4.3/5 stars on 4 reviews)
"Math Puzzles Volume 3" is the third in the series. (rated 3.8/5 stars on 5 reviews)
"40 Paradoxes in Logic, Probability, and Game Theory" contains thought-provoking and counter-intuitive results. (rated 4.3/5 stars on 12 reviews)
"The Best Mental Math Tricks" teaches how you can look like a math genius by solving problems in your head (rated 4.7/5 stars on 4 reviews)
"Multiply Numbers By Drawing Lines" This book is a reference guide for my video that has over 1 million views on a geometric method to multiply numbers. (rated 5/5 stars on 3 reviews)
Blog post (text/image explanation). I also justify why the pattern continues for the first solution:
Technical proof
StackExchange discussion
Thanks to Patrons!
Kyle
Alberto Nishikawa
Brian M. Mooney
If you like my videos, you can support me at Patreon:
Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.
If you buy from the links below I may receive a commission for sales. This has no effect on the price for you.
My Books
"The Joy of Game Theory" shows how you can use math to out-think your competition. (rated 3.8/5 stars on 31 reviews)
"The Irrationality Illusion: How To Make Smart Decisions And Overcome Bias" is a handbook that explains the many ways we are biased about decision-making and offers techniques to make smart decisions. (rated 4.6/5 stars on 3 reviews)
"Math Puzzles Volume 1" features classic brain teasers and riddles with complete solutions for problems in counting, geometry, probability, and game theory. Volume 1 is rated 4.4/5 stars on 13 reviews.
"Math Puzzles Volume 2" is a sequel book with more great problems. (rated 4.3/5 stars on 4 reviews)
"Math Puzzles Volume 3" is the third in the series. (rated 3.8/5 stars on 5 reviews)
"40 Paradoxes in Logic, Probability, and Game Theory" contains thought-provoking and counter-intuitive results. (rated 4.3/5 stars on 12 reviews)
"The Best Mental Math Tricks" teaches how you can look like a math genius by solving problems in your head (rated 4.7/5 stars on 4 reviews)
"Multiply Numbers By Drawing Lines" This book is a reference guide for my video that has over 1 million views on a geometric method to multiply numbers. (rated 5/5 stars on 3 reviews)
Комментарии
 0:17:43
0:17:43
 0:15:55
0:15:55
 0:00:22
0:00:22
 0:00:32
0:00:32
 0:00:41
0:00:41
 0:00:35
0:00:35
 0:02:57
0:02:57
 0:14:24
0:14:24
 0:00:09
0:00:09
 0:25:43
0:25:43
 0:00:14
0:00:14
 0:29:59
0:29:59
 0:00:21
0:00:21
 0:00:41
0:00:41
 0:03:02
0:03:02
 0:00:49
0:00:49
 0:00:59
0:00:59
 0:00:27
0:00:27
 0:00:17
0:00:17
 0:00:50
0:00:50
 0:16:51
0:16:51
 0:17:17
0:17:17
 0:22:21
0:22:21
 0:00:22
0:00:22