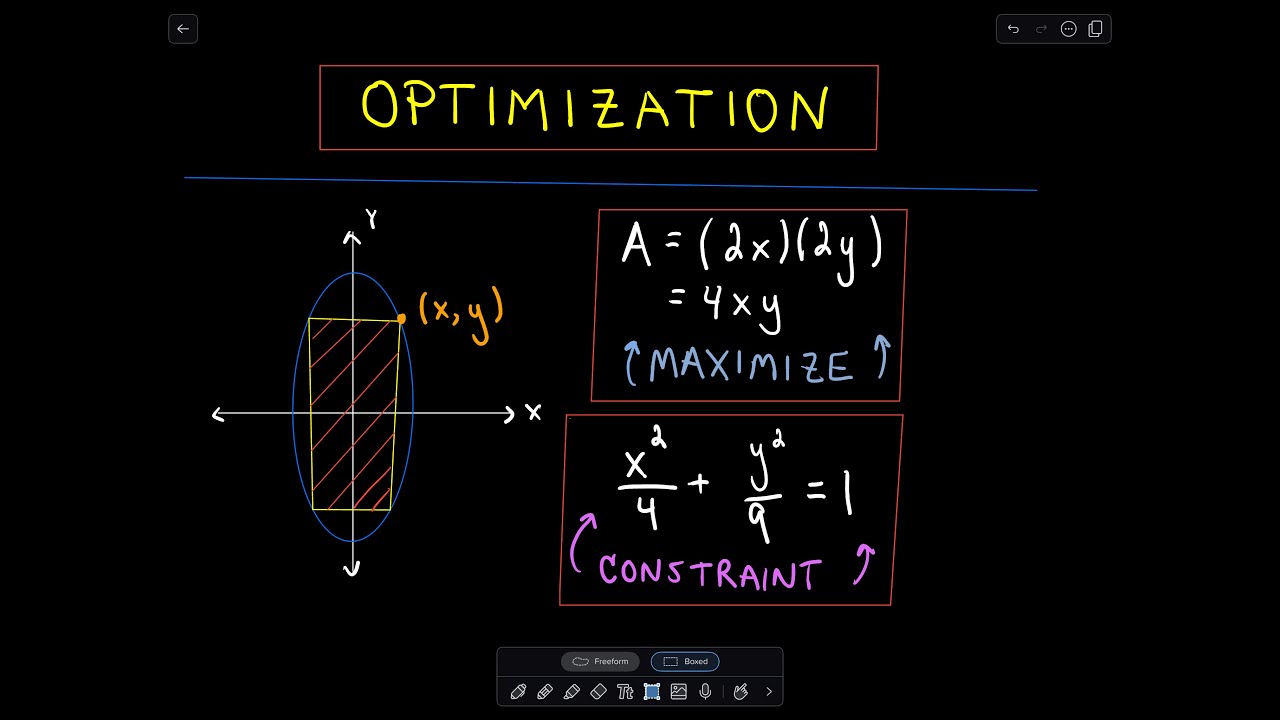
Optimization Problem: Largest Rectangle Inscribed in an Ellipse

Optimization Problem: Largest Rectangle Inscribed in an Ellipse

📐 Maximize Your Geometry Skills! 📐
In this video, we tackle a calculus optimization question: finding the largest rectangle that can be inscribed within the ellipse defined by the equation (x^2) / 4 + (y^2) / 9 = 1

What You’ll Learn:

Understanding the Ellipse: Get a clear overview of the ellipse and how it relates to the rectangle we want to inscribe.
Setting Up the Optimization Problem: Learn how to formulate the area of the rectangle in terms of the ellipse’s dimensions.
Applying Calculus Techniques: Follow along as I derive the area function, find critical points, and determine the dimensions of the rectangle that maximize the area.

Why Watch This Video?

Ideal for Students: Perfect for high school and college students looking to strengthen their understanding of optimization in calculus.
Clear Explanations: Enjoy step-by-step guidance that makes complex concepts easy to grasp.
Real-World Applications: Discover how these optimization principles can be applied in various fields, including engineering and design.

📈 Engage with the Content:

LIKE this video if it helps enhance your understanding of optimization!
SHARE with friends or classmates eager to learn more about calculus!
SUBSCRIBE for more insightful math tutorials, problem-solving strategies, and educational resources!

#Optimization #Ellipse #Calculus #MaximizingArea #Mathematics #MathTutorial #EducationalContent #LearningCalculus #ProblemSolving #HighSchoolMath #CollegeCalculus #RealWorldApplications #Geometry

[sumairsunny]

From equation of an ellipse: a=2, b =3:
by observation the area of the rectangle inscribed inside the ellipse is 2*a*cos(theta) * 2*b*sin(theta).Which is 4*a*b*sin(theta)*cos(theta). since 4*a*b are constants of the ellipse we don't really need to care about them (as the ellipse changes as long as the area of the rectangle has a*b in its formula, the rectangle will also change accordingly). What will determine the biggest (or largest area) rectangle will be the biggest possible value of cos(theta)*sin(theta). Even if you're bad at math, just plug in values for theta and see what the biggest value of (sin(theta*cos(theta)) comes to. you will notice the largest value is 0.5 @ theta = 45 degrees... so largest AREA of rectangle inscribed in an ellipse = 4*a*b*0.5 = 2ab.

[yanarisutandey]

Hell nah man. My professor gave a problem like this for an exam tonight except it was an ellipsoid and we had to maximize a rectangular box with in it's constraints. Talk about PISSED. Hard ass exam.

[cctang]

I think it is easier to consider the square of the area . After substituting y in terms of x in the equation of square of area, we can take the derivative of square of A with respect to x and set it to zero, the critical values of x can still be obtained . One of the advantages of doing this is that the differentiation is more simple. The other is that there is no need to discuss the numerator of the derivative as in your method.

[animeshbhargava]

Please make a video of cauchy-riemann equation
Your videos are really helpful

[GlaceGFX]

I think you can just set the area function equal to another function but it is squared to remove the square root in order to make differentiation easier.

[Crissybooable]

hate the sharpie sound, love the learning

[TAYLOR-]

Dude, I have a quiz on optimization tomorrow. Perfect timing lol

[gofio]

Amazing work you just made my studentlife much easier ! 👍

[kindaamazing]

you have saved the life of a Pakistani student!thnx a lot

[annapabreu]

You have already helped a brazilian student! Many thanks

[Bangalibabu]

Can't we assume the co-ordinate as (2x, 2y) or (x/2, y/2) in place of (x, y) ?

[roseb]

im confused when you took the derivative of x squared/4 if you use the quotient rule you get 1/16 and derivative of 1 is equal zero I get how you get half but which answer and method is it?

[logeshwarsaravanakumar]

Guys, take f as 4xy and g has given ellipse equation and use Lagrangian multiplier method i.e F = f + lambda g. It will be pretty ezy✌️

[K-qg]

Patrick JMT - that was wow! Your the best !

[heliocentric]

A=4xy so A'=4y+4xy'=0 implies y'=-y/x
(Where A' and y' are the derivatives with respect to [wrt] x)
Differentiate the equation of the ellipse wrt x:
(2x/4)+(2yy'/9)=0, substitute -y/x for y':
(x/2)-(2y^2/9x)=0, so 9x^2=4y^2 ...(1)
The equation of the ellipse can be simplified to:
9x^2+4y^2=36 ..(2)
Equations (1) and (2) give x=sqrt(2) and y=3/sqrt(2).
After showing that these values of x and y actually yield a maximum area: A=12

[turkeyroast]

Isn't this easier with Lagrange Multipliers?

[nathangillespie]

I believe that your original area equation is incorrect, I think it should be [ A=2x*2y OR A=2(xy)]. Correct me if I'm wrong though.

[cctang]

Correction, it should be denominator not numerator. I make a mistake.

[kiritokun]

need help with this problem, Find the dimension of rectangle of maximum Area A that can be inscribed in the portion of the parabola y*2 = 4px intercepted by the line x = email me with a detailed solution:)

[colinsilver]

God I hate fractions. I try to get rid of them as soon as possible. I needed clarity on the "constraint" function, once I get pass that, I'm OK.