filmov
tv
Number Theory 1 - Infinitely Many Prime Numbers
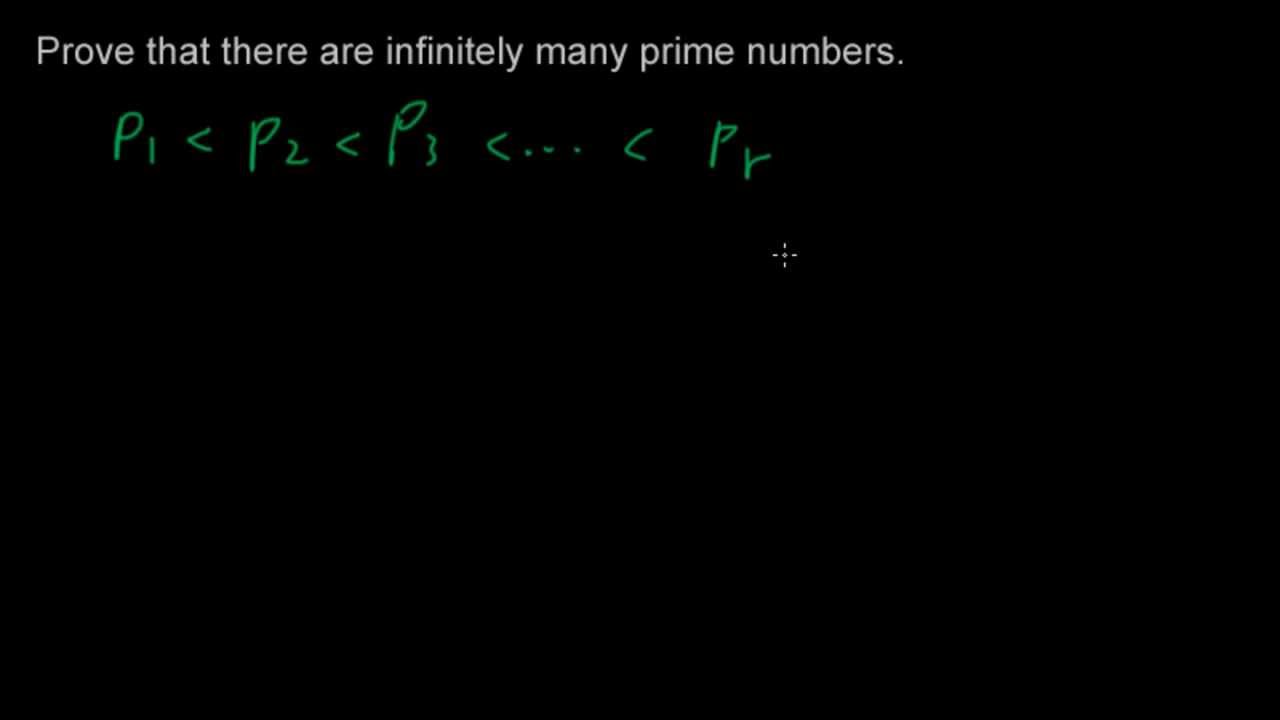
Показать описание
Prove that there are infinitely many prime numbers. This is one of the classic proofs in number theory, and in math. It was first proved by Euclid. This version is slightly different from Euclid's proof.
Number Theory 1 - Infinitely Many Prime Numbers
Number Theory | Infinitely many primes of the form 4n+1.
Number Theory | Infinitely many primes of the form 3n+1.
Mathematicians Use Numbers Differently From The Rest of Us
Number Theory | Infinitely many primes of the form 4n+3.
Infinity is bigger than you think - Numberphile
The High Schooler Who Solved a Prime Number Theorem
Elementary Number Theory: Infinitely Many Primes
Determine The Largest Radical Number? | An Algebra Puzzle
Number Theory: There are an Infinite Number of Irrational Numbers - Part 1
Quadratic Residue (Part 9) Infinite Primes of the form 4k+1 and 8k-1
1 Billion is Tiny in an Alternate Universe: Introduction to p-adic Numbers
How An Infinite Hotel Ran Out Of Room
How Goldbach and Euclid Proved There Are Infinitely Many Primes?
A proof from the Book on the infinite number of primes
Mathematician Explains Infinity in 5 Levels of Difficulty | WIRED
The Simplest Math Problem No One Can Solve - Collatz Conjecture
Proving there are INFINITE Prime Numbers in 1 Minute! #shorts
Infinite Series - Numberphile
Every Student Should See This
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
Why is 1 not #prime? #math #numbertheory
Proof: There are Infinitely Many Primes (There is no Largest Prime)
When mathematicians get bored (ep1)
Комментарии
 0:02:01
0:02:01
 0:06:41
0:06:41
 0:10:17
0:10:17
 0:33:06
0:33:06
 0:08:54
0:08:54
 0:08:00
0:08:00
 0:05:15
0:05:15
 0:28:43
0:28:43
 0:11:14
0:11:14
 0:04:07
0:04:07
 0:10:46
0:10:46
 0:21:53
0:21:53
 0:06:07
0:06:07
 0:05:02
0:05:02
 0:00:55
0:00:55
 0:24:44
0:24:44
 0:22:09
0:22:09
 0:00:59
0:00:59
 0:09:31
0:09:31
 0:00:58
0:00:58
 0:00:38
0:00:38
 0:01:00
0:01:00
 0:04:25
0:04:25
 0:00:37
0:00:37