filmov
tv
The Content of a Polynomial (Algebra 2 Lecture 9 Video 2)

Показать описание
Lecture 9: We started this lecture by proving that if R[x] is a UFD then R is a UFD. The main goal of the rest of this lecture was to prove the converse, that if R is a UFD then R[x] is a UFD. Let R be a UFD and F be its field of fractions. We proved Gauss' Lemma, which helps to explain the relationship between factorizations of a polynomial p(x) in R[x] and factorizations in F[x]. We defined the content of a polynomial in R[x] and proved that content is multiplicative. We then proved the main result of this lecture. At the end of the lecture we talked briefly about factorizations of monic polynomials in R[x] where R is an integral domain that is not necessarily a UFD.
 0:16:34
0:16:34
 0:05:13
0:05:13
 0:01:09
0:01:09
 0:12:33
0:12:33
 0:12:18
0:12:18
 0:11:09
0:11:09
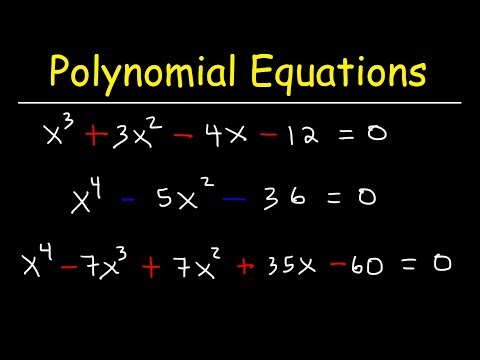 0:14:19
0:14:19
 0:03:30
0:03:30
 0:03:07
0:03:07
 0:03:38
0:03:38
 0:10:04
0:10:04
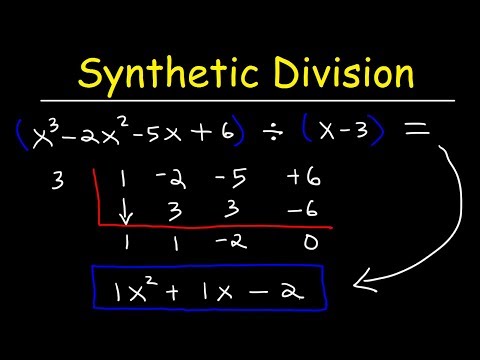 0:10:32
0:10:32
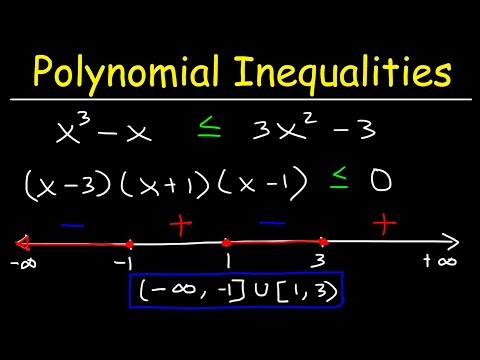 0:12:56
0:12:56
 0:05:48
0:05:48
 0:03:49
0:03:49
 0:05:15
0:05:15
 0:04:57
0:04:57
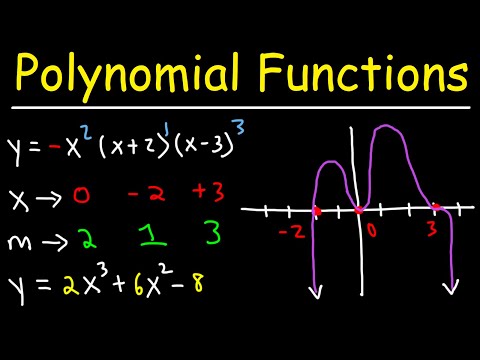 0:20:28
0:20:28
 0:03:07
0:03:07
 0:44:03
0:44:03
 0:14:10
0:14:10
 0:00:59
0:00:59
 0:00:45
0:00:45
 0:14:35
0:14:35