filmov
tv
Learn how to use the chain rule in calculus
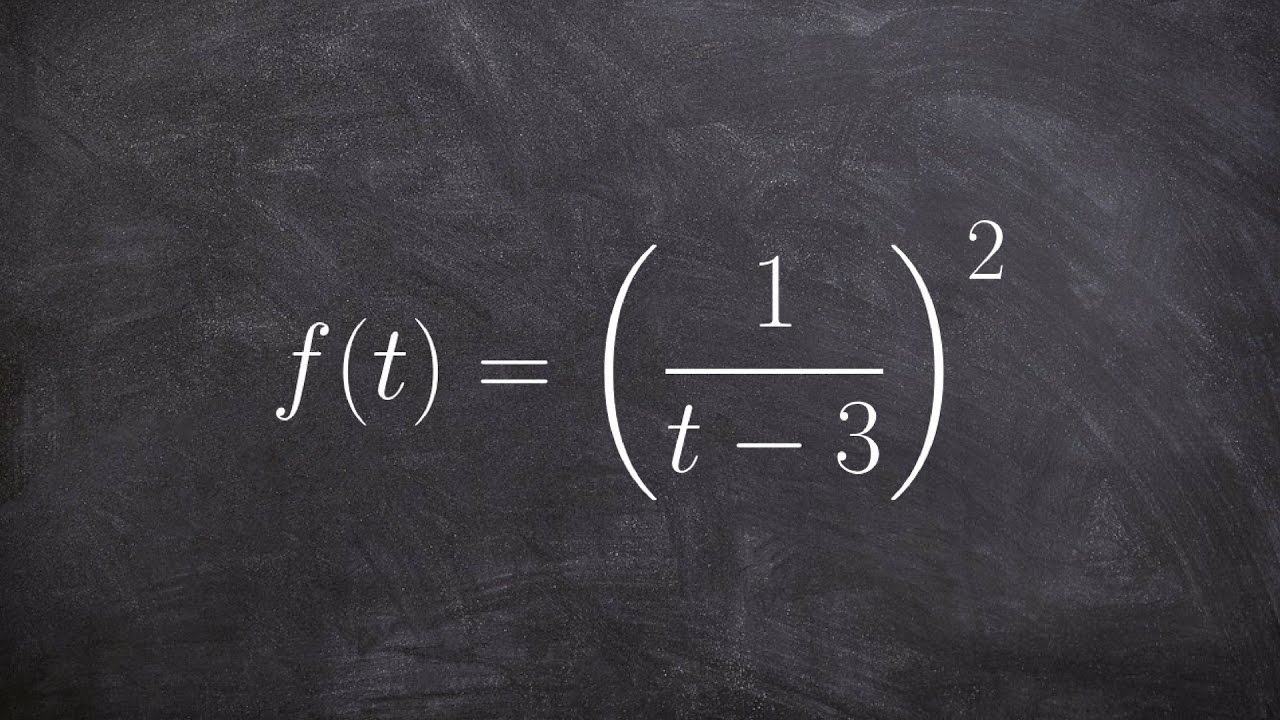
Показать описание
👉 Learn how to find the derivative of a function using the chain rule. The derivative of a function, y = f(x), is the measure of the rate of change of the function, y, with respect to the variable x. The process of finding the derivative of a function is called differentiation. There are various methods of finding the derivative of a function including, direct differentiation, product rule, quotient rule, chain rule (function of a function), etc.
When given a function of the form y = f(g(x)), then the derivative of the function is given by y' = f'(g(x))g'(x). This method of differentiation is called the chain rule. The chain rule is used to find the derivative of a function that is a function of another function.
Organized Videos:
✅The Derivative
✅Find the First and Second Derivatives of a Function
✅Find the Differentiability of a Function
✅Find the Derivative of Absolute Value Function
✅Find the Derivative of Exponential and Logarithmic Functions
✅Find the Derivative using Implicit Differentiation
✅Find the Derivative of Inverse Functions
✅Find the Point Where the Tagent Line is Horizontal
✅Write the Equation of the Tangent Line
✅Find the Derivative from a Table
✅Chain Rule Differentiation
✅Product Rule Derivatives
✅Find the Derivative of Trigonometric Functions
✅Find the Derivative using the Power Rule
✅Quotient Rule Derivatives
✅Solve Related Rates Problems
Connect with me:
#derivatives #brianmclogan
When given a function of the form y = f(g(x)), then the derivative of the function is given by y' = f'(g(x))g'(x). This method of differentiation is called the chain rule. The chain rule is used to find the derivative of a function that is a function of another function.
Organized Videos:
✅The Derivative
✅Find the First and Second Derivatives of a Function
✅Find the Differentiability of a Function
✅Find the Derivative of Absolute Value Function
✅Find the Derivative of Exponential and Logarithmic Functions
✅Find the Derivative using Implicit Differentiation
✅Find the Derivative of Inverse Functions
✅Find the Point Where the Tagent Line is Horizontal
✅Write the Equation of the Tangent Line
✅Find the Derivative from a Table
✅Chain Rule Differentiation
✅Product Rule Derivatives
✅Find the Derivative of Trigonometric Functions
✅Find the Derivative using the Power Rule
✅Quotient Rule Derivatives
✅Solve Related Rates Problems
Connect with me:
#derivatives #brianmclogan
Комментарии
 0:13:12
0:13:12
 0:08:08
0:08:08
 0:14:01
0:14:01
 0:00:44
0:00:44
 0:25:15
0:25:15
 0:04:58
0:04:58
 0:01:37
0:01:37
 0:21:25
0:21:25
 0:52:07
0:52:07
 0:39:20
0:39:20
 0:11:57
0:11:57
 0:06:58
0:06:58
 0:12:33
0:12:33
 0:03:02
0:03:02
 0:15:50
0:15:50
 0:13:26
0:13:26
 0:32:07
0:32:07
 0:01:29
0:01:29
 0:03:45
0:03:45
 0:03:17
0:03:17
 0:31:30
0:31:30
 0:02:40
0:02:40
 0:00:16
0:00:16
 0:44:04
0:44:04