filmov
tv
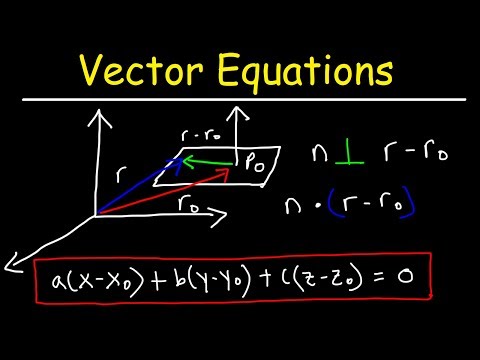
Vector and Scalar Equations of a Plane
Показать описание
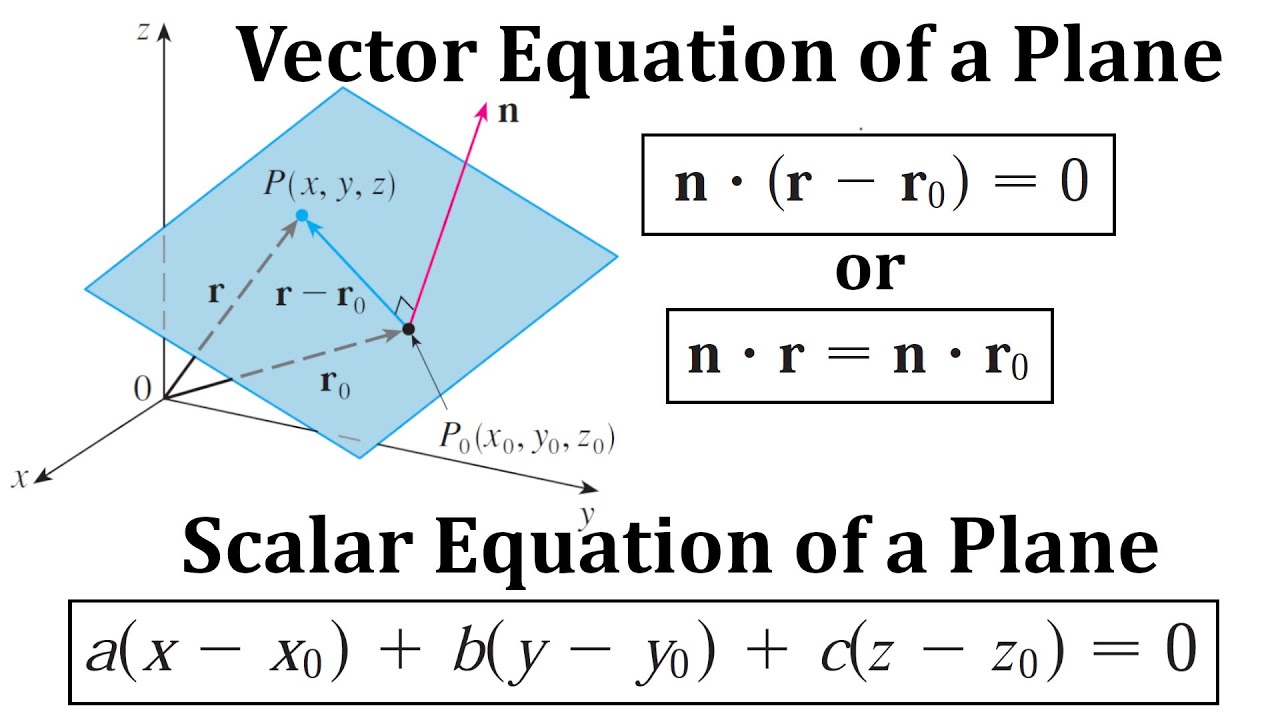
In this video I go over planes in 3D and derive the vector equation of a plane and then convert that into scalar equations of a plane. A plane can be described by a point on the plane and a vector perpendicular to the plane. This perpendicular or orthogonal vector is called a normal vector. We can obtain a formula for the plane by noting that the dot product is equal to zero for perpendicular vectors. And then we can take a vector on the plane and towards the point on the plane and compute the dot product with the normal vector to obtain the vector equation of a plane. If we expand this vector equation by writing out its components and expanding out the dot product, we obtain the scalar equation of a plane.
The timestamps of key parts of the video are listed below:
- Vector Equation of a Plane: 0:00
- Scalar Equation of a Plane: 6:02
- Example 4: 8:02
- Graphing Plane with GeoGebra 3D Graphing Calculator: 13:38
This video was taken from my earlier video listed below:
Related videos:
------------------------------------------------------
The timestamps of key parts of the video are listed below:
- Vector Equation of a Plane: 0:00
- Scalar Equation of a Plane: 6:02
- Example 4: 8:02
- Graphing Plane with GeoGebra 3D Graphing Calculator: 13:38
This video was taken from my earlier video listed below:
Related videos:
------------------------------------------------------
How To Find The Vector Equation of a Line and Symmetric & Parametric Equations
Vector and Scalar Equations of a Plane
Vector and scalar equations of a plane
Scalars and Vectors
What is a vector? - David Huynh
12 - What are Vectors and Scalars?
Vectors - Basic Introduction - Physics
Write Vector Parametric form from Scalar Equation of Line Through Origin
Everything You Need to Know About VECTORS
Vectors | Chapter 1, Essence of linear algebra
Vectors - Precalculus
Vector and Scalar Equations of a Plane
Scalar and vector fields | Lecture 11 | Vector Calculus for Engineers
How to Find the Scalar Equation of a Plane
How To Find The Equation of a Plane Given Three Points
Introduction to Vectors and Their Operations
Lecture 7.3 | Scalar vs. Vector Equations of Planes.
How to write a vector equation of a line in parametric scalar form
How To Find The Equation of a Plane Given a Point and Perpendicular Normal Vector
Calculus 3 - Intro To Vectors
Steps to Scalar Equation of Line from vector Equation Q5b
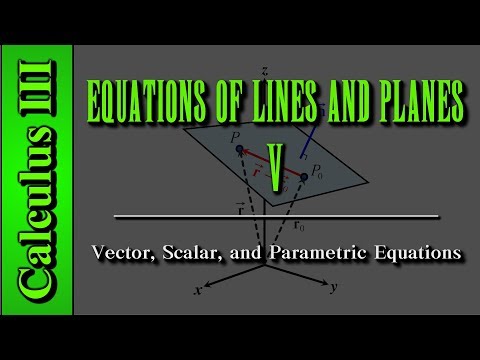
Calculus III: Equations of Lines and Planes (Level 5) | Vector, Scalar, and Parametric Equations
Vector, Parametric and Scalar Equations in 3 Space
Vector and Scalar EQuations of a Line in R2
Комментарии
 0:11:37
0:11:37
 0:14:28
0:14:28
 0:03:21
0:03:21
 0:11:21
0:11:21
 0:04:41
0:04:41
 0:19:33
0:19:33
 0:12:13
0:12:13
 0:06:21
0:06:21
 0:17:42
0:17:42
 0:09:52
0:09:52
 0:18:47
0:18:47
 0:01:56
0:01:56
 0:08:53
0:08:53
 0:03:15
0:03:15
 0:06:56
0:06:56
 0:10:17
0:10:17
 0:07:07
0:07:07
 0:01:43
0:01:43
 0:07:37
0:07:37
 0:57:54
0:57:54
 0:04:00
0:04:00
 0:12:04
0:12:04
 0:08:44
0:08:44
 0:41:33
0:41:33