filmov
tv
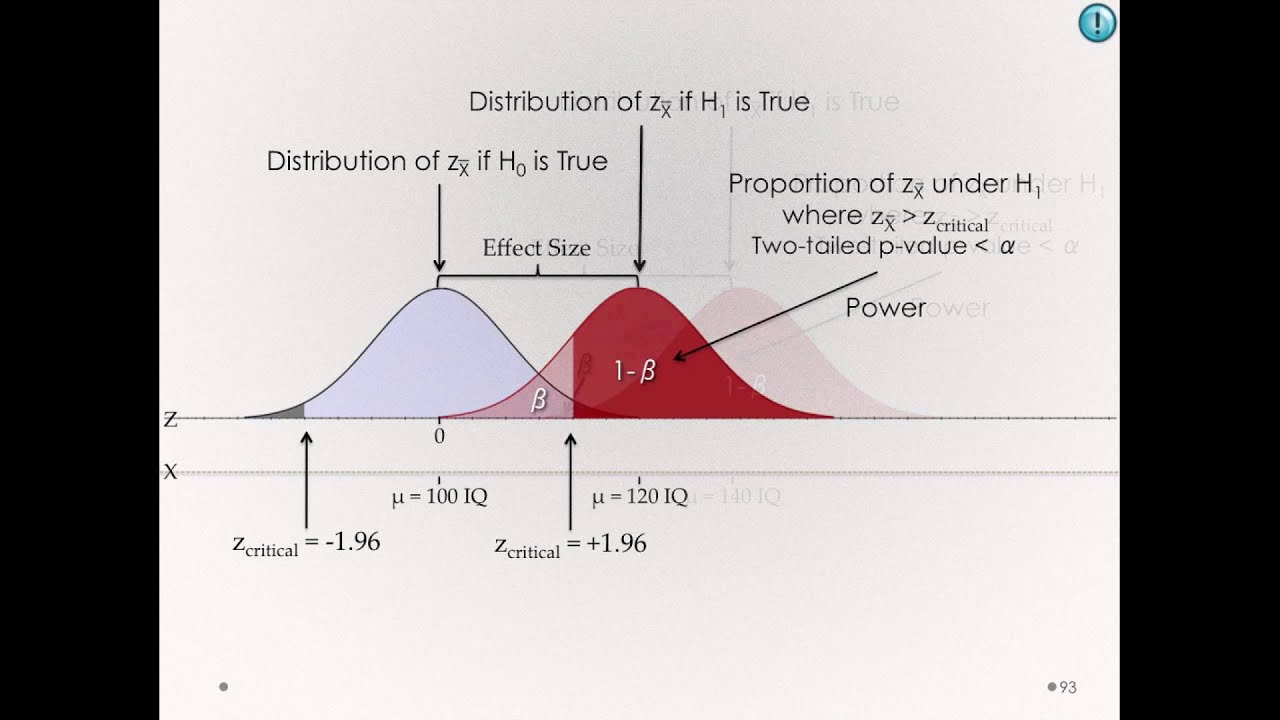
Factors Affecting Power - Effect size, Variability, Sample Size (Module 1 8 7)
Показать описание
Power Factor Explained - The basics what is power factor pf
Statistical Power, Clearly Explained!!!
Power Analysis, Clearly Explained!!!
What Factors Affect the Power of a Z Test?
Factors Affecting Power - Effect size, Variability, Sample Size (Module 1 8 7)
Introduction to power in significance tests | AP Statistics | Khan Academy
Factors Affecting Power - Alpha Level (Module 1 8 8)
Factors Affecting the Resistance | Electricity | Physics
Factors Affecting Power - Directional Hypotheses (Module 1 8 9)
FACTORS AFFECTING SOLAR POWER GENERATION, Let's Explore - Tech
Lecture#26: Corona Effect & Factors Affecting the Corona
Factors Affecting Sag
PS45 Factors Affecting Corona Loss
Factors affecting Resistance
Factors Affecting Electricity Usage
Electrical Corona Effect | Causes, Effects & Ways to minimise | TheElectricalGuy
Factors affecting Power wrap-up (Module 1 8 10)
Factors Affecting Power Effect size, Variability, Sample Size Module 1 8 7
Factors Affecting Corona Power Loss
Science 5 | Factors that Affect the Strength of Electromagnets || Joshua Alejo
GCSE Physics - Factors Affecting Gas Pressure #30
What is corona effect/ Factor affecting corona/Method for reducing corona
Power & Effect Size
Corona Loss, Factors Affecting Corona Loss - Corona - Power System 2
Комментарии
 0:11:09
0:11:09
 0:08:19
0:08:19
 0:16:45
0:16:45
 0:12:25
0:12:25
 0:08:09
0:08:09
 0:09:45
0:09:45
 0:03:14
0:03:14
 0:06:01
0:06:01
 0:11:52
0:11:52
 0:06:48
0:06:48
 0:06:58
0:06:58
 0:07:59
0:07:59
 0:16:31
0:16:31
 0:03:14
0:03:14
 0:01:05
0:01:05
 0:08:53
0:08:53
 0:03:27
0:03:27
 0:08:10
0:08:10
 0:08:32
0:08:32
 0:09:39
0:09:39
 0:03:39
0:03:39
 0:06:26
0:06:26
 0:11:04
0:11:04
 0:22:54
0:22:54